A particle is projected from the inclined plane at angle 37∘ with the inclined plane in upward direction with speed 10m/s. The angle of inclined plane with horizontal is 53∘. Then the maximum height attained by the particle from the inclined plane will be-
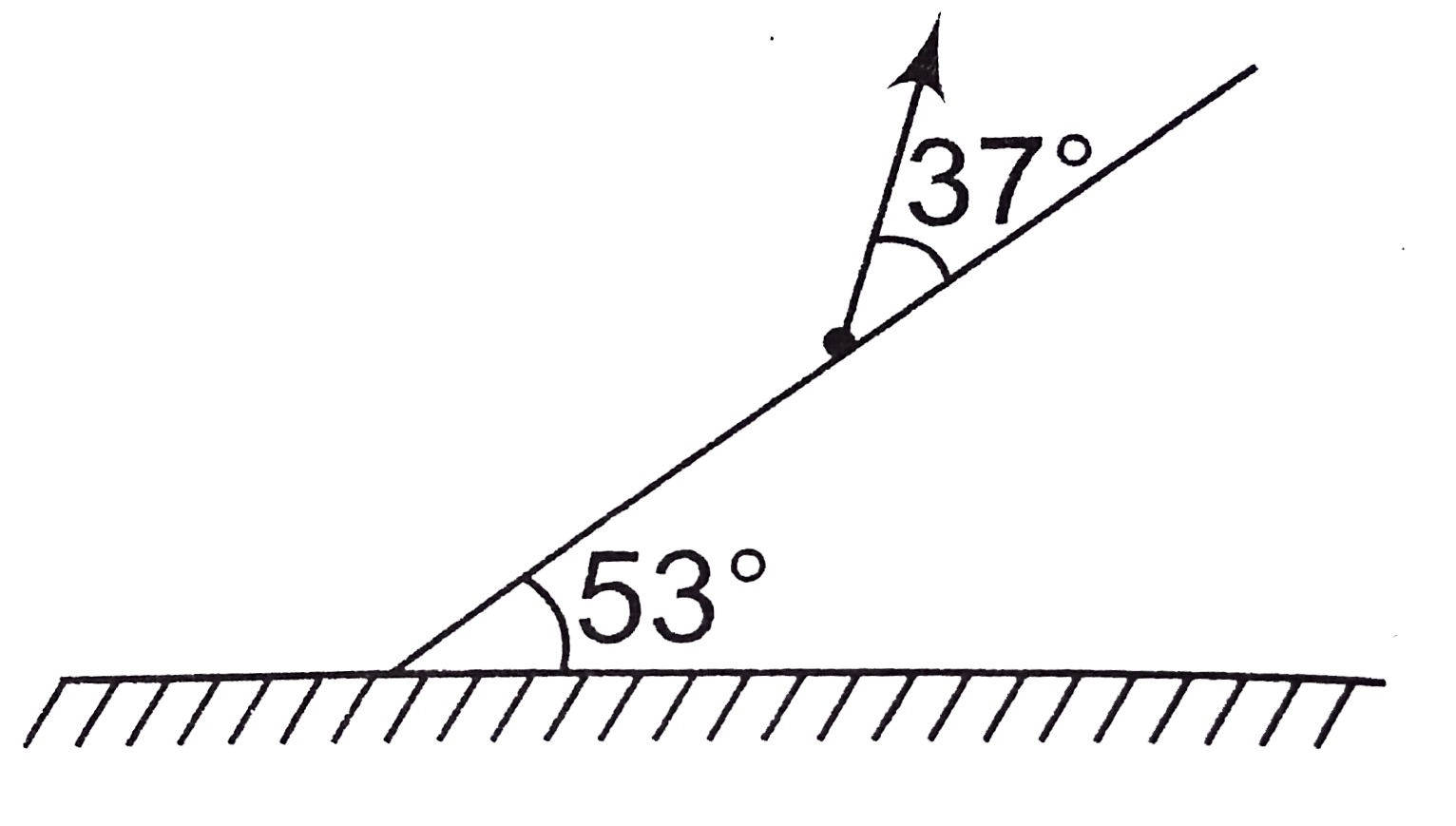
3m
4m
5m
zero
The correct Answer is:A
Maximum height from inclined plane is
H=u2⊥2a⊥=(10sin37∘)22gcos53∘=3m.
Topper's Solved these Questions
- DAILY PRACTICE PROBLEMBOOK - RESONANCECHAPTER - DAILY PRACTICE PROBLEMEXERCISE - DPP No.12
9 Videos
- DAILY PRACTICE PROBLEMBOOK - RESONANCECHAPTER - DAILY PRACTICE PROBLEMEXERCISE - DPP No.13
20 Videos
- DAILY PRACTICE PROBLEMBOOK - RESONANCECHAPTER - DAILY PRACTICE PROBLEMEXERCISE - DPP No.10
9 Videos
- CURRENT ELECTRICITYBOOK - RESONANCECHAPTER - CURRENT ELECTRICITYEXERCISE - High Level Problems (HIP)
21 Videos
- ELECTRO MAGNETIC WAVESBOOK - RESONANCECHAPTER - ELECTRO MAGNETIC WAVESEXERCISE - Exercise 3
27 Videos
Similar Questions
A bullet is fired from the bottom of the inclined plane at angle θ=37∘ with the inclined plane. The angle of incline is 30∘ with the horizontal. Find the
(a) position of the maximum height of the bullet from the inclined plane ,
(b) time of light ,
( c) range along the incline ,
(d) the value of θ at which the range will be maximum ,
( e) maximum range.
View SolutionA cannon of mass 1000 kg located at the base of an inclined of an inclined plane fires a shell of mass 100 kg in a horizontal direction with a velocity 180 km//hr.The angle of inclination of inclined plane with the horizontal is 45∘. The coefficient of friction between the canon and inclined plane is 0.5. The height in meter to which the cannon ascends the inclined plane as a result of recoil is (g=10m/s2)
View Solution
Knowledge Check
If the particle is projected down onto the inclined plane at same speed and angle with the inclined plane the ratio of the time of flight and time of flight for upward projection is :
A1:√2B√2:1C1:1Dnone of thesea particle is projected down on inclined plane with a velocity of 21m/s at an angle of 60∘ with the horizontal its range on the inclined plane inclined at an angle of 30∘ with the horizontal is
A21dmB2.1dmC30dmD6dma particle is projected down on inclined plane with a velocity of 21m/s at an angle of 60∘ with the horizontal its range on the inclined plane inclined at an angle of 30∘ with the horizontal is
A21dmB2.1dmC30dmD6dm
A particle is projected on an inclined plane with a speed u as shown in (Fig. 5.61). Find the range of the particle on the inclined plane.
 .
.
View SolutionAs an inclined plane is made slowly horizontal by reducing the value of angle θ with horizontal. The component of weight parallel to the plane of a block resting on the inclined plane-
View SolutionA ball is projected from point A with velocity 10ms−1 perpendicular to the inclined plane as shown in figure. Range of the ball on the inclined plane is

View SolutionA projectile is projected on an inclined plane as shown in figure. 'D' is a point of maximum height from inclined plane then :

View SolutionIn figure, the angle of inclination of the inclined plane is 30∘. Find the horizontal velocity V0 so that the particle hits the inclined plane perpendicularly.
 .
.
View Solution
