Water is filled to height $h$ in a fixed vertical cylinder placed on horizontal surface. At time $t = 0$ a small hole a drilled at a height $h / 4$ from bottom of cylinder as shown. The cross section area of hole is a and the cross-section area of cylinder is $A$ such that $A > > a$ .

The duration of time for which water flows out of hole is.

$\frac{A}{a} \sqrt{\frac{3 h}{2 g}}$

$\frac{a}{A} \sqrt{\frac{3 h}{2 g}}$

$\frac{A}{a} \sqrt{\frac{2 h}{3 g}}$

None of these

Video Solution

Text Solution

Verified by Experts

The correct Answer is:A

The initial velocity of water coming out of hole is horizontal and hole is at a height $\frac{h}{4}$ from ground. Hence time taken by water to reach ground is $t = \sqrt{\frac{2 (h / 4)}{g}}$ which remains constant
$∴ x = v t$ where v is velocity of effux…
Since v decreases with time x will decrease

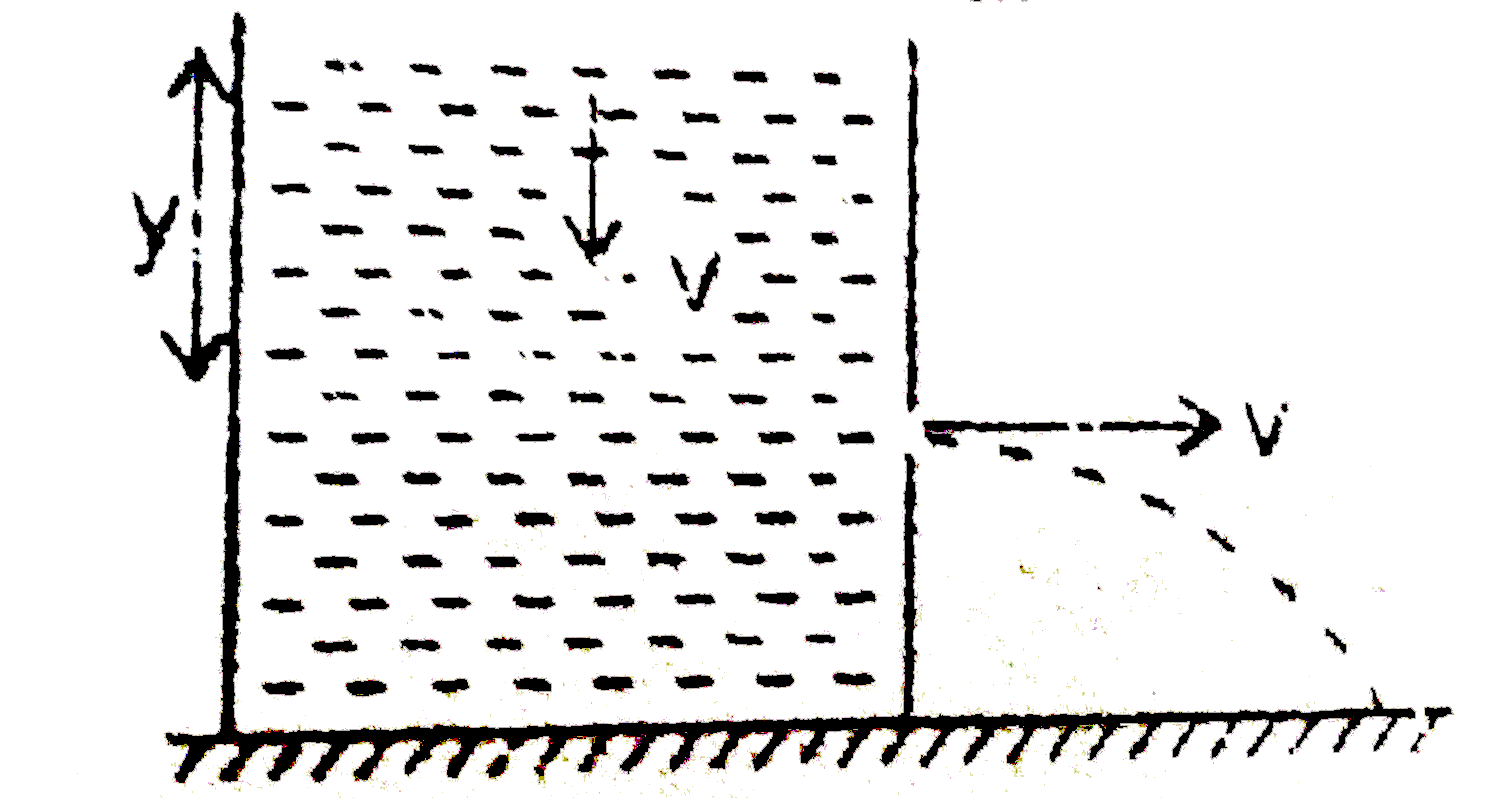
Let y be the height of water surface above hole
$∴ - \frac{d y}{d t} = \frac{a v}{A} = \frac{a \sqrt{2 g y}}{A}$
$∴ 0 \int 3 h / 4 \frac{d y}{\sqrt{2 g y}} = t \int 0 - \frac{a}{A} d t$
$∴ t = \frac{A}{a} \sqrt{\frac{3 h}{2 g}}$

Updated on:21/07/2023

Class 12 PHYSICS QUESTION BANK

Topper's Solved these Questions

Knowledge Check

Question 1 - Select One or More
Water is filled to height $h$ in a fixed vertical cylinder placed on horizontal surface. At time $t = 0$ a small hole a drilled at a height $h / 4$ from bottom of cylinder as shown. The cross section area of hole is a and the cross-section area of cylinder is $A$ such that $A > > a$ .

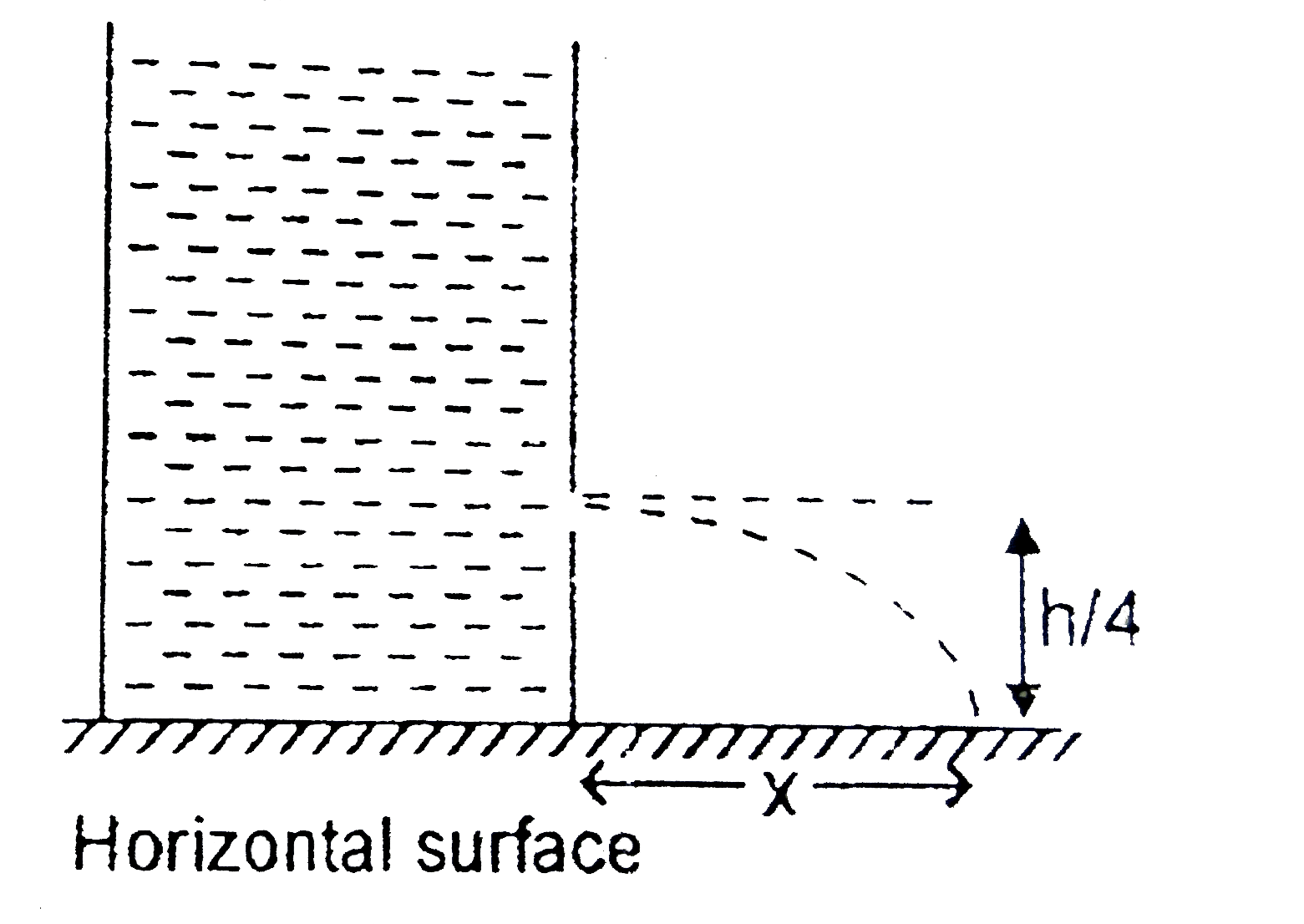
Let the value of horizontal distance of point where the4 water fall on horizontal surface from bottom of cylinder be $x$ as shown. Then from time $t = 0$ till water comes out of hole, pick the correct statement:
A $x$ increases with time.
B $x$ decreases with time.
CAs long as water comes out of hole, the time taken by a water particle starting from hole to reach the horizontal surface remians constant.
DAs long as water comes out of hole, the time taken by a water particle starting from hole to reach the horizontal surface decreases
Question 2 - Select One
A cylindrical tank is filled with water to a level of 3m. A hole is opened at a height of 52.5 cm from bottom. The ratio of the area of the hole to that of cross-sectional area of the cylinder is 0.1. The square of the speed with which water is coming out from the orifice is (Take $g = 10 m / s^{2}$ )
A $50 m^{2} / s^{2}$
B $40 m^{2} / s^{2}$
C $51.5 m^{2} / s^{2}$
D $50.5 m^{2} / s^{2}$
Question 3 - Select One
A cylindrical tank is filled with water to a level of 4 m. A hole is opened at a height of 60 cm from bottom. The ratio of the area of the hole to that at cross-sectinal area at cylinder is $0.2 .$ Then the velocity with which water is coming out is $(g = 10 m / s^{2})$
A $7.9 m / s$
B $9.2 m / s$
C $8.4 m / s$
D $8.9 m / s$