Water is filled in a container upto height 3m. A small hole of area 'a' is punched in the wall of the container at a height 52.5 cm from the bottom. The cross sectional area of the container is A. If a/A=0.1 then v2 is (where v is the velocity of water coming out of the hole)
(a) 50
(b) 51
(c) 48
(d) 51.5
The correct Answer is:A
To solve the problem, we will use the principles of fluid dynamics, specifically the Bernoulli equation and the equation of continuity.
1. Identify the Given Values:
- Height of water in the container, H1=3m
- Height of the hole from the bottom, H2=52.5cm=0.525m
- The ratio of the areas, aA=0.1
2. Calculate the Height Difference:
- The height difference h between the water surface and the hole is:
h=H1−H2=3m−0.525m=2.475m
3. Apply the Bernoulli Equation:
- According to Bernoulli's principle, for a fluid flowing in a streamline, the following equation holds:
P1+12ρv21+ρgH1=P2+12ρv22+ρgH2
- Since both points are open to the atmosphere, the pressures P1 and P2 cancel out. Thus, we have:
12ρv21+ρgH1=12ρv22+ρgH2
4. Cancel the Density:
- Dividing the entire equation by ρ gives:
12v21+gH1=12v22+gH2
5. Rearranging the Equation:
- Rearranging gives:
12v22=12v21+g(H1−H2)
- Simplifying further:
v22=v21+2g(H1−H2)
6. Using the Continuity Equation:
- From the continuity equation, we have:
Av1=av2⟹v1=aAv2
- Given aA=0.1, we can write:
v1=0.1v2
7. Substituting v1 in the Bernoulli Equation:
- Substitute v1 into the equation:
v22=(0.1v2)2+2g(H1−H2)
- Expanding gives:
v22=0.01v22+2g(2.475)
- Rearranging yields:
v22−0.01v22=2g(2.475)
- This simplifies to:
0.99v22=2g(2.475)
8. Calculating v22:
- Using g=10m/s2:
0.99v22=2×10×2.475
0.99v22=49.5
v22=49.50.99≈50m2/s2
Final Answer:
Thus, the velocity squared v2 of the water coming out of the hole is:
v2=50m2/s2
- Height of water in the container, H1=3m
- Height of the hole from the bottom, H2=52.5cm=0.525m
- The ratio of the areas, aA=0.1
- The height difference h between the water surface and the hole is:
h=H1−H2=3m−0.525m=2.475m
- According to Bernoulli's principle, for a fluid flowing in a streamline, the following equation holds:
P1+12ρv21+ρgH1=P2+12ρv22+ρgH2
- Since both points are open to the atmosphere, the pressures P1 and P2 cancel out. Thus, we have:
12ρv21+ρgH1=12ρv22+ρgH2
- Dividing the entire equation by ρ gives:
12v21+gH1=12v22+gH2
- Rearranging gives:
12v22=12v21+g(H1−H2)
- Simplifying further:
v22=v21+2g(H1−H2)
- From the continuity equation, we have:
Av1=av2⟹v1=aAv2
- Given aA=0.1, we can write:
v1=0.1v2
- Substitute v1 into the equation:
v22=(0.1v2)2+2g(H1−H2)
- Expanding gives:
v22=0.01v22+2g(2.475)
- Rearranging yields:
v22−0.01v22=2g(2.475)
- This simplifies to:
0.99v22=2g(2.475)
- Using g=10m/s2:
0.99v22=2×10×2.475
0.99v22=49.5
v22=49.50.99≈50m2/s2
Thus, the velocity squared v2 of the water coming out of the hole is:
v2=50m2/s2
Topper's Solved these Questions
- MECHANICAL PROPERTIES OF MATTER AND FLUIDSBOOK - SUNIL BATRA (41 YEARS IITJEE PHYSICS)CHAPTER - MECHANICAL PROPERTIES OF MATTER AND FLUIDSEXERCISE - Subjective Problems
1 Videos
- LAWS OF MOTIONBOOK - SUNIL BATRA (41 YEARS IITJEE PHYSICS)CHAPTER - LAWS OF MOTIONEXERCISE - JEE Main And Advanced
79 Videos
- MOMENTUM & IMPULSEBOOK - SUNIL BATRA (41 YEARS IITJEE PHYSICS)CHAPTER - MOMENTUM & IMPULSEEXERCISE - JEE Main And Advanced
30 Videos
Similar Questions
Water is filled to a height in a long container. From what height below the free surface a hole should be bored in the wall of a container so that the horizontal range is maximum?
View SolutionThe level of water in a tank is 5m high.A hole of area 1cm^2 is made in the bottom of the tank. The rate of leakage of water from the hole is (g=10ms^(-2)
View Solution
Knowledge Check
Oil is filled in a cylindrical container up to height 4m. A small hole of area 'p' is punched in the wall of the container at a height 1.52m from the bottom. The cross sectional area of the container is Q. Ifpq=0.1 then v is (where vis the velocity of oil coming out of the hole)
A5√2B6√3C8√2D7√5A tank, which is open at the top, contains a liquid up to a height H. A small hole is made in the side of the tank at a distance y below the liquid surface. The liquid emerging from the hole lands at a distance x from the tank :
 A2√h(H−h)B√h(H−h)C2√h2H−hD√h2H−h
A2√h(H−h)B√h(H−h)C2√h2H−hD√h2H−hA hole is made in the bottom of a container having water filled up to a height h. The velocity of water flowing out of the hole is :
Aindependent of h .Bproportional to h1/2Cproportional to hDproportional to h2
Water is filled in a cylindrical container to a height of 3m. The ratio of the cross-sectional area of the orifice and the beaker is 0.1. The square of the speed of the liquid coming out from the orifice is (g=10m/s2).
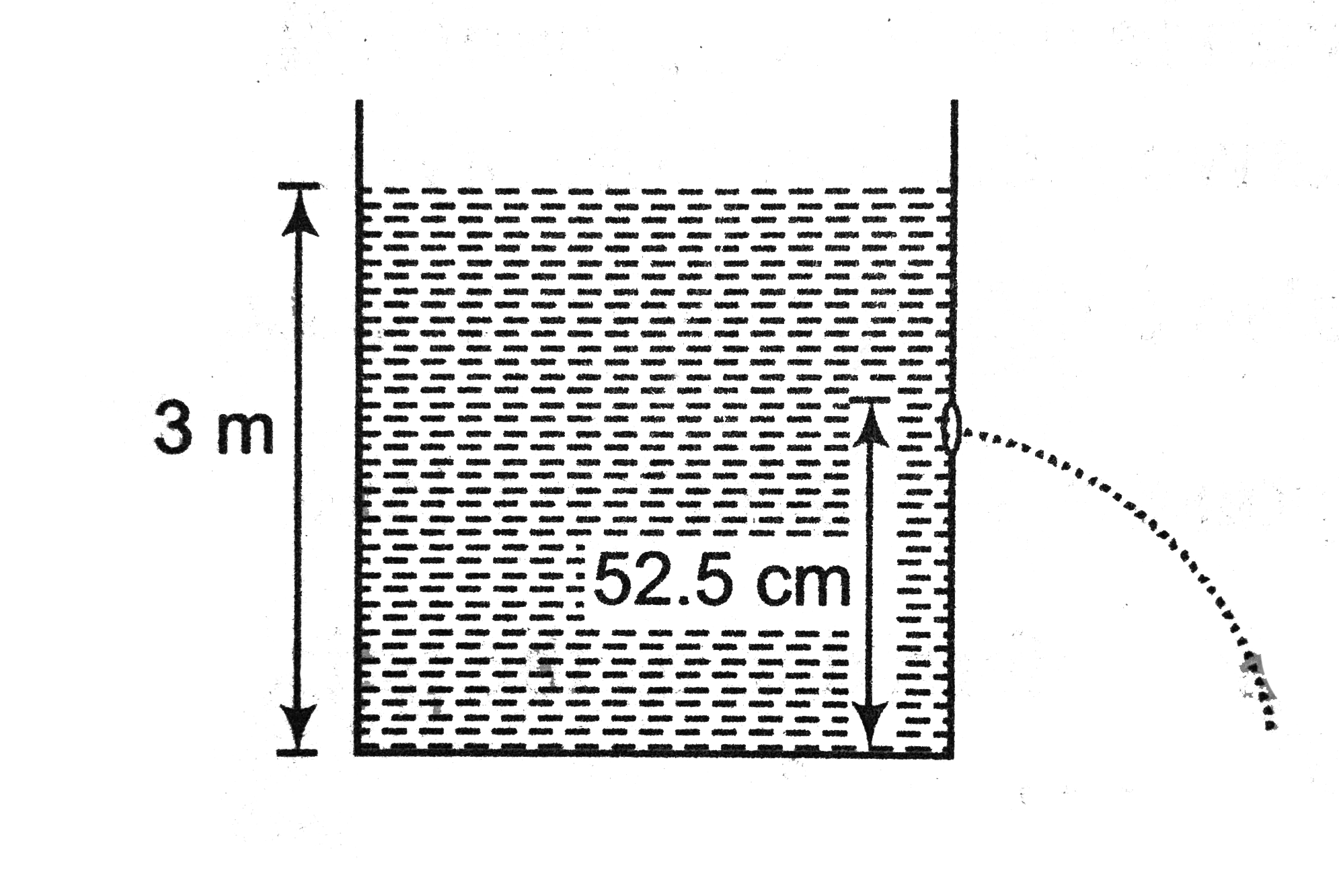
View SolutionWhat is filled in a cylindrical container to a height of 3m. The ratio of the cross-sectional area of the orifice and the beaker is 0.1. The square of the speed of the liquid coming out from the orifice is (g=10m/s2).
View SolutionA cylindrical tank is filled with water to a level of 3m. A hole is opened at a height of 52.5 cm from bottom. The ratio of the area of the hole to that of cross-sectional area of the cylinder is 0.1. The square of the speed with which water is coming out from the orifice is (Take g=10m/s2)
View SolutionIn a container, filled with water upto a height h, a hole is made in the bottom. The velocity of water flowing out of the hole is
View SolutionThe level of water in a tank is 5 m high . A hole of the area 10cm2 is made in the bottom of the tank . The rate of leakage of water from the hole is
View Solution
