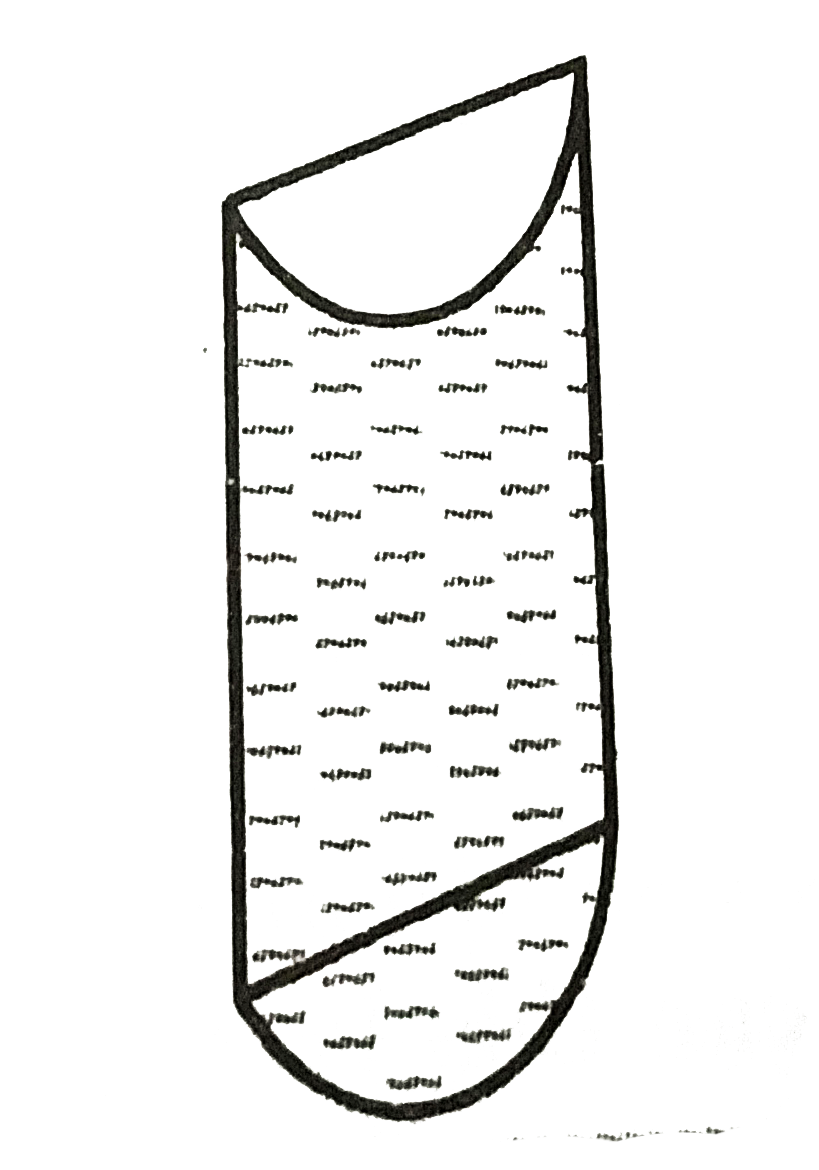
A liquid is contained in a vertical tube of semi-circular cross-section. The angle of contact is zero fig. What is the ratio of the force of surface tension on the curved part and the flat part of the tube?
2:π
1:π
3:π
2.7:π
The correct Answer is:A
Effective length of flat part = 2r
Effective length of curved part is πr
So, required ratio is 2:π
Topper's Solved these Questions
- BOOK - RESONANCECHAPTER - DAILY PRACTICE PROBLEMEXERCISE - DPP No.599videos
DAILY PRACTICE PROBLEM
- BOOK - RESONANCECHAPTER - DAILY PRACTICE PROBLEMEXERCISE - DPP No.609videos
DAILY PRACTICE PROBLEM
- BOOK - RESONANCECHAPTER - DAILY PRACTICE PROBLEMEXERCISE - DPP No.579videos
DAILY PRACTICE PROBLEM
- BOOK - RESONANCECHAPTER - CURRENT ELECTRICITYEXERCISE - High Level Problems (HIP)21videos
CURRENT ELECTRICITY
- BOOK - RESONANCECHAPTER - ELECTRO MAGNETIC WAVESEXERCISE - Exercise 327videos
ELECTRO MAGNETIC WAVES
Similar Questions
A liquid is containe in a vertical tube of semicircular cross section figure.The contact angle is zero. The force of surface tension on the curved part and on the flat part are in ratio
 View Solution
View SolutionA uniform vertical tube of circular crss section contaons a liquid. The contact angle is 90∘. Consider a diameter of the tube lying in the surface of the liquid. The surface of the right of this diameter pulls the surface on the left of it. What keeps the surface on the left in equilibrium?
View Solution
Knowledge Check
- Question 1 - Select One
A liquid is filled into a semi eliiptical cross section with a as semi major axis and b as semi minor axis. The rato of surface tension forces on the curved part and the plane part of the tube in vertical position will be
Aπ(a+b)4bB2πabCπa4bDπ(a−b)4b - Question 2 - Select One
A liquid is coming out from a vertical tube. The relation between the weight of the drop W , surface tension of the liquid T and radius of the tube r is given by, if the angle of contact is zero
AW=πr2TBW=2πrTCW=2r2πTDW=34πr3T - Question 3 - Select One
A liquid of density ρ and surface tension σ rises in a capillary tube of inner raduis R. The angle of contact between the liquid and the glass is θ. The point A lies just below the maniscus in the tube and the point B lies at the outside level of liquid in the beaker as shown in figure. The pressure at A is
 ApB−ρghBpB−2σcosθRCpatm−2σcosθRDAll of these
ApB−ρghBpB−2σcosθRCpatm−2σcosθRDAll of these
A long thin walled capillary tube of mass M and radius r is partially immersed in a liquid of surface tension T. The angle of contact for the liquid and the tube wall is 30∘. How much force is needed to hold the tube vertically? Neglect buoyancy force on the tube.
 View Solution
View Solution_S01_083_Q01.png)
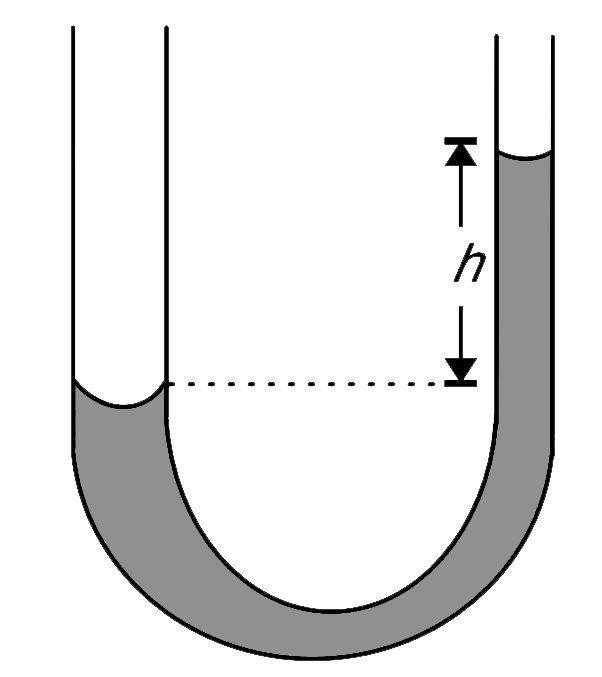
Water (density ρ) is flowing through the uniform tube of cross-sectional area A with a constant speed v as shown in the figure. Find the magnitude of force exerted by the water on the curved corner of the tube is (neglect viscous forces)View SolutionThe radii of two columns in a U tube are r1andr2(r1>r2). A liquid of density ρ is filled in it. The contact angle of the liquid with the tube wall is θ. If the surface tension of the liquid is T then plot the graph of the level difference (h) of the liquid in the two arms versus contact angle θ. Plot the graph for angle θ changing from 0∘→90∘. Assume the curved surface of meniscus to be part of a sphere.
 View Solution
View SolutionA liquid of density ρ comes out with a velocity v from a horizontal tube of area of cross-section A. The reaction force exerted by the liquid on the tube is F :
View SolutionA capillary tube of radius r is lowered into a liquid of surface tension T and density ρ. Given angle of contact =0∘.
The work done by surface tension will beView Solution

