A stone projected at angle 53∘ attains maximum height 25m during its motion in air. Then its distance from the point of projection where it will fall is
75m
4003m
50m
60m
The correct Answer is:A
velocity of projectile =50√54m/s (by appling equation v2=u2+2as in vertical direction )
time of flight =8v5g
distance travelled =vcos53∘×T=35v×85vg=75m (by putting the value of v )
Topper's Solved these Questions
Similar Questions
A body projected from a point O at an angle θ, just crosses a wall y m high at a distance. x m from the point of projection and strikes the ground at Q beyond the wall as shown, then find height of the wall
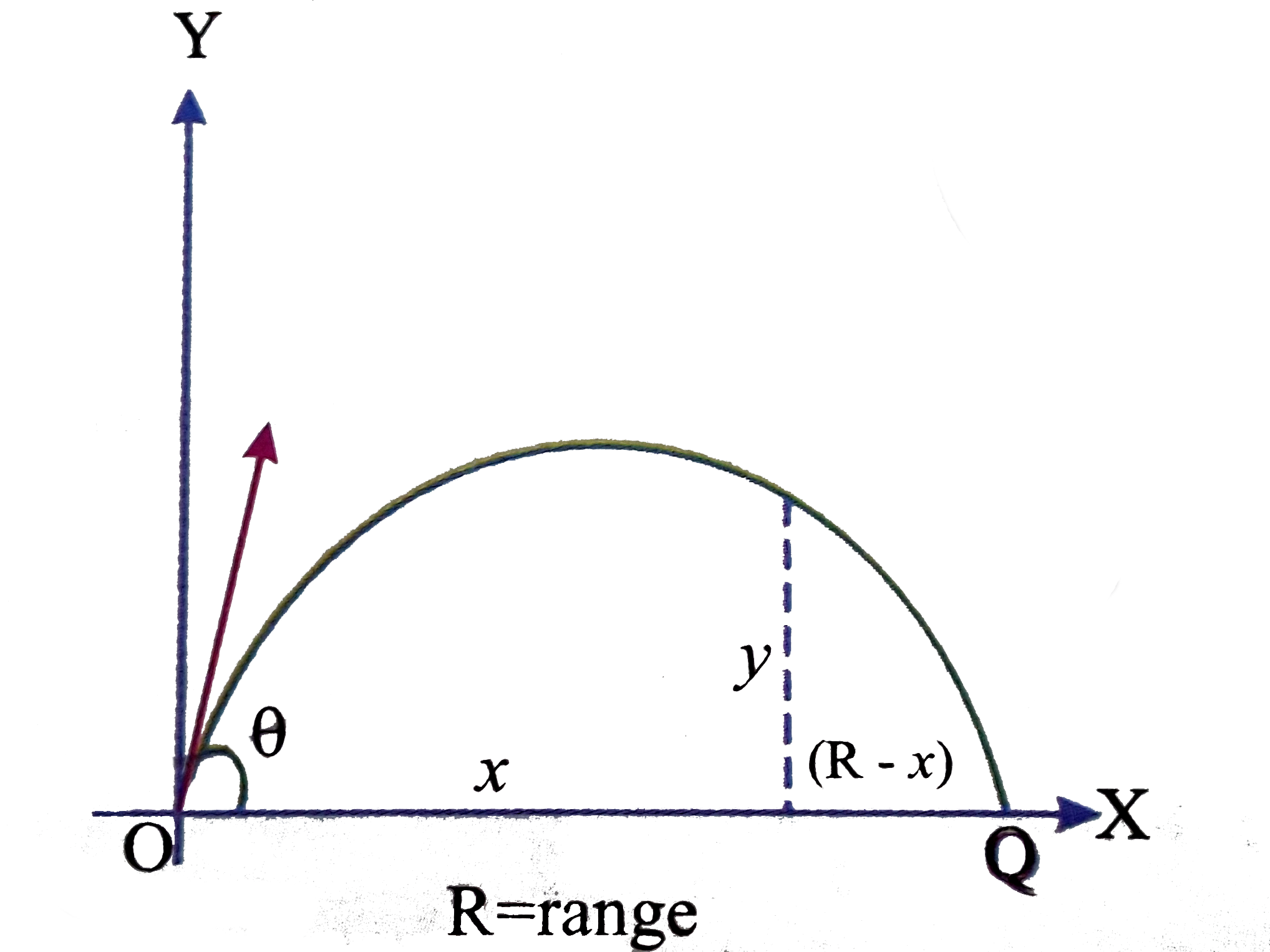
View SolutionA particle is projected from a point on the level ground and its height is h when at horizontal distances aand2a from its point of projection. Find the velocity of projection.
View Solution
Knowledge Check
Maximum height attained from the point of projection is:
A1.25 mB12.5 mC2025 mDNone of theseA ball is projected at an angle 45∘ with horizontal. It passes through a wall of height h at horizontal distance d1 from the point of projection and strikes the ground at a horizontal distance (d1+d2) from the point of projection, then
Ah=2dd_(2)d+d2Bh=d1d2d1+d2Ch=√2d1d2d1+d2Dh=d1d22(d1+d2)A body is projected obliquely from the ground such that its horizontal range is maximum.If the change in its maximum height to maximum height, is P, the change in its linear momentum as it travels from the point of projection to the landing point on the ground will be
APB√PC2PD2√2P
A particle just clear a wall of height b at a distance a and strikes the ground at a distance from the point of projection, the angle of projection is :
View SolutionA projectile is fired at an angle of 45∘ with the horizontal. Elevation angle of the projection at its highest point as seen from the point of projection is
View SolutionA body is projected obliquely from the ground such that its horizontal range is maximum. If the change in its linear momentum, as it moves from half the maximum height to maximum height, is P, the change in its linear momentum as it travels from the point of projection to the landing point on the ground will be:
View Solution
A stone is projected from a horizontal plane. It attains maximum height H & strikes a stationary smooth wall & falls on the ground vertically below the maximum height. Assuming the collision to be elastic the height of the point on the wall where ball will strike is
View SolutionA stone is thrown with a speed of 10ms−1 at an angle of projection 60∘. Find its height above the point of projection when it is at a horizontal distance of 3m from the thrower ? (Take g=10ms−2)
View Solution
