In the given figure a ball strikes a uniform rod of same mass elastically and rod is hinged at point A. Then which of the statements (s)is/are correct ?
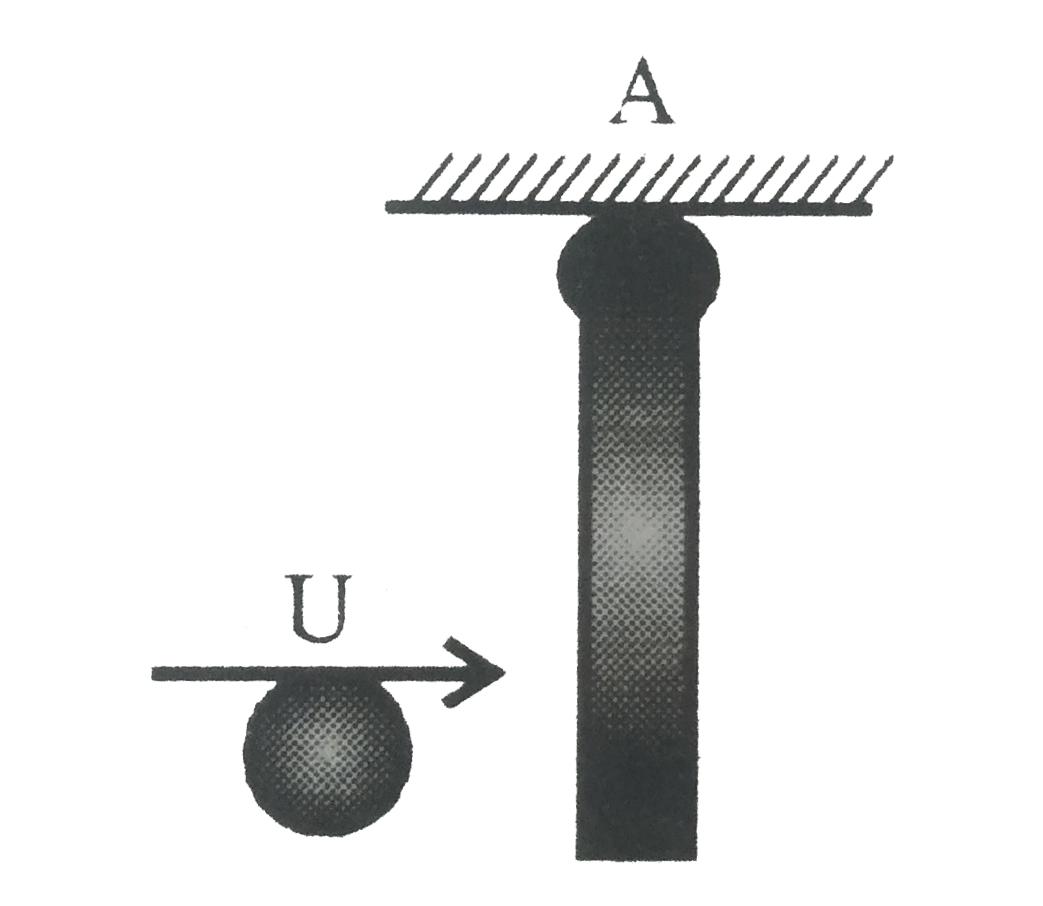 .
.
linear momentum of system (ball+rod) is conserved
angular momentum of system about hinged point A is not conserved
kinetic energy of system is conserved
linear momentum of ball is conserved.
The correct Answer is:C
Collision is elastic and work of hinge force =0 so K.E. energy of system is conserved
hinge force does not produce angular momentum about hinge so angular momentum about hinge is conserved. Due to impulse of hinge the linear momentum is not conserved.
Topper's Solved these Questions
Similar Questions
Knowledge Check
Figure shows a composite system of two uniform rods of lengths as indicated. Then the coordinate of the center of mass of the system of rods are
 A(L2,2L3)B(L4,2L3)C(L6,2L3)D(L6,L3)
A(L2,2L3)B(L4,2L3)C(L6,2L3)D(L6,L3)Two particles each of mass m are attached at end points of a massless rod AB of length l. Rod is hinged at point C as shown. Rod is released from rest from horizontal position. At the instant when rod reaches its vertical position as shown, which of the following is / are correct:
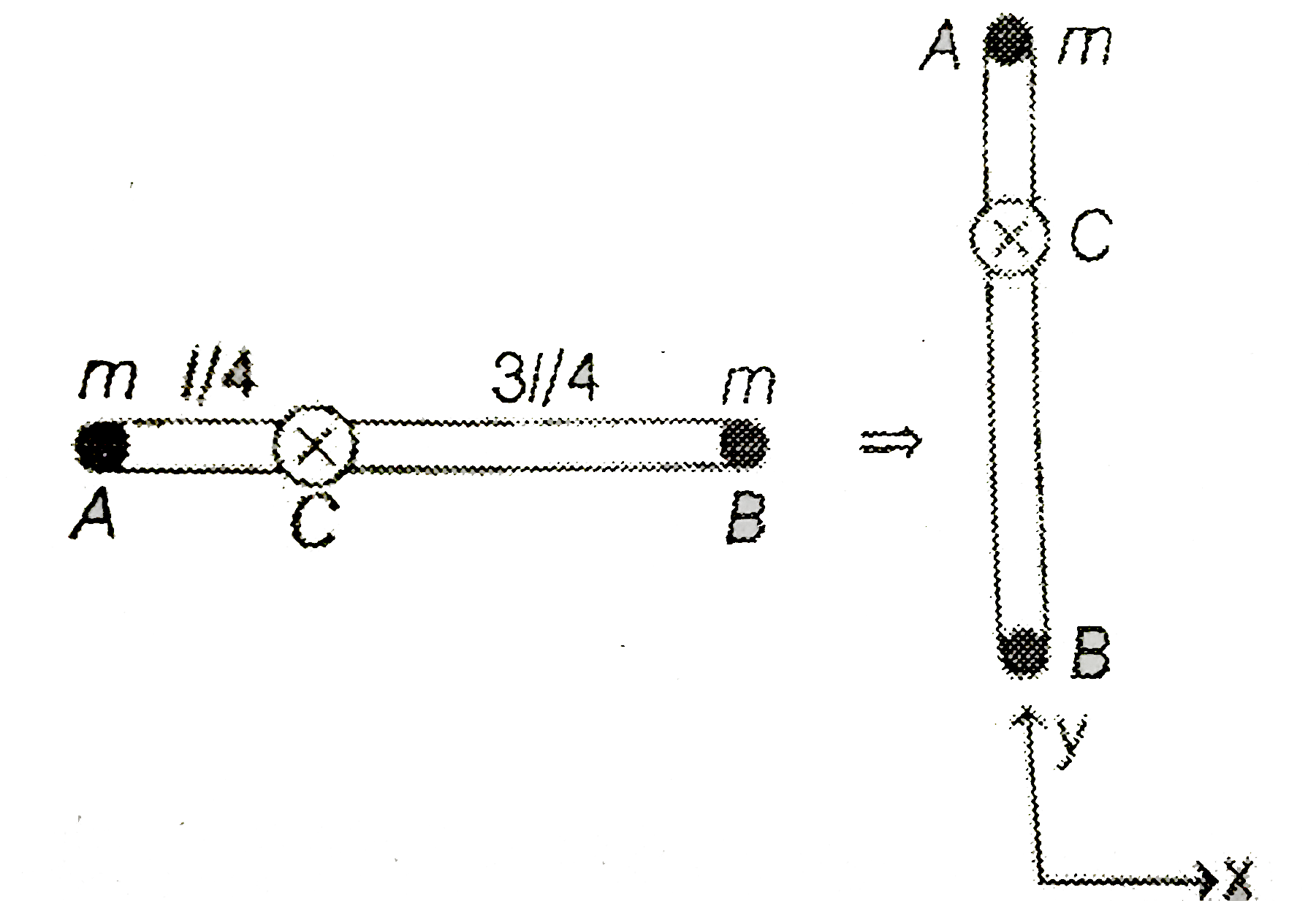 ASpeed of the particle at B is thrice the speed of particle at ABNet force on particle B is 6mg5CAngular acceleration of the system is zero.DBoth x and y components of hinge force are non-zero
ASpeed of the particle at B is thrice the speed of particle at ABNet force on particle B is 6mg5CAngular acceleration of the system is zero.DBoth x and y components of hinge force are non-zeroIn the figure shown, the spring are connected to the rod at one end and at the midpoint. The rod is hinged at its lower end. Rotational SHM of the rod (Mass m, length L) will occur only if-
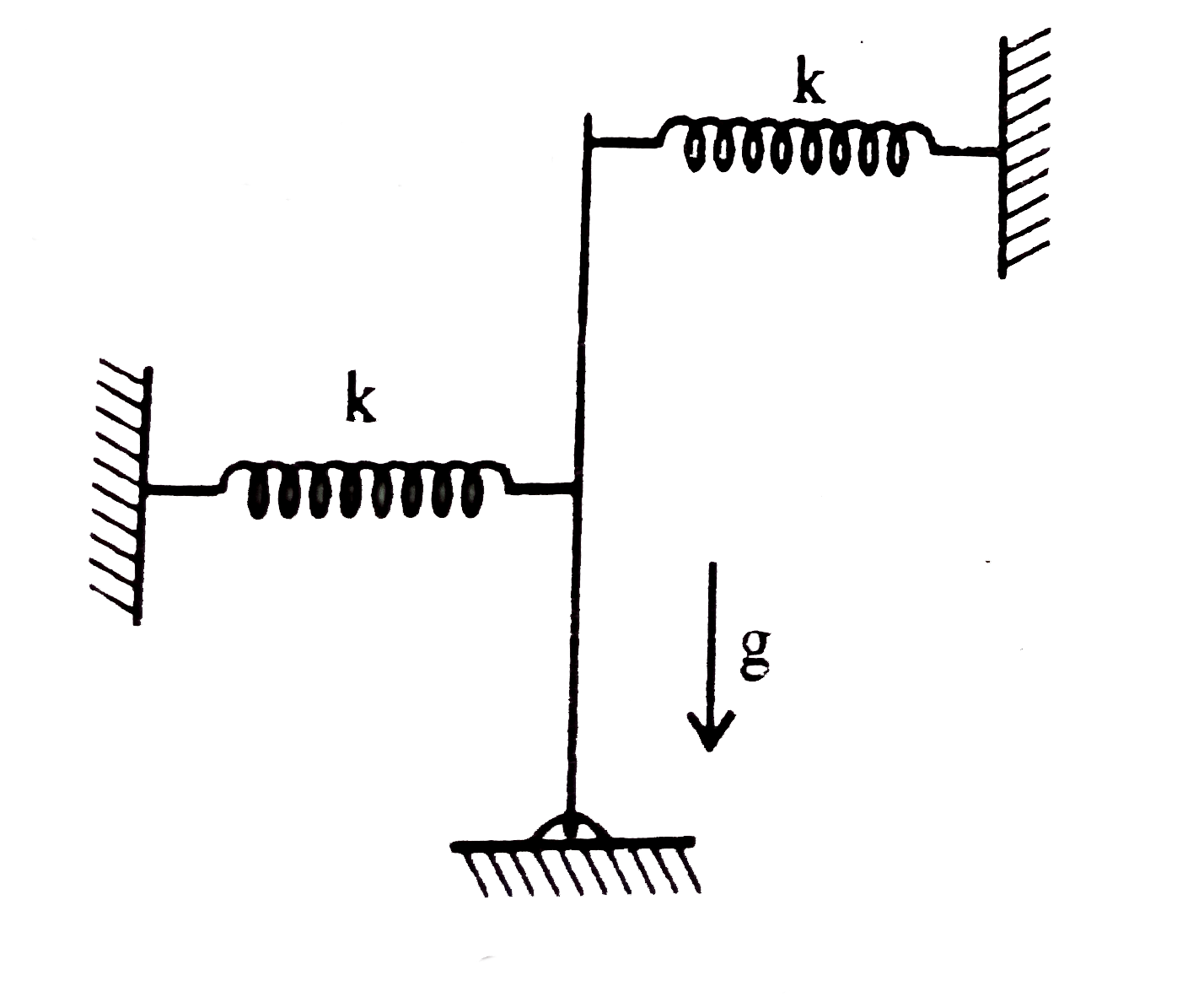 Ak>mg/3LBk>2mg/3LCk>2mg/5LDk=0
Ak>mg/3LBk>2mg/3LCk>2mg/5LDk=0
In the figure a uniform rod of mass 'm' and length 'l' is hinged at one end and the other end is connected to a light vertical of spring constant 'k' as shown in figure. The spring has extension such that rod is equilibrium when it is horizontal . The rod rotate about horizontal axis passing through end 'B'. Neglecting friction at the hinge find (a) extension in the spring (b) the force on the rod due to hinge.
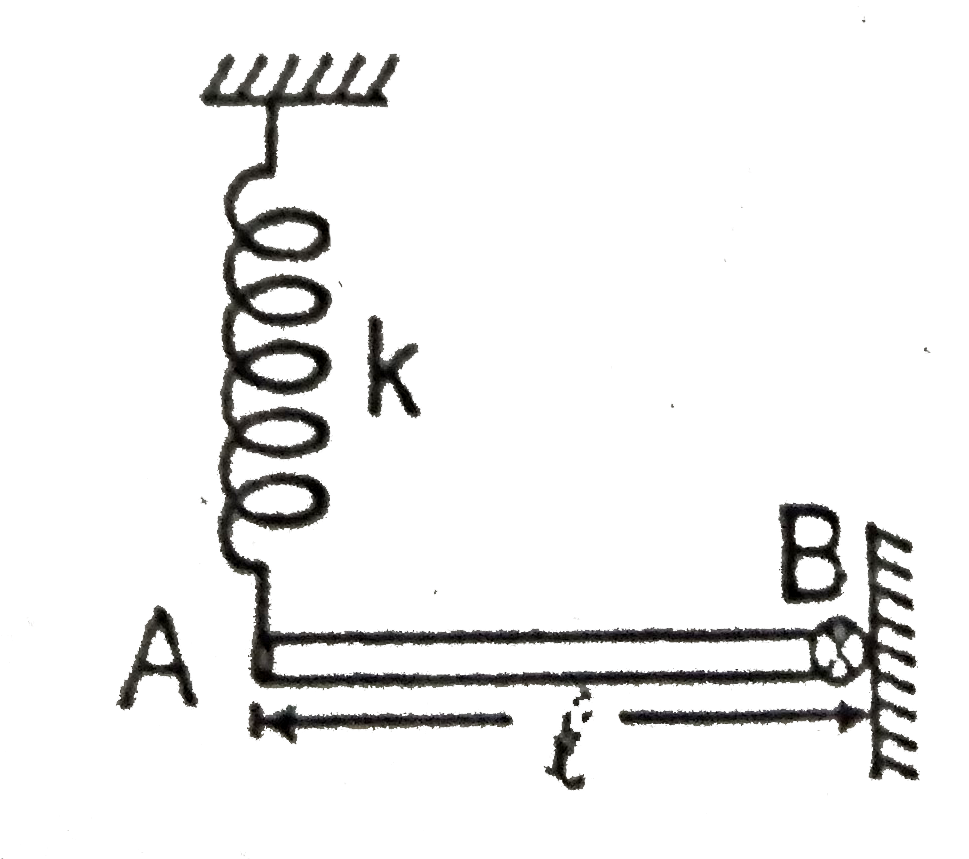
View SolutionFind the frequency of small oscillatinos of a thin uniform vertical rod of mass m and length l hinged at the point O (figure). The combined stiffness fo the springs is equal to x. The mass of the springs is negligible.
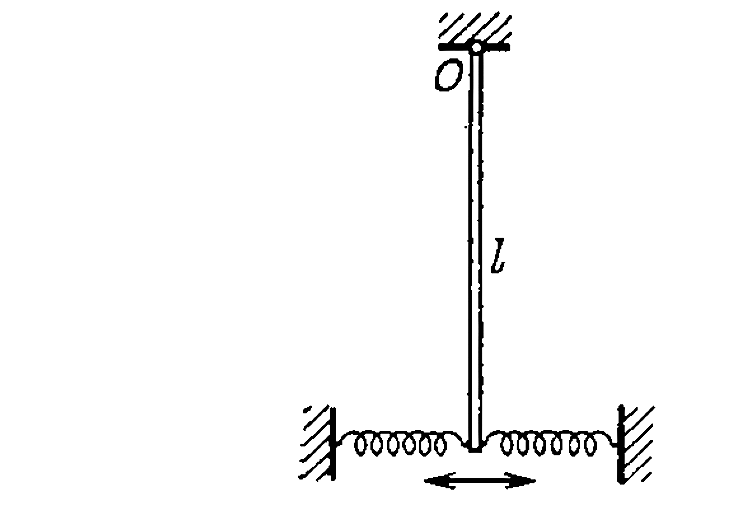
View SolutionCentre Of Mass Of Uniform Rod
View SolutionOne end of a uniform rod of mass M and length L is supproted by a frictionless hinge which can with stand a tension of 1.75Mg. The rod is free to rotate in a vertical plane. The maximum angle should the rod be rotated from the vertical position so that when left, the hinge does not brek is πn
View SolutionTwo uniform solid of masses m1 and m2 and radii r1 and r2 respectively, are connected at the ends of a uniform rod of length l and mass m. Find the moment of inertia of the system about an axis perpendicular to the rod and passing through a point at a distance of a from the centre of mass of the rod as shown in figure.

View Solution

