When a wire of uniform cross-section a, length I and resistance R is bent into a complete circle, resistance between two of diametrically opposite points will be
R4
R8
4R
R2
The correct Answer is:A
When wire is bent to form a complete circle then
2πr=R⇒r=R2π
Resistance of each semicircle =πr=πR2π=R2
Thus, net resistance in parallel combination of two semicircular resistance
R'=R2×R2R2+R2=R24R=R4
Topper's Solved these Questions
Similar Questions
Knowledge Check
When a wire of uniform cross-section a, length l and resistance R is bent into a complete circle, resistance between any two of diametrically opposite points will be
AR4BR8C4RDR2When a wire of uniform cross-section A, length 1 and resistance R is bent into a complete circle, resistance between two diametrically opposite points will be
AR4BR8C4RDR2A wire of resistance 10(Ω)is bent to form a complete circle.Find its resistance between two diametrically opposite points.
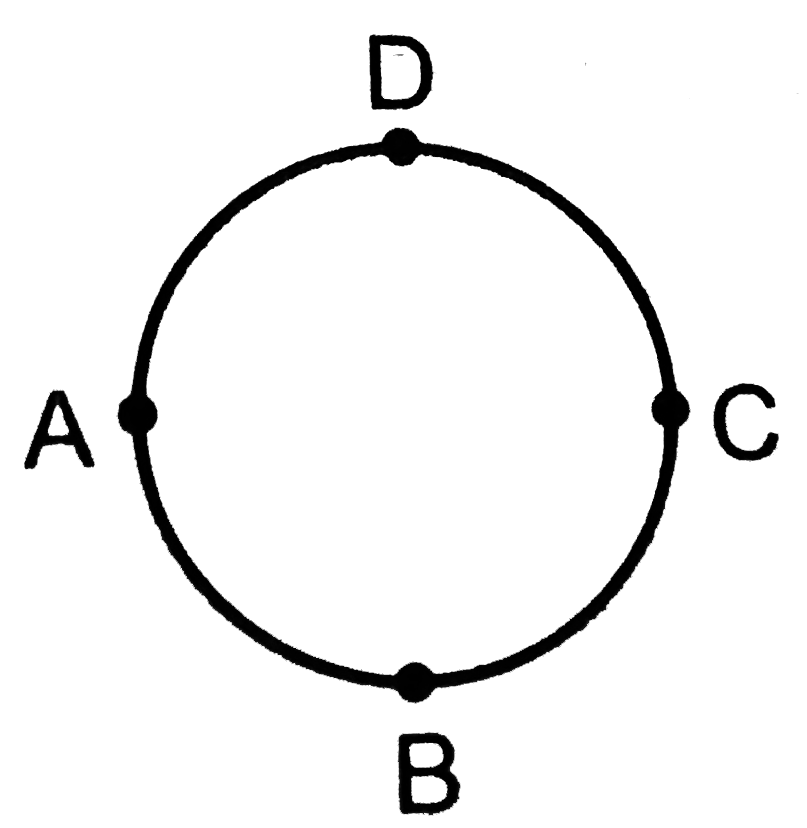 A1.5B2.5C3.5D4.5
A1.5B2.5C3.5D4.5
A metallic wire of resistance 12Ω is bent to form a square. The resistance between two diagonal points would be
View SolutionA metallic wire of resistance 12Ω is bent of form a square. The resistance between two diagonal points would be
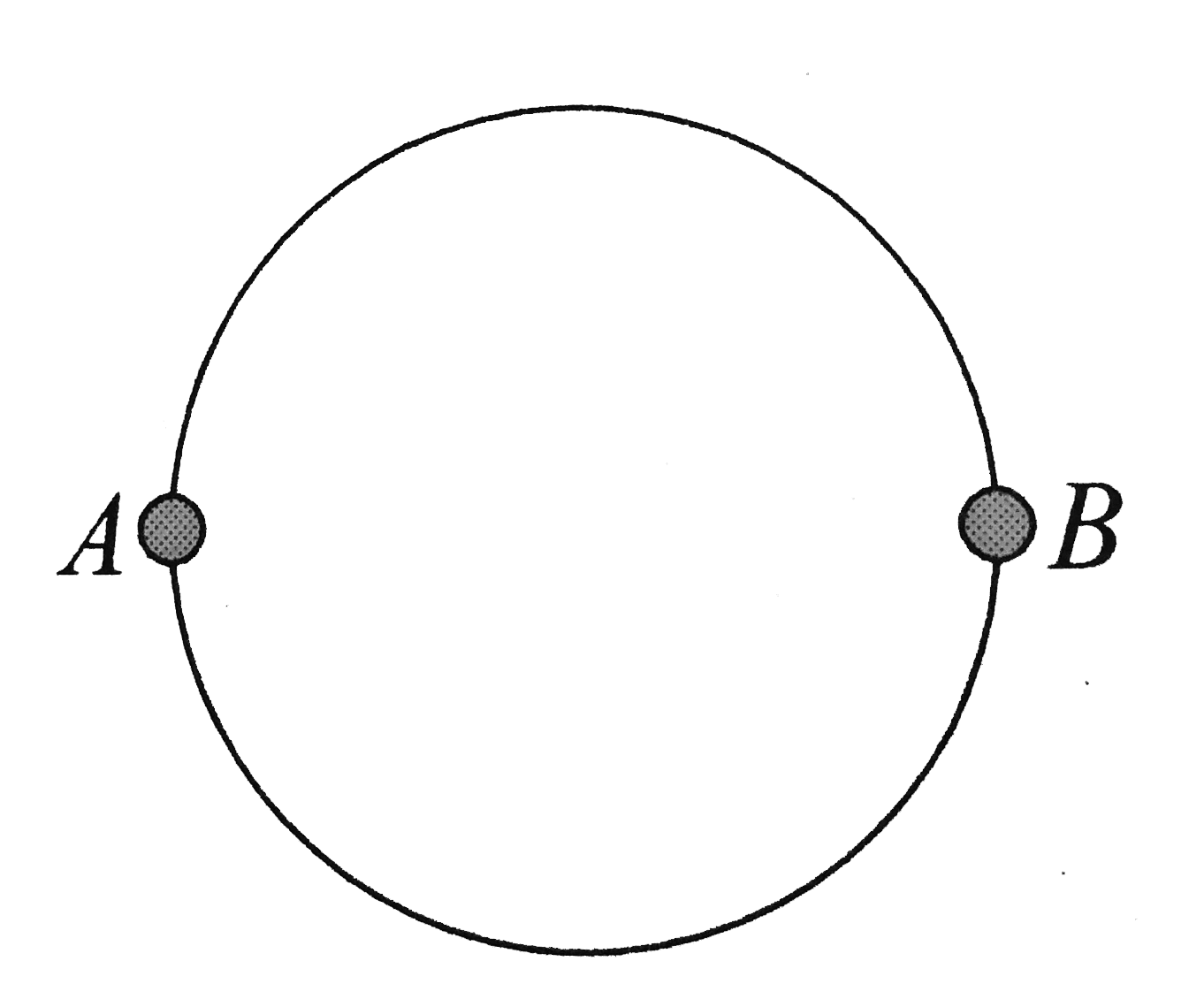
View SolutionA wire of resistance 12Ωm−1 is bent to from a complete circle of radius 10cm. The resistance between its two diametrically opposite points, A and B as shown in the figure, is
View SolutionA uniform wire of resistance 2R is bent in the form of a circle. The effective resistance between the ends of any diameter of the circle is
View SolutionA conducting wire of uniform cross section, having resistance R is bent to form a circle. O is the centre of this circle. Two points P and Q are selected on the ring in such a manner that ∠POQ is θ. If a battery of emf E and negligible internal resistance is connected between P and Q, then the current drawn from the battery would be
View Solution
