If y=5(mm)sinπt is equation of oscillation of source S1 and y2=5(mm)sinπ/6) be that of S2 and it takes 1sec and ^(1/2sec for the transverse waves to reach point A from source S1 and S2 respectively then the resulting amplitude at point A, is
_S01_006_Q01.png) .
.
5√2+√3mm
5√3mm
5mm
5√2mm
The correct Answer is:C
Wave originating at t=0 from S1 reaches point A at t=1
Wave originating at t=12 from S2 reaches point A at t=1
So phase difference in these waves =π2+π6,A=√A21+A22+2A1A2cosϕ=5.
Topper's Solved these Questions
Similar Questions
Find the ratio of intensities at the two points XandY on a screen in Young's double slit experiment, where waves from the two source S1andS2 have path difference of zero, and λ/4 respectively.
View SolutionThe phase difference between two waves reaching a point is π/2. What is the resultant amplitude, if the individual amplitudes are 3mmand4mm ?
View Solution
Knowledge Check
- Question 1 - Select One
Two waves are represented by y1=asin(ωt+π6)andy2=acosωt. What will be their resultant amplitude
AaB√2aC√3aD2a - Question 2 - Select One
In the case of light waves from two coherent sources S1 and S2, there will be constructive interference at an arbitrary point P, the path difference S1P−S2P is
A(n+12)λBnλC(n−12)λDλ2 - Question 3 - Select One
Ratio waves originating from sources S1 and S2 having zero phase difference and common wavelength λ will show completely destructive interference at a point P is S1P−S2P is
A5λB2λC3λ4D11λ2
The resultant loudness at a point P is n dB higher than the loudness of S1 whoch is one of the two identical sound sources S1 and S2 reaching at that point in phase. Find the value of n.
View SolutionTwo sources S1 and S2 separated 2.0 m, vibrate according to equation y1=0.03sinπt and y2=0.02sinπt where y1,y2 and t are in M.K.S unit. They send out waves of velocity 1.5 m/s. Calculate the amplitude of the resultant motion of the particle co-linear with S1 and S2 and located at a point to the left of S1.
View SolutionIn the figure shown A and B are two ends of a string of length 100m. S1 and S2 are two sources due to which point 'A' and 'B' oscillate in 'y' and 'z' directions respectively according to the equation y=2sin(100πt+30∘) and z=3sin(100πt+60∘) where t is in sec and y is in mm. The speed of propagation of disturbance along the string is 50m/s. Find the instantaneous positions vector (in mm) and velocity vector in (m/s) of a particle 'P' of string which is at 25m from A. You have to find these parameters after both the disturbance from S1 and S2 have reached 'P'. Also find the phase difference between the waves at the point 'P' when they meet at 'P' first time.
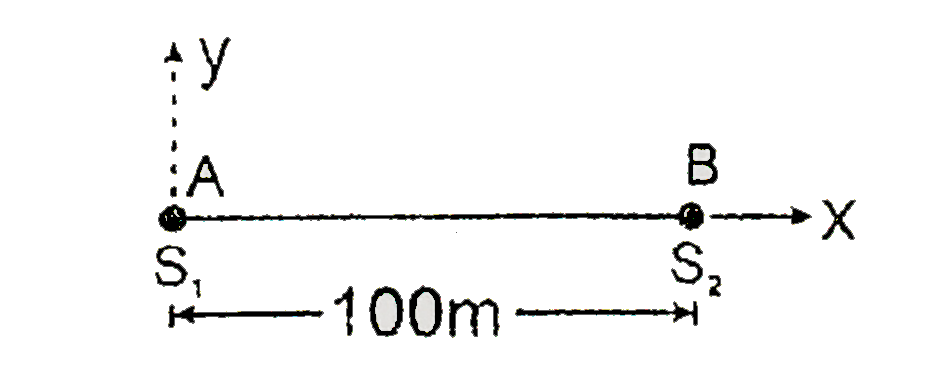 View Solution
View SolutionS1andS2 are two coherent sources of radiations separated by distance 100.25λ, where λ is the wave length of radiation. S1 leads S2 in phase by π/2.A and B are two points on the line joining S1andS2 as shown in figure.The ratio of amplitudes of component waves from source S1andS2 at A and B are in ratio 1:2. The ratio of intensity at A to that of B (IAIB) is
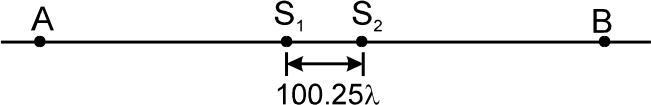 View Solution
View SolutionIf two waves represented by y1=4sinωt and y2=3sin(ωt+π3)interfere at a point, the amplitude of the resulting wave will be about
View Solution

