As shown, a big box of mass M is resting on a horziontal smooth florr. On the bottom of the box there is a small block of mass m. The block is given an intial speed v0 relative to the floor, and starts to bounce back and forth between the two walls of the box. Find the final speed of the box when the block has finally come to rest in the box :-

0
v0
mMv0
mm+Mv0
The correct Answer is:D
Fext= zero,
→P= constant
Pi=Pf
Finally moving together with velocity v
mv0=(m+M)v
v=mv0(m+M)
Topper's Solved these Questions
- CENTRE OF MASSBOOK - ALLENCHAPTER - CENTRE OF MASSEXERCISE - EXERCISE-II
43 Videos
- CENTRE OF MASSBOOK - ALLENCHAPTER - CENTRE OF MASSEXERCISE - EXERCISE-III
42 Videos
- CENTRE OF MASSBOOK - ALLENCHAPTER - CENTRE OF MASSEXERCISE - EXERCISE-V B
19 Videos
- BASIC MATHEMATICS USED IN PHYSICS &VECTORS BOOK - ALLENCHAPTER - BASIC MATHEMATICS USED IN PHYSICS &VECTORS EXERCISE - EXERCISE-IV ASSERTION & REASON
11 Videos
- ELASTICITY, SURFACE TENSION AND FLUID MECHANICSBOOK - ALLENCHAPTER - ELASTICITY, SURFACE TENSION AND FLUID MECHANICSEXERCISE - Exercise 5 B (Integer Type Questions)
3 Videos
Similar Questions
The block shown in figure is released from rest. Find out the speed of the block when the spring is compressed by 1 m
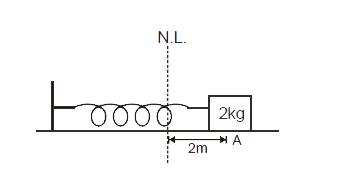
View SolutionA block os mass m moves on as horizontal circle against the wall of a cylindrical room of radius R. The floor of the room onwhich the block moves is smoth but the friction coefficient between the wall and the block is μ. The block is given an initial speed v0. As a function of the speed v write a. the normal force by the wall on the block. b. thefrictional force by the wall and c. the tangential acceleration of the block. d. Integrate the tangential acceleration (dvdt=vdvds) to obtain the speed of the block after one revoluton.
View Solution
Knowledge Check
A block of mass 1kg moving with a speed of 4ms−1, collides with another block of mass 2kg which is at rest. If the lighter block comes to rest after collision, then the speed of the heavier body is
A2ms−1B1ms−1C1.5ms−1D0.5ms−1The block of mass m is at rest. Find the tension in the string A .
 AmgB2 mgC3 mgD4 mg
AmgB2 mgC3 mgD4 mgA bullet of mass m moving with a speed v strikes a wooden block of mass M & gets embedded into the block. The final speed is
A√MM+nVB√mM+nVCmM+nVDV2
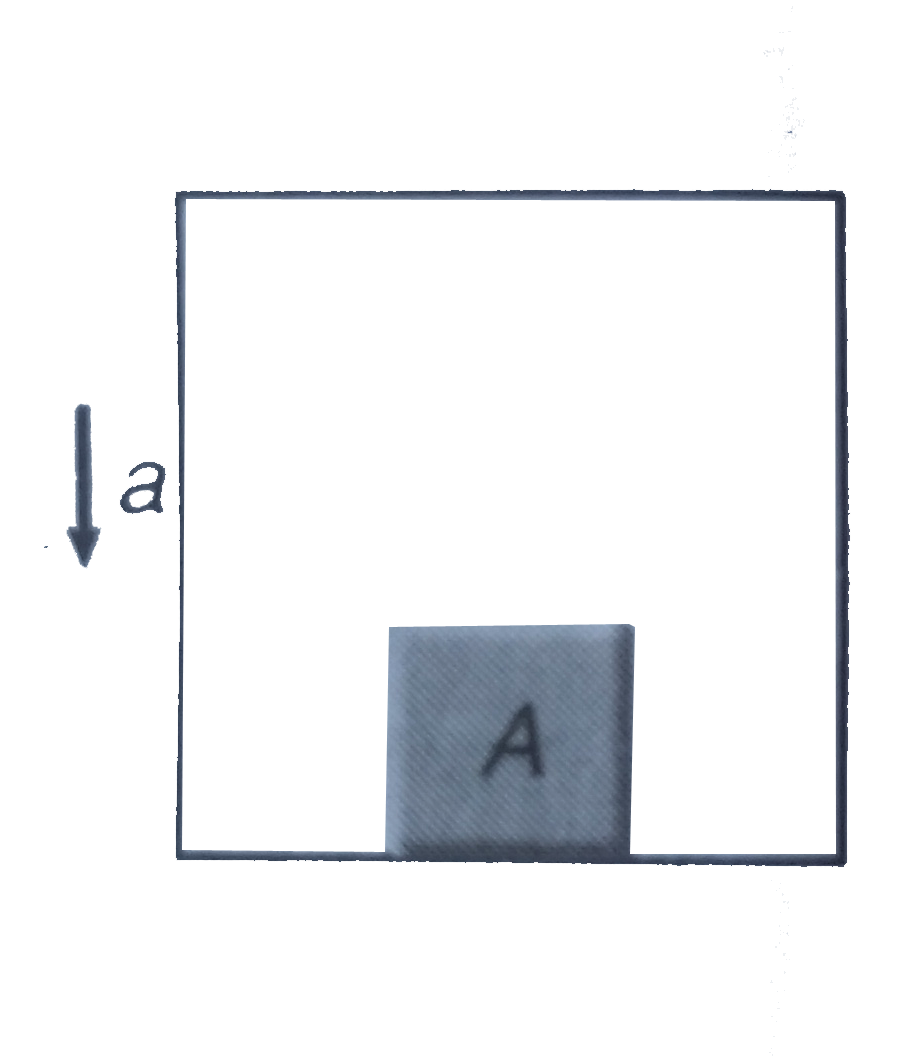
What should be the acceleration a of the box shown in figure so that the block of mass m exerts a force mg4 on the force of the box?
View SolutionA block of mass m moving at speed υ collides wilth another block of mass 2 m at rest. The lighter block comes to rest after the collision. Find the coefficient of restitution.
View SolutionFigure shows a small block of mass m which is started with a speed v on the horizontal part of the bigger block of mas m placed on a horizontal floor. The curved part of the surface shown is semicircular. All the surfaces are frictionless. Find the speed of the bigger block when the smaller block reaches the point A of the surface.

View SolutionConsider the situation as shown in the figure. The boy of mass M holds the light rope and the system is at rest. If m is mass of the box, find the force exerted by the boy on the rope and contact force beteween the boy and the box.

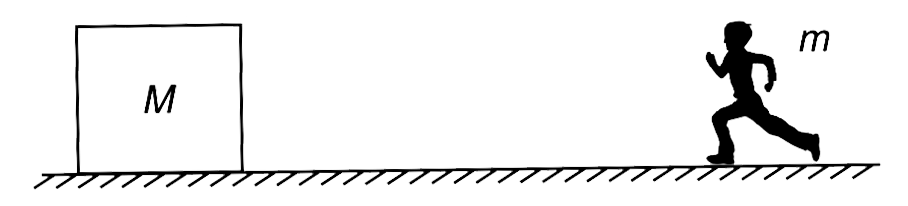
View SolutionA box of mass M is at rest on a horizontal surface. A boy of mass m(<M) wants to push the box by applying a horizontal force on it. The boy knows that he will not be able to push the box as the coefficient of friction μ between his shoes and ground is almost equal to that between the box and the ground. He decides to run, acquire a speed u and then bang into the box. After hitting the box, the boy keeps pushing as hard as possible. What is the maximum distance through which the box can be displaced this way ?
View Solution
