For three events $A, B, C$ the probability that only $A$ occur is (A) $P (A)$ (B) $P (A \cup B \cup C)$ (C) $P (A' \cap B' \cap C')$ (D) $P (A \cap B' \cap C')$

Video Solution

Answer

Step by step video & image solution for For three events A,B,C the probability that only A occur is (A) P(A) (B) P(AuuBuuC) (C) P(Aprime nn B prime nn C prime) (D) P(A nn B prime nn C prime) by Maths experts to help you in doubts & scoring excellent marks in Class 12 exams.

Updated on:21/07/2023

Class 12 MATHS DEFAULT

Similar Questions

If $A$ and $B$ are two events, then which of the following does not represent the probability that exactly one of $A, B$ occurs is.
(a) $P (A) + P (B) - P (A \cap B)$
(b) $P (A \cap B') + P (A' \cap B)$
(c) $P (A') + P (B') - 2 P (A' \cap B')$
(d) $P (A) + P (B) - 2 P (A \cap B)$
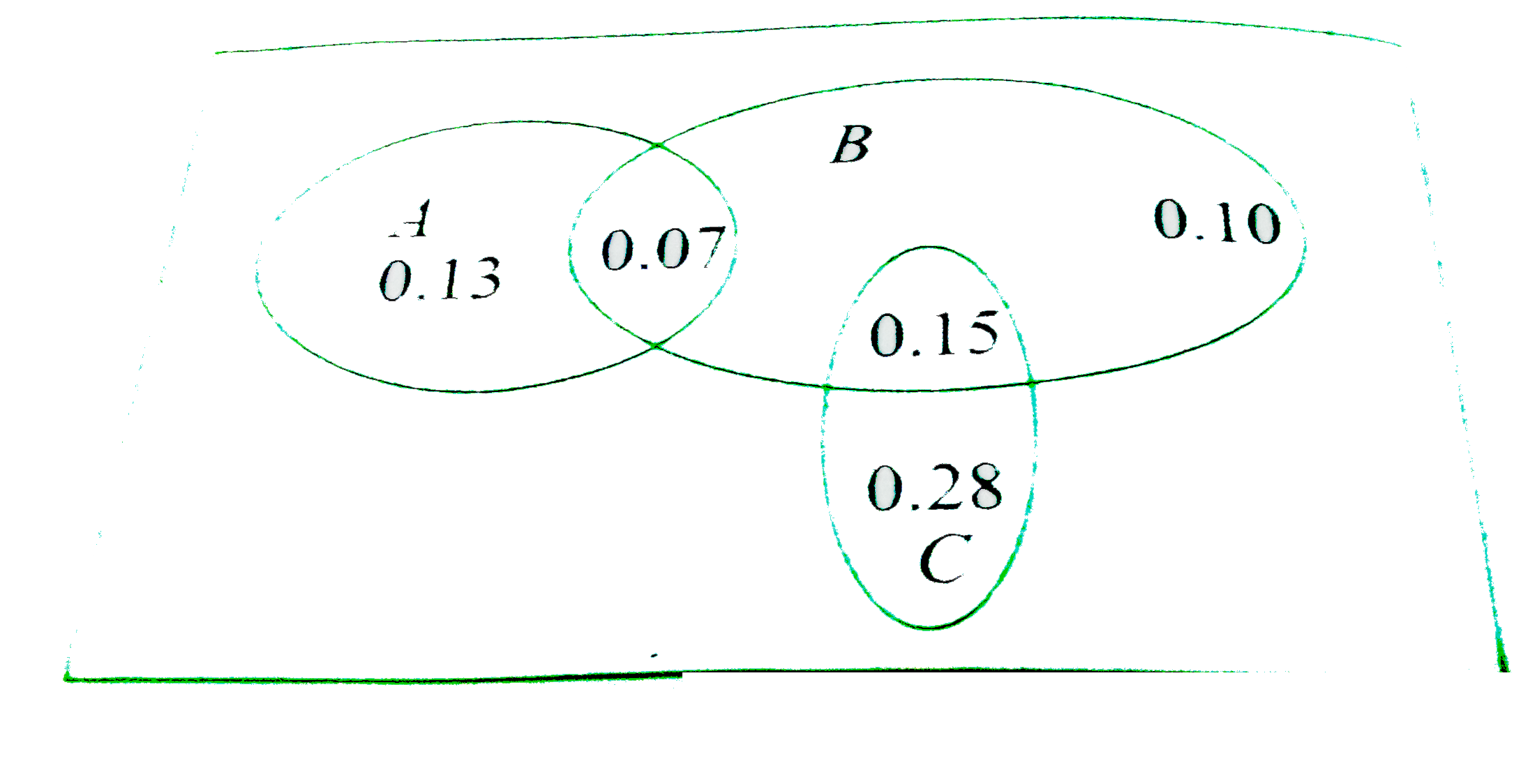
View Solution
The following Venn diagram shows three events, A, B, and C, and also the probabilities of the various intersections.
Determine
(a) P(A)
(b) $P (B \cap ¯ ¯ ¯ C)$
(c ) $P (A \cup B)$
(d) $P (A \cap ¯ ¯ ¯ B)$
(e) $P (B \cap C)$
(f) Probability of the event that exactly one of A, B, and C occurs.

View Solution
The following Venn diagram shows three events, A, B, and C, and also the probabilities of the various intersections.
Determine
(a) P(A)
(b) $P (B \cap ¯ ¯ ¯ C)$
(c ) $P (A \cup B)$
(d) $P (A \cap ¯ ¯ ¯ B)$
(e) $P (B \cap C)$
(f) Probability of the event that exactly one of A, B, and C occurs.

View Solution
For any three events A, B and C, prove that, $P (A \cup B \cup C) = P (A) + P (B) + P (C) - P (A \cap B) - P (B \cap C) - P (C \cap A) + P (A \cap B \cap C)$ .
View Solution
If $A a n d B$ are mutually exclusive events such that $P (A) = 0.35 a n d P (B) = 0.45$ , find $P (A \cup B)$ (ii) $P (A \cap B)$ (iii) $P (A \cap B^{'})$ (iv) $P (A^{'} \cap B^{'})$
View Solution
If $A a n d B$ are mutually exclusive events such that $P (A) = 0.35 a n d P (B) = 0.45$ , find $P (A \cup B)$ (ii) $P (A \cap B)$ (iii) $P (A \cap B^{'})$ (iv) $P (A^{'} \cap B^{'})$
View Solution
There are three events A, B, C such that $n (B) = 230, n (¯ ¯ ¯ A \cap B \cap ¯ ¯ ¯ C) = 100, n (A \cap B \cap ¯ ¯ ¯ C) = 50$ and $n (A \cap B \cap C) = 10$ , then value of $n (A \cap B \cap C)$ is
View Solution
The probabilities of three events $A, B, C$ are such that $P (A) = 0.3, P (B) = 0.4, P (C) = 0.8, P (A \cap B) = 0.09, P (A \cap C) = 0.28, P (A \cap B \cap C) 0.08 and P (A \cup B \cup C) \leq 0.75$ .Show that $P (B \cap C)$ lies in the interval $[0.21, 0.46]$ .
View Solution
If A and B are two sets then $A \cap (B - C) = (A \cap B) - (A \cap C)$
View Solution
If A, B, C are three events associated with a random experiment prove that $P (A \cup B \cup C) = P (A) + P (B) + P (C) - P (A \cap C) - P (B \cap C) + P (A \cap B \cap C)$
View Solution

For three events A,B,C the probability that only A occur is (A) P(A) (B) P(A∪B∪C) (C) P(A'∩B'∩C') (D) P(A∩B'∩C')

Similar Questions

Recommended Questions

For three events $A, B, C$ the probability that only $A$ occur is (A) $P (A)$ (B) $P (A \cup B \cup C)$ (C) $P (A' \cap B' \cap C')$ (D) $P (A \cap B' \cap C')$