Gecilia leaves home for school on her bike at 9:05 AM, riding at an average speed of 10 miles per hour. Fifteen minutes later, her mother realizes that Cecilia forgot to take her lunch. Cecilia's mother immediately gets into her car and drives after Cecilia at an average speed of 25 miles per hour. At what time will Cecilia mom catch up to her?
9:15A
9:20A
9:26A
9:30A
The correct Answer is:D
To solve the problem step by step, we can follow these calculations:
Step 1: Determine the time Cecilia rides before her mother starts.
Cecilia leaves home at 9:05 AM, and her mother realizes she forgot the lunch 15 minutes later. Therefore, Cecilia has been riding for:
- 9:05 AM + 15 minutes = 9:20 AM
Step 2: Calculate the distance Cecilia travels by the time her mother starts.
Cecilia rides at an average speed of 10 miles per hour. The time she rides before her mother starts is 15 minutes, which we convert to hours:
- 15 minutes = 15/60 hours = 0.25 hours
Now, we can calculate the distance (d) Cecilia travels:
- Distance (d) = Speed × Time
- d = 10 miles/hour × 0.25 hours = 2.5 miles
Step 3: Set up the equations for the distances traveled.
Let t be the time in hours that Cecilia's mother drives until she catches up to Cecilia. In this time, Cecilia will continue to ride her bike. The distance Cecilia travels during this time is:
- Distance = Speed × Time = 10 miles/hour × (t + 0.25 hours)
Cecilia's mother starts driving 15 minutes (or 0.25 hours) later than Cecilia, so the time she drives is t hours. The distance her mother travels is:
- Distance = Speed × Time = 25 miles/hour × t
Step 4: Set the distances equal to each other.
Since both Cecilia and her mother will have traveled the same distance when they meet:
- 10(t + 0.25) = 25t
Step 5: Solve for t.
Expanding the left side:
- 10t + 2.5 = 25t
Now, rearranging the equation:
- 2.5 = 25t - 10t
- 2.5 = 15t
- t = 2.5 / 15
- t = 0.1667 hours (approximately)
Step 6: Convert t to minutes.
To convert hours to minutes, multiply by 60:
- t = 0.1667 hours × 60 minutes/hour = 10 minutes
Step 7: Calculate the time when Cecilia's mother catches up.
Cecilia's mother starts driving at 9:20 AM and drives for approximately 10 minutes:
- 9:20 AM + 10 minutes = 9:30 AM
Final Answer:
Cecilia's mother will catch up to her at 9:30 AM.
---
Cecilia leaves home at 9:05 AM, and her mother realizes she forgot the lunch 15 minutes later. Therefore, Cecilia has been riding for:
- 9:05 AM + 15 minutes = 9:20 AM
Cecilia rides at an average speed of 10 miles per hour. The time she rides before her mother starts is 15 minutes, which we convert to hours:
- 15 minutes = 15/60 hours = 0.25 hours
- Distance (d) = Speed × Time
- d = 10 miles/hour × 0.25 hours = 2.5 miles
Let t be the time in hours that Cecilia's mother drives until she catches up to Cecilia. In this time, Cecilia will continue to ride her bike. The distance Cecilia travels during this time is:
- Distance = Speed × Time = 10 miles/hour × (t + 0.25 hours)
- Distance = Speed × Time = 25 miles/hour × t
Since both Cecilia and her mother will have traveled the same distance when they meet:
- 10(t + 0.25) = 25t
Expanding the left side:
- 10t + 2.5 = 25t
- 2.5 = 25t - 10t
- 2.5 = 15t
- t = 2.5 / 15
- t = 0.1667 hours (approximately)
To convert hours to minutes, multiply by 60:
- t = 0.1667 hours × 60 minutes/hour = 10 minutes
Cecilia's mother starts driving at 9:20 AM and drives for approximately 10 minutes:
- 9:20 AM + 10 minutes = 9:30 AM
Cecilia's mother will catch up to her at 9:30 AM.
Topper's Solved these Questions
Similar Questions
Etiennne is graphing the quadratic equation y=x2−8x−48. He substtitute 0 for x and finds that the y-intercept of the graph is-48. Next, he wants to plot the x-intercepts of the graph, so he rewrites the equation in a different form. Assuming he rewrote the equations correctly and the equation reveals the x-intercepts, which of the following Etienne's new equations?
01:27View Solution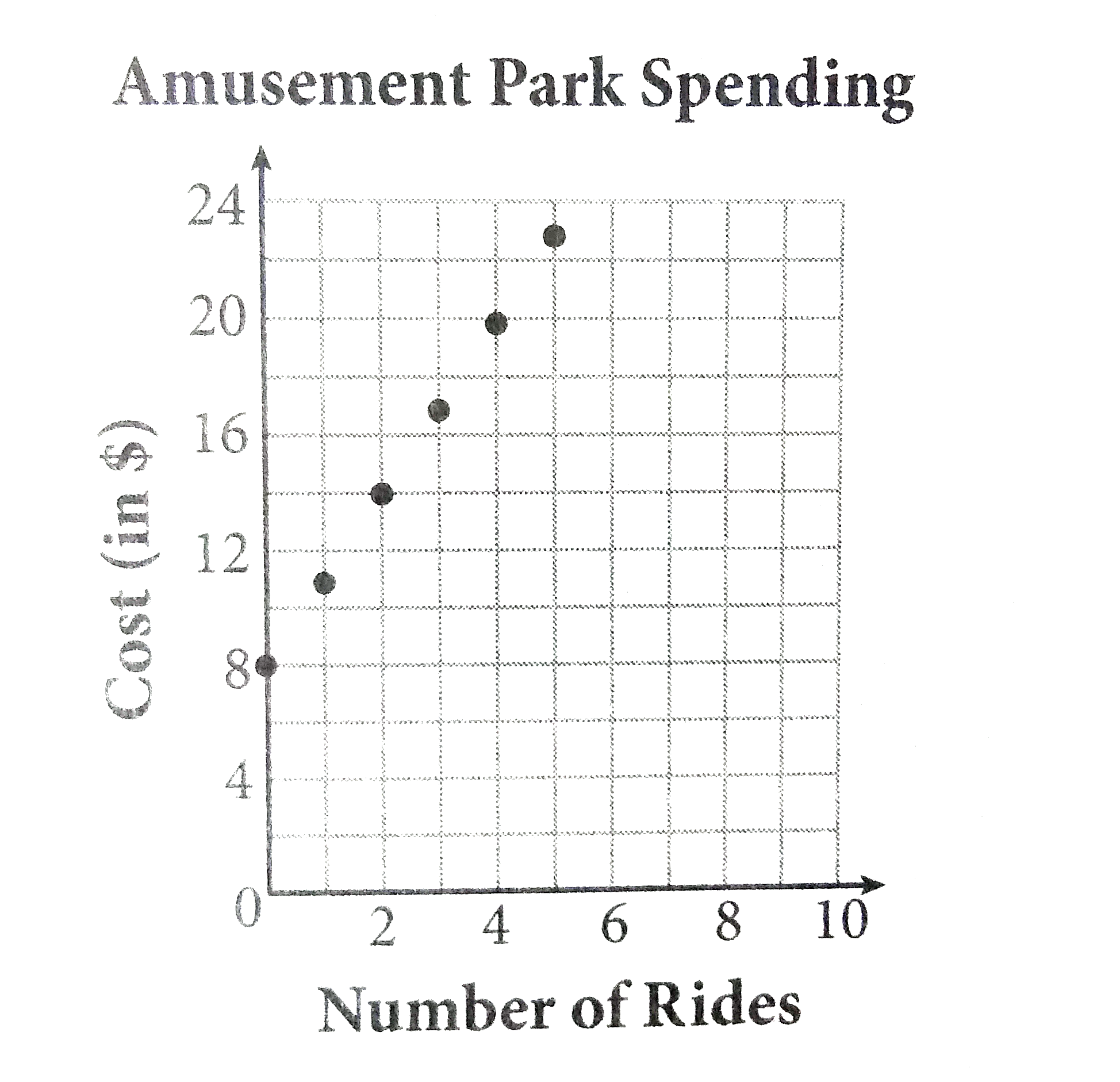
Wesley went to an amusement park with his family. He paid for his own admission ticket and all of the rides he rode. The scatterplot shows possible amounts that he could have paid. If a line of best fit (not shown) is used to model the data, the equation of the line would by y=3x+8. In this scenario, what does 3 represent?03:46View SolutionShalu cycles to her school at an average speed of 12 km/hr. It takes her 20 minutes to reach the school. If she wants to reach her school in 15 minutes, what should be her average speed?
07:48View Solutionएक छात्रा अपने घर से विधालय जाने में 30 मिनट तथा विधालय से घर वापस में भी 30 मिनट का समय लगाती है यदि उसके विधालय से घर की दूरी 5 किमी हो तो बताइए की छात्रा की औसत चाल तथा औसत वेग कितना होगा ?
View SolutionKayla drove from Bayside to Chatham at a constant speed of 21 miles per hour and then returned along the same route from Chatham to Bayside. If her average speed for the entire journey was 26.25 miles per hour, at what average speed, in miles per hour, did Kayla return from Chatham to Bayside ?
01:19View SolutionPaula jogged for a total of 30 minutes. Her average speed for the first 10 minutes was 5 miles per hour. During the remainder of her time, she jogged 2.5 miles. What was Paula's average for her entire jog ?
03:31View SolutionA man drove to work at an average rate of speed of 60 miles per hour and returned over the same route driving at an average rate of speed of 40 miles per hour. If his total driving time was 1 hour, what was the total number of miles in the round trip?
04:49View SolutionA man drove a car at an average rate of speed of 45 miles per hour for the first 3 hours of a 7-hour car trip. If the average rate speed for the entire trip was 53 miles per hour, what was the average rate of speed in miles per hour for the remaining part of the trip?
View SolutionLauren rode her bike from her house to a friend's house 312 miles away. On the first leg of her trip , she rode uphill at 3 miles per hour. The second part of the trip covered a larger distance but was downhill, and Lauren rode at 5 miles per hour. If the downhill part of the ride took half an hour, how many minutes did the uphill part take ?
01:05View SolutionAmy drove the 200 miles to New Orleans at at average speed 10 miles per hour faster than her usual average speed. If the complwrws the trip in 1 hour less than usual, what is her usual driving speed, in miles per hour?
06:17View SolutionIf Edna drove s miles in t hours, which of the following represents her average speed, in miles per hour?
04:39View SolutionJuan bikes halfway to school at 9 miles per hour, and walks the rest of the distance at 3 miles per hour. What is Juan's average speed for the whole trip?
03:16View SolutionA car travels from Twon A to Twon B at an average speed of 40 miles per hour, and returns immediately along the same route at an average speed of 50 miles per hour. What is the average speed in miles per hour for the round - trip?
02:10View SolutionShalu cycles to her school at an average speed of 12 km/hr. It takes her 20 minutes to reach the school. If she wants to reach her school in 15 minutes, what should be her average speed?
02:08View SolutionGita rides with her father’s bike to her uncle’s house which is 40 km away from her home. She takes 40 minutes to reach there.
Statement 1 : She travels with a speed of 1km/minute.
Statement 2 : She travels with a speed of 1km/hour.02:16View Solution

