A uniform rod of length 4 m and mass 20 kg is lying horizontal on the ground. The work done in keeping it vertical with one of its ends touching the ground, will be -
784 J
392 J
196 J
98 J
To find the work done in keeping a uniform rod of length 4 m and mass 20 kg vertical with one of its ends touching the ground, we can follow these steps:
Step 1: Understand the initial and final positions of the rod
- The rod is initially lying horizontally on the ground.
- When we raise it to a vertical position, one end remains on the ground, and the center of gravity of the rod is lifted.
Step 2: Identify the center of gravity
- For a uniform rod, the center of gravity is located at its midpoint.
- Given the length of the rod (L = 4 m), the center of gravity will be at L/2 = 4 m / 2 = 2 m from the end that is on the ground.
Step 3: Calculate the height the center of gravity is raised
- When the rod is raised to a vertical position, the center of gravity moves from a height of 0 m (when lying horizontally) to a height of 2 m (when vertical).
- Thus, the height (h) through which the center of gravity is raised is 2 m.
Step 4: Use the work done formula
- The work done (W) against gravity to raise the center of gravity can be calculated using the formula:
W=mgh
where:
- m is the mass of the rod (20 kg),
- g is the acceleration due to gravity (approximately 9.8 m/s²),
- h is the height raised (2 m).
Step 5: Substitute the values into the formula
- Substitute the values into the formula:
W=20kg×9.8m/s2×2m
Step 6: Calculate the work done
- Performing the calculation:
W=20×9.8×2=392Joules
Step 7: Determine the sign of the work done
- Since we are doing work against gravity to lift the center of gravity, the work done is positive. However, if we consider the work done by the gravitational force, it would be negative. In this context, the work done by us to lift the rod is 392 Joules.
Final Answer
The work done in keeping the rod vertical with one of its ends touching the ground is 392 Joules.
---
- The rod is initially lying horizontally on the ground.
- When we raise it to a vertical position, one end remains on the ground, and the center of gravity of the rod is lifted.
- For a uniform rod, the center of gravity is located at its midpoint.
- Given the length of the rod (L = 4 m), the center of gravity will be at L/2 = 4 m / 2 = 2 m from the end that is on the ground.
- When the rod is raised to a vertical position, the center of gravity moves from a height of 0 m (when lying horizontally) to a height of 2 m (when vertical).
- Thus, the height (h) through which the center of gravity is raised is 2 m.
- The work done (W) against gravity to raise the center of gravity can be calculated using the formula:
W=mgh
- m is the mass of the rod (20 kg),
- g is the acceleration due to gravity (approximately 9.8 m/s²),
- h is the height raised (2 m).
- Substitute the values into the formula:
- Performing the calculation:
- Since we are doing work against gravity to lift the center of gravity, the work done is positive. However, if we consider the work done by the gravitational force, it would be negative. In this context, the work done by us to lift the rod is 392 Joules.
The work done in keeping the rod vertical with one of its ends touching the ground is 392 Joules.
Topper's Solved these Questions
Similar Questions
One fourth length of a uniform rod of length 2l and mass m is place don a horizontal table and the rod is held horizontal. The rod is released from rest. Find the normal reaction on the rod as soon as the rod is released.
 View Solution
View Solution
A uniform rod of length 4l and mass m is free to rotate about a horizontal axis passing through a point distant l from its one end. When the rod is horizontal its angular velocity is ω as shown in figure. calculate
(a). reaction of axis at this instant,
(b). Acceleration of centre of mass of the rod at this instant.
(c). reaction of axis and acceleration of centre mass of the rod when rod becomes vertical for the first time.
(d). minimum value of ω, so that centre of rod can complete circular motion.View Solution
Knowledge Check
- Question 1 - Select One
A rod of length L and mass m is kept vertically on the ground. Its potential energy is :
AmgLBmg.L2Cmg.L3Dmg.L4 - Question 2 - Select One
A bicycle chain of length 1.6m and of mass 1kg is lying on a horizontal floor. If g=10ms−2, the work done in lifting it with one end touching the floor and the other end 1.6m above the floor is
A10JB3.2JC8JD16J - Question 3 - Select One
A meter scale of mass 400gm is lying horizontally on the floor. If it is to be held vertically with one end touching the floor, the work to be done is
A6JB4JC40JD2J
A metre scale of mass 200 g, is lying on a horizontal table. What is the work done by student in making it stand vertically on one of its ends?
View SolutionA rod of mass m and length l is lying on a horizontal table. Work done in making it stand on one end will be
View SolutionA uniform cylinder of radius 'r' length 'L' and mass 'm' is lying on the ground with the curved surface touching the ground. F it is to be oriented on the ground with the flat circular end in contact with ground, the work to be done is
View SolutionA long rod ABC of mass "m" and length "L" has two particles of masses "m" and "2m" attached to it as shown in the figure. The system is initially in the horizontal position. The work to be done to keep it vertical with A hinged at the bottom is
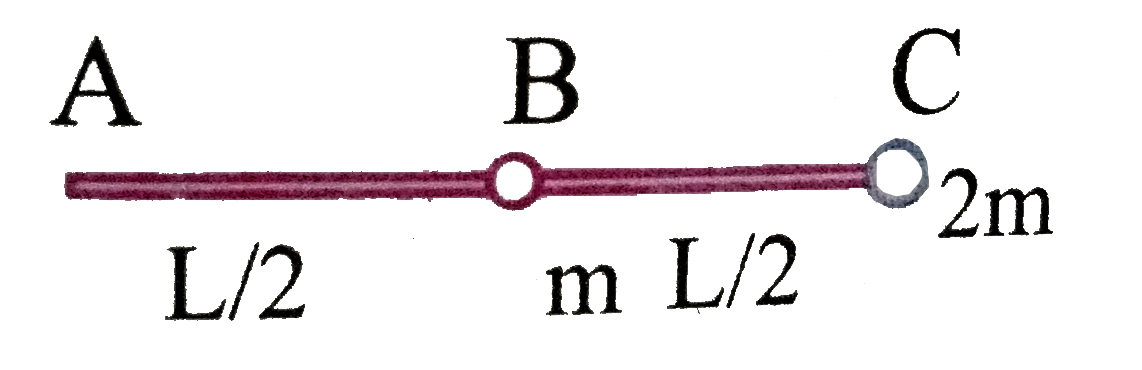 .View Solution
.View SolutionA thin uniform rod of length l and mass m is freely pivoted about its end. The rod is initially held horizontally and released from rest. When the rod is vertical, an impulse J is applied to bring it to rest (this is in addition to any impulse provided by the pivot)

What is the angular velocity of the rod at the moment it reaches the vertical position ?View Solution

