Two bodies of masses 2 kg and 4 kg are moving with velocities 2 m/s and 10 m/s respectively along same direction. Then the velocity of their centre of mass will be
To find the velocity of the center of mass of two bodies, we can use the formula for the velocity of the center of mass (V_cm):
Vcm=m1v1+m2v2m1+m2
Where:
- m1 and m2 are the masses of the two bodies,
- v1 and v2 are their respective velocities.
1. Identify the masses and velocities:
- Mass of the first body, m1=2kg
- Velocity of the first body, v1=2m/s
- Mass of the second body, m2=4kg
- Velocity of the second body, v2=10m/s
2. Calculate the total momentum:
Total momentum=m1v1+m2v2
=(2kg×2m/s)+(4kg×10m/s)
=4kg⋅m/s+40kg⋅m/s=44kg⋅m/s
3. Calculate the total mass:
Total mass=m1+m2=2kg+4kg=6kg
4. Calculate the velocity of the center of mass:
Vcm=Total momentumTotal mass=44kg⋅m/s6kg
=446m/s=223m/s≈7.33m/s
Thus, the velocity of the center of mass is approximately 7.33m/s.
- m1 and m2 are the masses of the two bodies,
- v1 and v2 are their respective velocities.
- Mass of the first body, m1=2kg
- Velocity of the first body, v1=2m/s
- Mass of the second body, m2=4kg
- Velocity of the second body, v2=10m/s
Total momentum=m1v1+m2v2
=(2kg×2m/s)+(4kg×10m/s)
=4kg⋅m/s+40kg⋅m/s=44kg⋅m/s
Total mass=m1+m2=2kg+4kg=6kg
Vcm=Total momentumTotal mass=44kg⋅m/s6kg
=446m/s=223m/s≈7.33m/s
Topper's Solved these Questions
Similar Questions
2 bodies of different masses of 2 kg and 4 kg are moving with velocities 20m/s and 10 m//s towards each other due to mutual gravitational attraction. What is the velocity of their centre of mass?
View SolutionTwo particle of mass 2kgand1kg are moving along the same straight line with speeds 2m/sand5m/s respectively. What is the speed of the centre of mass of the system if both the particles are moving (a) in same direction (b) in opposite direction ?
View Solution
Knowledge Check
- Question 1 - Select One
Two spheres of masses 1 kg and 2 kg are moving with velocities of 10 m/s and 5 m/s respectively in the same direction. After the collision the velocity of the lighter sphere decreases by 4 m/s. What is the coefficient of restitution?
A0.1B0.15C0.2D0.25 - Question 2 - Select One
Two bodies of different masses 2kg and 4kg are moving with velocities 2m/s and 10m/s towards each other due to mutual gravitational attraction. Then the velocity of the centre of mass is
A5ms−1B6ms−1C8ms−1Dzero - Question 3 - Select One
Two bodies of masses 1kgand2kg are moving in two perpendicular direction with velocities 1m/sand2m/s as shown in figure. The velocity of the centre of mass (in magnitide) of the system will be :
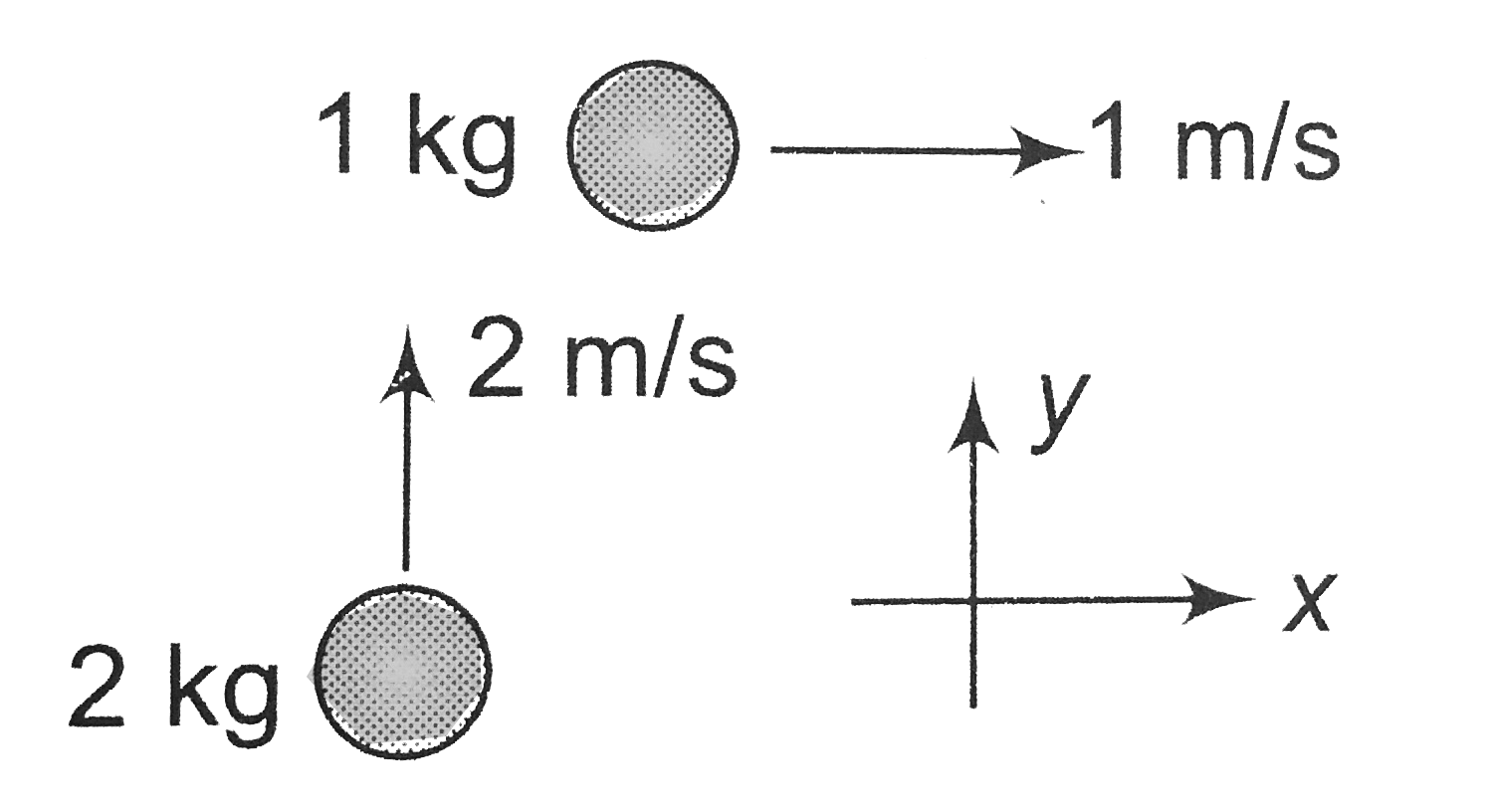 A3m/sB1.67m/sC1.5m/sD1.37m/s
A3m/sB1.67m/sC1.5m/sD1.37m/s
Two bodies of masses 2kg and 4 kg are moving with velocities 20 m/s and 10m/s towards each other due to mutual gravitational attraction . What is the velocity of their centre of mass ?
View SolutionThree bodies having masses 5 kg, 4 kg and 2 kg is moving at the speed of 5 m/sand2m//s respectively along X-axis.The magnitude of velocity of centre of mass is
View SolutionTwo bodies of 6 kg and 4 kg masses have their velocity 5ˆi−2ˆj+10ˆk and 10ˆi−2ˆj+5ˆk, respectively. Then, the velocity of their centre of mass is
View SolutionA body of mass 20 kg is moving with a velocity of 2v and another body of mass 10 kg is moving with velocity V along same direction. The velocity of their centre of mass is
View SolutionTwo blocks of masses 1kg and 3kg are moving with velocities 2m/s and 1m/s , respectively , as shown. If the spring constant is 75N/m , the maximum compression of the spring is
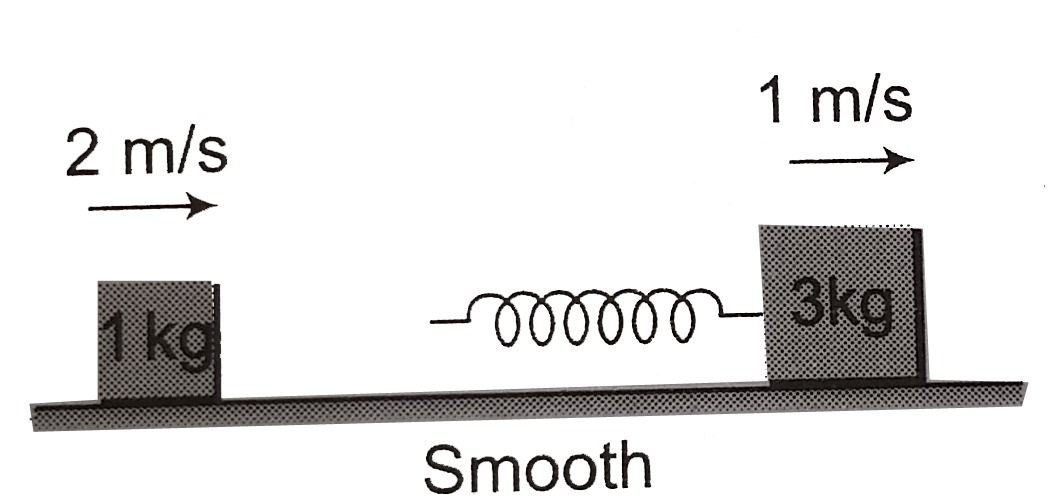 View Solution
View Solution

