Three bodies having masses 5 kg, 4 kg and 2 kg is moving at the speed of 5 m/sand2m//s respectively along X-axis.The magnitude of velocity of centre of mass is
1.0m/s
4m/s
0.9m/s
1.3m/s
The correct Answer is:B
To find the magnitude of the velocity of the center of mass of the three bodies, we can follow these steps:
Step 1: Identify the masses and their velocities
We have three bodies with the following masses and velocities:
- Mass m1=5kg with velocity v1=5m/s
- Mass m2=4kg with velocity v2=2m/s
- Mass m3=2kg with velocity v3=5.5m/s
Step 2: Calculate the total mass
The total mass M of the system is the sum of the individual masses:
M=m1+m2+m3=5kg+4kg+2kg=11kg
Step 3: Calculate the total momentum
The total momentum P of the system is given by the sum of the momenta of each mass:
P=m1v1+m2v2+m3v3
Substituting the values:
P=(5kg×5m/s)+(4kg×2m/s)+(2kg×5.5m/s)
Calculating each term:
P=25kg m/s+8kg m/s+11kg m/s=44kg m/s
Step 4: Calculate the velocity of the center of mass
The velocity of the center of mass Vcm is given by the total momentum divided by the total mass:
Vcm=PM=44kg m/s11kg=4m/s
Final Answer
The magnitude of the velocity of the center of mass is 4m/s.
---
We have three bodies with the following masses and velocities:
- Mass m1=5kg with velocity v1=5m/s
- Mass m2=4kg with velocity v2=2m/s
- Mass m3=2kg with velocity v3=5.5m/s
The total mass M of the system is the sum of the individual masses:
M=m1+m2+m3=5kg+4kg+2kg=11kg
The total momentum P of the system is given by the sum of the momenta of each mass:
P=m1v1+m2v2+m3v3
Substituting the values:
P=(5kg×5m/s)+(4kg×2m/s)+(2kg×5.5m/s)
Calculating each term:
P=25kg m/s+8kg m/s+11kg m/s=44kg m/s
The velocity of the center of mass Vcm is given by the total momentum divided by the total mass:
Vcm=PM=44kg m/s11kg=4m/s
The magnitude of the velocity of the center of mass is 4m/s.
Topper's Solved these Questions
- SOLVD PAPERS 2017 NEET, AIIMS & JIPMERBOOK - DC PANDEYCHAPTER - SOLVD PAPERS 2017 NEET, AIIMS & JIPMEREXERCISE - Solved paper 2018(JIPMER)
38 Videos
- SOLVD PAPERS 2017 NEET, AIIMS & JIPMERBOOK - DC PANDEYCHAPTER - SOLVD PAPERS 2017 NEET, AIIMS & JIPMEREXERCISE - Solved paper 2018(NEET)
22 Videos
- SIMPLE HARMONIC MOTIONBOOK - DC PANDEYCHAPTER - SIMPLE HARMONIC MOTIONEXERCISE - Integer type questions
14 Videos
- SOUND WAVESBOOK - DC PANDEYCHAPTER - SOUND WAVESEXERCISE - Exercise 19.7
4 Videos
Similar Questions
Two bodies of masses 2 kg and 4 kg are moving with velocities 2 m/s and 10 m/s respectively along same direction. Then the velocity of their centre of mass will be
View SolutionTwo particle of mass 2kgand1kg are moving along the same straight line with speeds 2m/sand5m/s respectively. What is the speed of the centre of mass of the system if both the particles are moving (a) in same direction (b) in opposite direction ?
View Solution
Knowledge Check
Two particles of mass 1 kg and 0.5 kg are moving in the same direction with speed of 2m/s and 6 m/s respectively on a smooth horizontal surface. The speed of centre of mass of the system is
A103m/sB107m/sC112m/sD123m/sTwo bodies of masses 1kgand2kg are moving in two perpendicular direction with velocities 1m/sand2m/s as shown in figure. The velocity of the centre of mass (in magnitide) of the system will be :
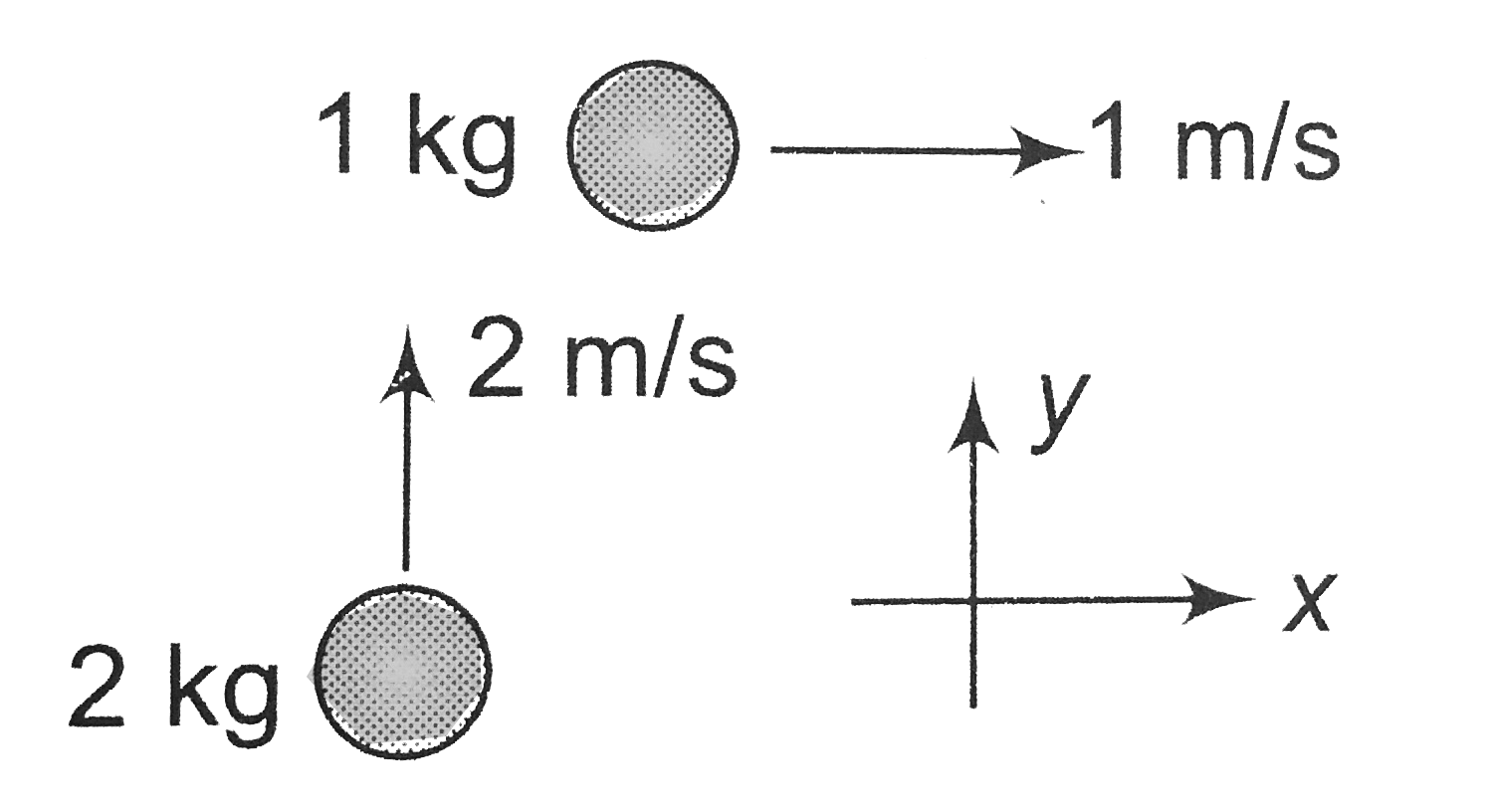 A3m/sB1.67m/sC1.5m/sD1.37m/s
A3m/sB1.67m/sC1.5m/sD1.37m/sThe centres of three spherical masses of 1 kg, 2 kg and 3 kg have co-orinates (4,0) m,(0,3)m and (-2,5)m respectively. What is the position vector of its centre of mass is terms of its x and y co-ordinates?
A→Rcm=2ˆi+3ˆjB→RcM=13ˆi+52ˆjCveRcm=≡−13ˆi+3.5ˆjD→rcm=23ˆi−53ˆj
Two particles of mass 1kg and 2kg are moving along the same line with speeds 2m/s and 4m/s respectively. Calculate the speed of the centre of mass of the system if both the particles are moving in the same direction.
View SolutionA shell at rest at origin explodes into three fragments of masses 1 kg, 2 kg and m kg. The fragments of masses 1 kg and 2 kg fly off with speeds 12m/s along x-axis and 8m/s along y- axis. If the m kg piece flies off with speed of 40m/s then find the total mass of the shell.
View SolutionTwo spheres are moving towards each other. Both have same radius but their masses are 2kg and 4kg. If the velocities are 4m/s and 2m/s respectively and coefficient of restitution is e=1/3, Find final velocities along line of impact.
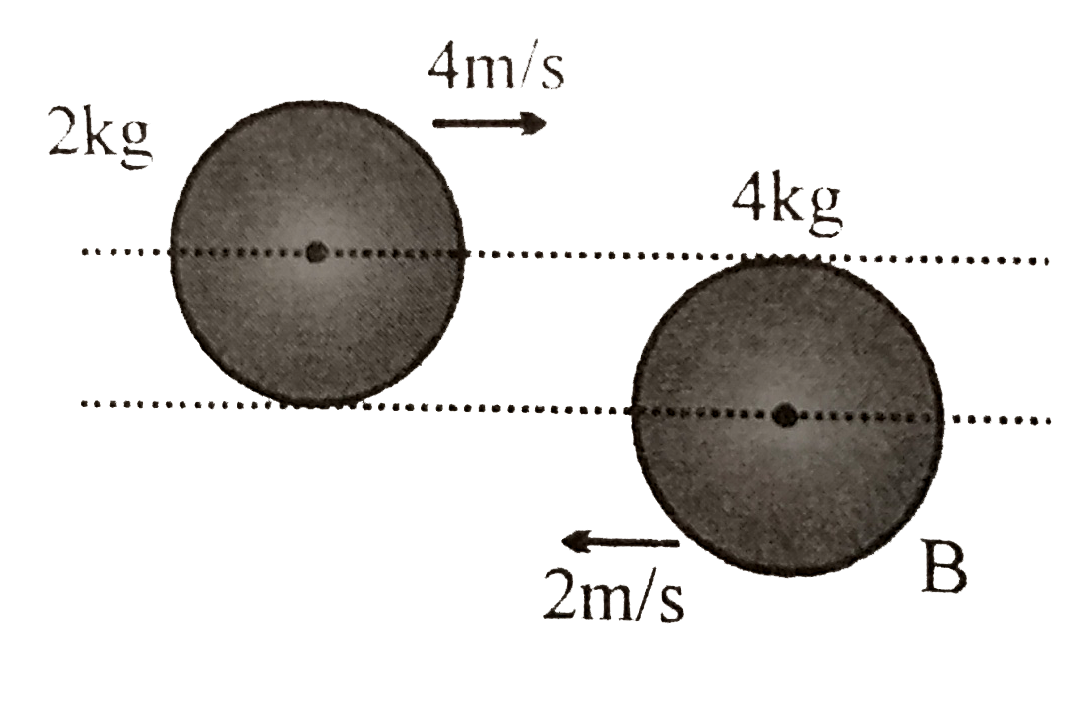
View SolutionTwo bodies of different masses 2kg and 4kg are moving with velocities 2m/s and 10m/s towards each other due to mutual gravitational attraction. Then the velocity of the centre of mass is
View SolutionTwo bodies of mass 2 kg and 5 kg have position (1 m, 2 m, 1 m) and (3 m, 2 m, -1 m) respectively. The position vector of centre of mass is
View Solution