The position of a particle moving along the x-axis at certain times is given below ∣∣∣t(s)0123x(m)−20616∣∣∣ Which of the following describes the motion correctly?
(a) Uniform, accelerated
(b) Uniform, decelerated
(c) Non-uniform, accelerated
(d) There is not enough data for generalization
To analyze the motion of the particle moving along the x-axis, we will follow these steps:
Step 1: Gather the Data
We have the following data points for time (t in seconds) and position (x in meters):
- At t = 0 s, x = -2 m
- At t = 1 s, x = 0 m
- At t = 2 s, x = 6 m
- At t = 3 s, x = 16 m
Step 2: Plot the Points
We can plot these points on a graph where the x-axis represents time (t) and the y-axis represents position (x):
- Point 1: (0, -2)
- Point 2: (1, 0)
- Point 3: (2, 6)
- Point 4: (3, 16)
Step 3: Calculate the Velocity
The velocity (v) between two points can be calculated using the formula:
v=ΔxΔt
1. Between t = 0 s and t = 1 s:
v1=0−(−2)1−0=21=2m/s
2. Between t = 1 s and t = 2 s:
v2=6−02−1=61=6m/s
3. Between t = 2 s and t = 3 s:
v3=16−63−2=101=10m/s
Step 4: Analyze the Velocity
From the calculated velocities:
- v_1 = 2 m/s
- v_2 = 6 m/s
- v_3 = 10 m/s
The velocity is increasing with time, indicating that the particle is accelerating.
Step 5: Calculate the Acceleration
The acceleration (a) can be calculated using the change in velocity over time:
a=ΔvΔt
1. Between t = 0 s and t = 1 s:
- Not applicable since we need two velocities.
2. Between t = 1 s and t = 2 s:
a1=6−22−1=41=4m/s2
3. Between t = 2 s and t = 3 s:
a2=10−63−2=41=4m/s2
Step 6: Conclusion
The acceleration is constant at 4 m/s² between the intervals calculated. Since the velocity is increasing and the acceleration is constant, the motion of the particle can be described as uniformly accelerated motion.
We have the following data points for time (t in seconds) and position (x in meters):
- At t = 0 s, x = -2 m
- At t = 1 s, x = 0 m
- At t = 2 s, x = 6 m
- At t = 3 s, x = 16 m
We can plot these points on a graph where the x-axis represents time (t) and the y-axis represents position (x):
- Point 1: (0, -2)
- Point 2: (1, 0)
- Point 3: (2, 6)
- Point 4: (3, 16)
The velocity (v) between two points can be calculated using the formula:
v=ΔxΔt
v1=0−(−2)1−0=21=2m/s
v2=6−02−1=61=6m/s
v3=16−63−2=101=10m/s
From the calculated velocities:
- v_1 = 2 m/s
- v_2 = 6 m/s
- v_3 = 10 m/s
The acceleration (a) can be calculated using the change in velocity over time:
a=ΔvΔt
- Not applicable since we need two velocities.
a1=6−22−1=41=4m/s2
a2=10−63−2=41=4m/s2
The acceleration is constant at 4 m/s² between the intervals calculated. Since the velocity is increasing and the acceleration is constant, the motion of the particle can be described as uniformly accelerated motion.
Topper's Solved these Questions
Similar Questions
The position of a particle along x-axis at certain time is given below
Determine the following
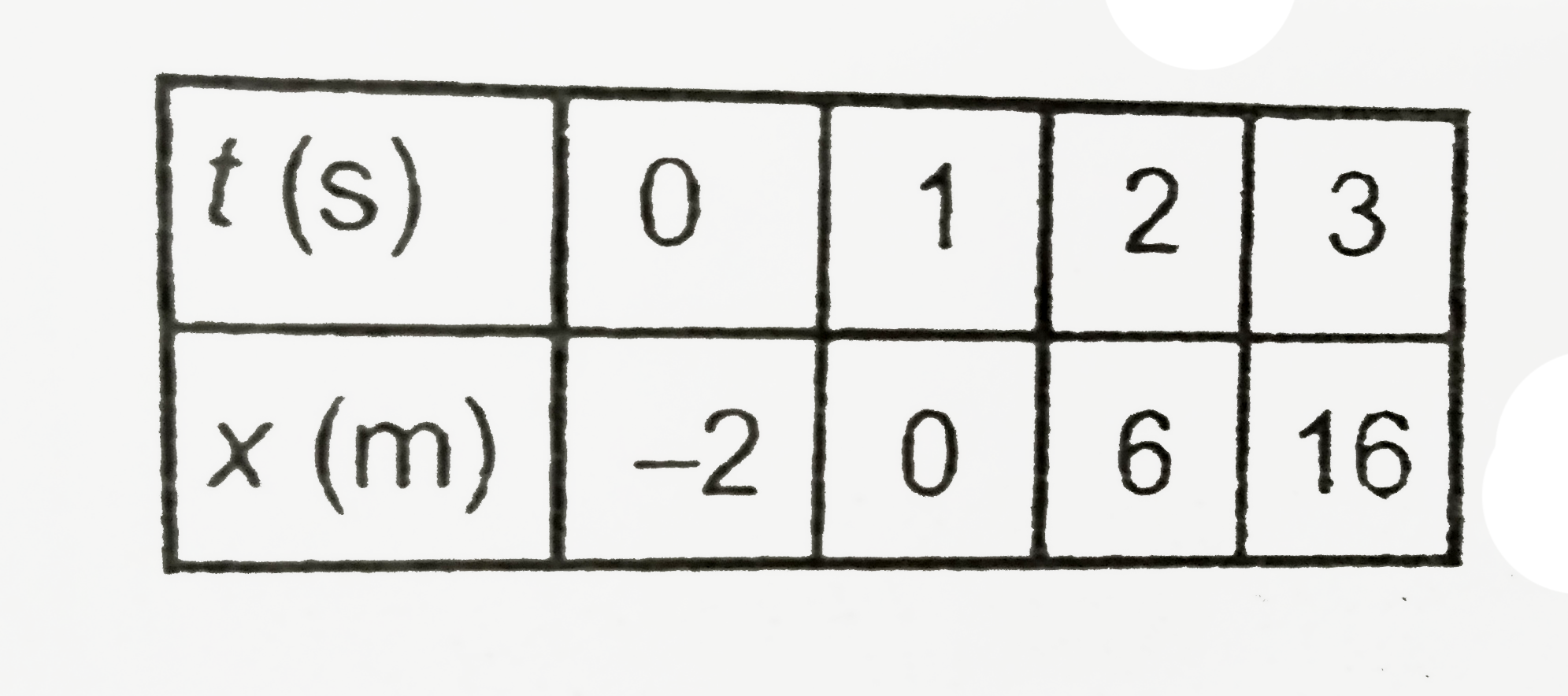
(a) Average velocity of particle in first 3 seconds.
(b) Position of particle at the end of 4th second if particle continue the trend.
(c) Acceleration of particle at time t = 3 s
(d) Type of motionView SolutionThe position (x) of a particle of mass 1 kg moving along X-axis at time t is given by (x=12t2) metre. Find the work done by force acting on it in time interval from t=0 to t=3s.
View Solution
Knowledge Check
- Question 1 - Select One
The position of a particle moving along the x-axis at certain times is given below:
t(s)0123x(m)−20616
Which of the following describes the motion correctly?AUniform, acceleratedBUniform, deceleratedCNon-uniform, acceleratedDThere is not enough data for generalization - Question 2 - Select One or More
Position of particle moving along x-axis is given as x=2+5t+7t2 then calculate :
AVelocity (i.edxdt) of particleBInitial velocity(i.edxdt att=0)CVelocity at t=2secDAccleration(i.ed2xdt2) of particle - Question 3 - Select One
Position of a particle moving along x-axis is given by x=6t−t2+4, where x is in metre and t is in second. Which of the following is correct?
AFor t = 3, particle is moving along positive x-axisBInitial velocity is 4 m/sCInitial acceleration is 4m/s2DInitial acceleration is 6m/s2
The position of particle moving along the x-axis veries with time t as x=6t−t2+4. Find the time-interval during which the particle is moving along the positive x-direction.
View SolutionThe position of a particle moving along x-axis varies eith time t as x=4t−t2+1. Find the time interval(s) during which the particle is moving along positive x-direction.
View SolutionThe position (x) of a particle of mass 1 kg moving along X-axis at time t is given by (x=12t2) metre. Find the work done by force acting on it in time interval from t=0 to t=3s.
View SolutionThe displacement x of a particle moving along x-axis at time t is given by x2=2t2+6t. The velocity at any time t is
View SolutionThe position of a particle moving along x- axis is given by x=x0cos2(ωt) . Its when it is at mean position is
View Solution

