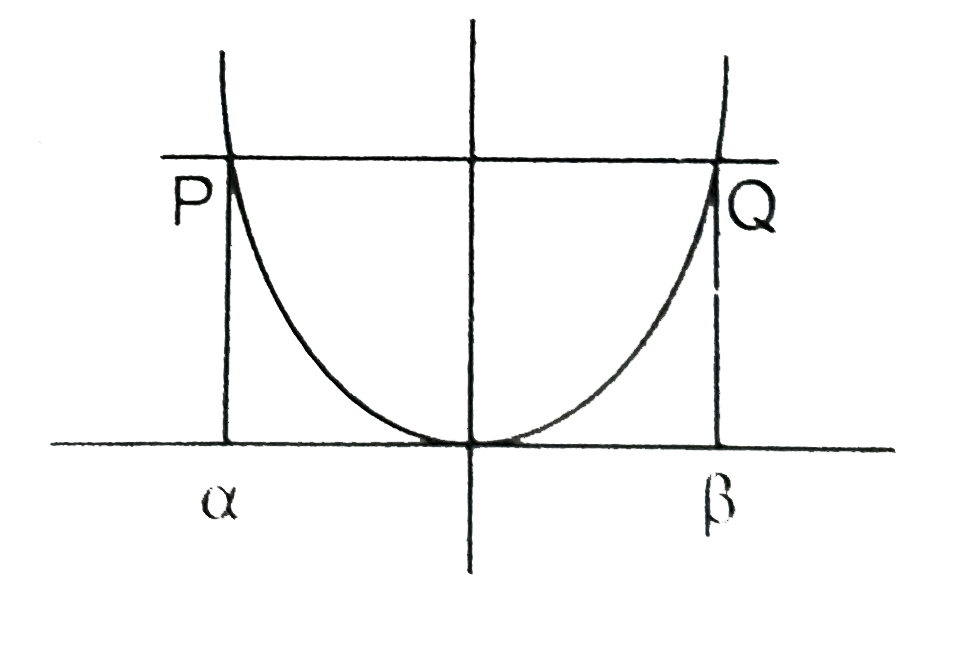
A variable line $L$ intersects the parabola $y = x^{2}$ at points $P$ and $Q$ whose $x$ - coordinate are $α$ and $β$ respectively with $α < β$ the area of the figure enclosed by the segment $P Q$ and the parabola is always equal to $\frac{4}{3}$ . The variable segment $P Q$ has its middle point as $M$
Which of the following is/are correct?

equations of the pair of tangents, drawn to the curve, represented by locus of $M$ from origin are $y = 2 x$ and $y = - 2 x$

equation of pair of tangents to be curve, represented by locus of $M$ from origin are $y = x$ and $y = - x$

area of the region enclosed between the curve, represented by locus of $M$ , and the pair of tangents drawn to it from origin is $\frac{2}{3}$ sq. units

area of the region enclosed beween the curve, represented by locus of $M$ , and the pair of tangents drawn of it, from origin is $\frac{1}{3}$ sq. units

Text Solution

Verified by Experts

The correct Answer is:C

Any two point on $y = x^{2}$ is $P (α, α^{2}), Q (β, β^{2})$
Equation of $P Q, y - α^{2} = (α + β) (x - α)$
$y = (α + β) x - α β$
Required area $\int_{α}^{β} ((α + β) x - α β - x^{2}) d x$
$\Rightarrow β - α = 2$
Pair of tangents from origin are $y = 2 x$ and $y = - 2 x$
Area $\int_{0}^{1} ((x^{2} +) - 2 x) d x = \frac{2}{3}$

Updated on:21/07/2023

Class 12 MATHS QUESTION BANK

Topper's Solved these Questions

Knowledge Check

The straight lines $y = \pm x$ intersect the parabola $y^{2} = 8 x$ in points P and Q, then length of PQ is
A4
B $4 \sqrt{2}$
C $8$
D $16$
The straight lines $y = \pm x$ intersect the parabola $y^{2} = 8 x$ in points P and Q, then length of PQ is
A4
B $4 \sqrt{2}$
C8
D16
A variable tangent to the parabola $y^{2} = 4 a x$ meets the parabola $y^{2} = - 4 a x$ P and Q. The locus of the mid-point of PQ, is
A $y^{2} = - 2 a x$
B $y^{2} = - a x$
C $y^{2} = - \frac{4}{3} a x$
D $y^{2} = - 4 a x$

FIITJEE-TEST PAPERS-MATHEMATICS

Home
Ask Doubt
Profile

The correct Answer is:C

Any two point on y=x2 is P(α,α2),Q(β,β2) Equation of PQ,y−α2=(α+β)(x−α) y=(α+β)x−αβ Required area ∫βα((α+β)x−αβ−x2)dx ⇒β−α=2 Pair of tangents from origin are y=2x and y=−2x Area ∫10((x2+)−2x)dx=23

Topper's Solved these Questions

Similar Questions

Knowledge Check

The straight lines y=±x intersect the parabola y2=8x in points P and Q, then length of PQ is

The straight lines y=±x intersect the parabola y2=8x in points P and Q, then length of PQ is

A variable tangent to the parabola y2=4ax meets the parabola y2=−4ax P and Q. The locus of the mid-point of PQ, is

FIITJEE-TEST PAPERS-MATHEMATICS

The straight lines $y = \pm x$ intersect the parabola $y^{2} = 8 x$ in points P and Q, then length of PQ is

The straight lines $y = \pm x$ intersect the parabola $y^{2} = 8 x$ in points P and Q, then length of PQ is

A variable tangent to the parabola $y^{2} = 4 a x$ meets the parabola $y^{2} = - 4 a x$ P and Q. The locus of the mid-point of PQ, is