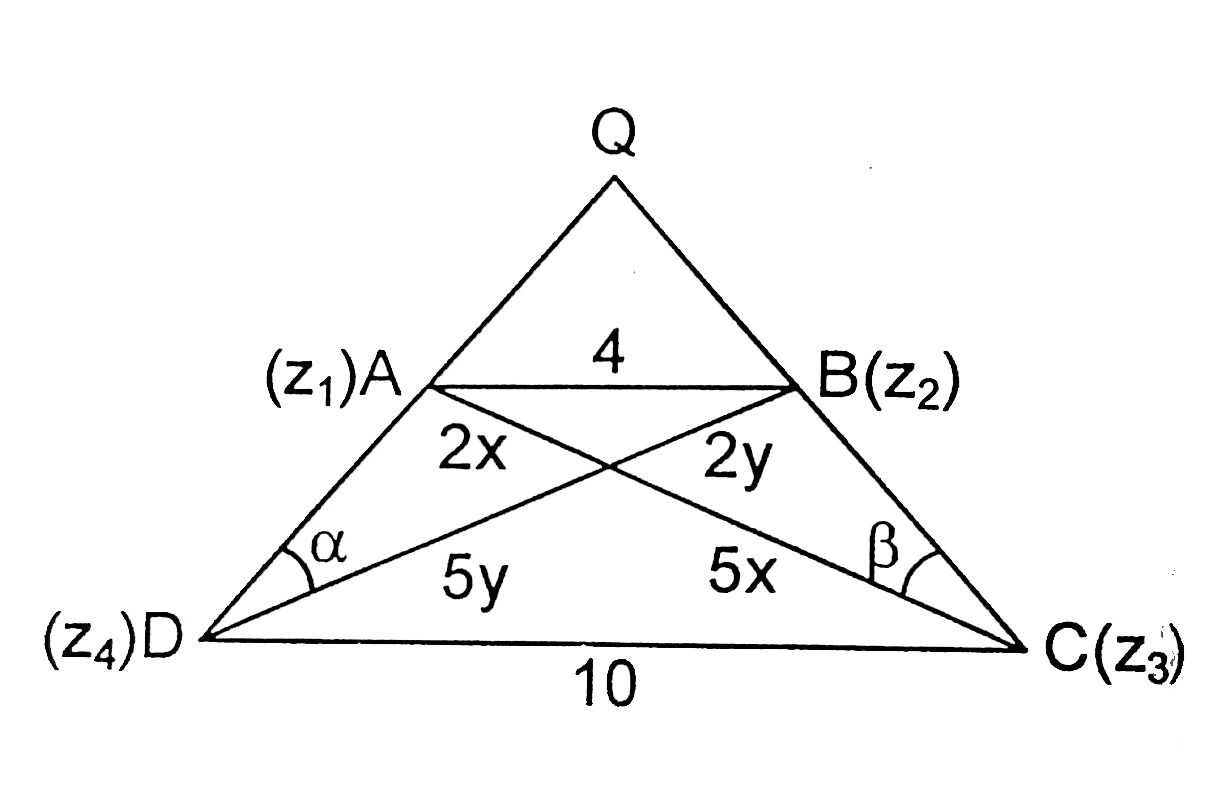
Let A(z1),B(z2),C(z3 and D(z4) be the vertices of a trepezium in an Argand plane such that AB∣∣CD Let |z1−z2|=4,|z3,z4|=10 and the diagonals AC and BD intersects at P. It is given that Arg(z4−z2z3−z1)=π2 and Arg(z3−z2z4−z1)=π4
Which of the following option(s) is/are correct?
Area of trepezium ABCD is equla to 1403 sq. units
Area of the trapezium ABCD is equal to 703 sq. units
Area of the triangle BCP is equal, to 10021 sq. units
Area of the triangle BCP is equal to 20042 sq. units
The correct Answer is:A
ΔABP and ΔCDP are similar if AP=2x
BP=2y then CP=5x,DP=5y
Area of trapezium ABCD=492xy
tanα=2x5y,tanβ=2y2x also α+β=45∘
⇒xy=1021(x2+y2)
Also AB2=AP2+BP2⇒x2+y2=4⇒xy=4021
Ar(ΔPCD)=5xy
Topper's Solved these Questions
Similar Questions
Let A(z1),B(z2),C(z3 and D(z4) be the vertices of a trepezium in an Argand plane such that AB∣∣CD Let |z1−z2|=4,|z3,z4|=10 and the diagonals AC and BD intersects at P. It is given that Arg(z4−z2z3−z1)=π2 and Arg(z3−z2z4−z1)=π4
Which of the following option(s) is/are incorrect?
View SolutionLet A(z1),B(z2),C(z3) be the vertices of an equilateral triangle ABC in the Argand plane, then the number z2−z32z1−z2−z3, is
View Solution
Knowledge Check
Let A(z1),B(z2),C(z3 and D(z4) be the vertices of a trepezium in an Argand plane such that AB∣∣CD Let |z1−z2|=4,|z3,z4|=10 and the diagonals AC and BD intersects at P. It is given that Arg(z4−z2z3−z1)=π2 and Arg(z3−z2z4−z1)=π4
Which of the following option(s) is/are incorrect?AValue of |CP−DP| is equal to 10√21BPB:PD=2:5CPC:AC=5:7DPC:AC=2:3Let A(z1),B(z2),C(z3) be the vertices of an equilateral triangle ABC in the Argand plane, then the number z2−z32z1−z2−z3, is
Apurely realBpurely imaginaryCa complex number with non-zero and imaginary partsDnone of theseIf z1,z2andz3 are the vertices of a triangle in the argand plane such that |z1−z2|=|z1−z3|, then ∣∣∣arg(2z1−z2−z3z3−z2)∣∣∣ is
Aπ3B0Cπ2Dπ6
If z1,z2andz3 are the vertices of a triangle in the argand plane such that |z1−z2|=|z1−z3|, then ∣∣∣arg(2z1−z2−z3z3−z2)∣∣∣ is
View SolutionIf A(z1),B(z2),C(z3) are the vertices of an equilateral triangle ABC, then arg 2z1−z2−z3z3_z2=
View SolutionIf z1,z2,z3,z4 are the affixes of the vertices of a parallelogram taken in order in Argand plane, then
View Solutionz1,z2,z3andz4 are the affixes of four points in the argand plane and z affix of a point such that |z−z1|=|z−z2|=|z−z3| then =|z−z4|, z1,z2,z3andz4 are
View SolutionIf A(z1),B(z2),C(z3) are the vertices of an equilateral triangle ABC, then arg 2z1−z2−z3z3_z2=
View Solution
