Consider an ellipse 4x2=52−13y2 and a variable point P on the line y+2x=12 such that angle F1PF2 is maximum where F1 and F2 are the foci of the given ellipse then (F2PF1P)2 is equal to (where F1 lies on positives x-axis)
The correct Answer is:C
∠F1PF2 is maximum if P is point of tangency of circle through F1,F2&PF2ony+2x=12
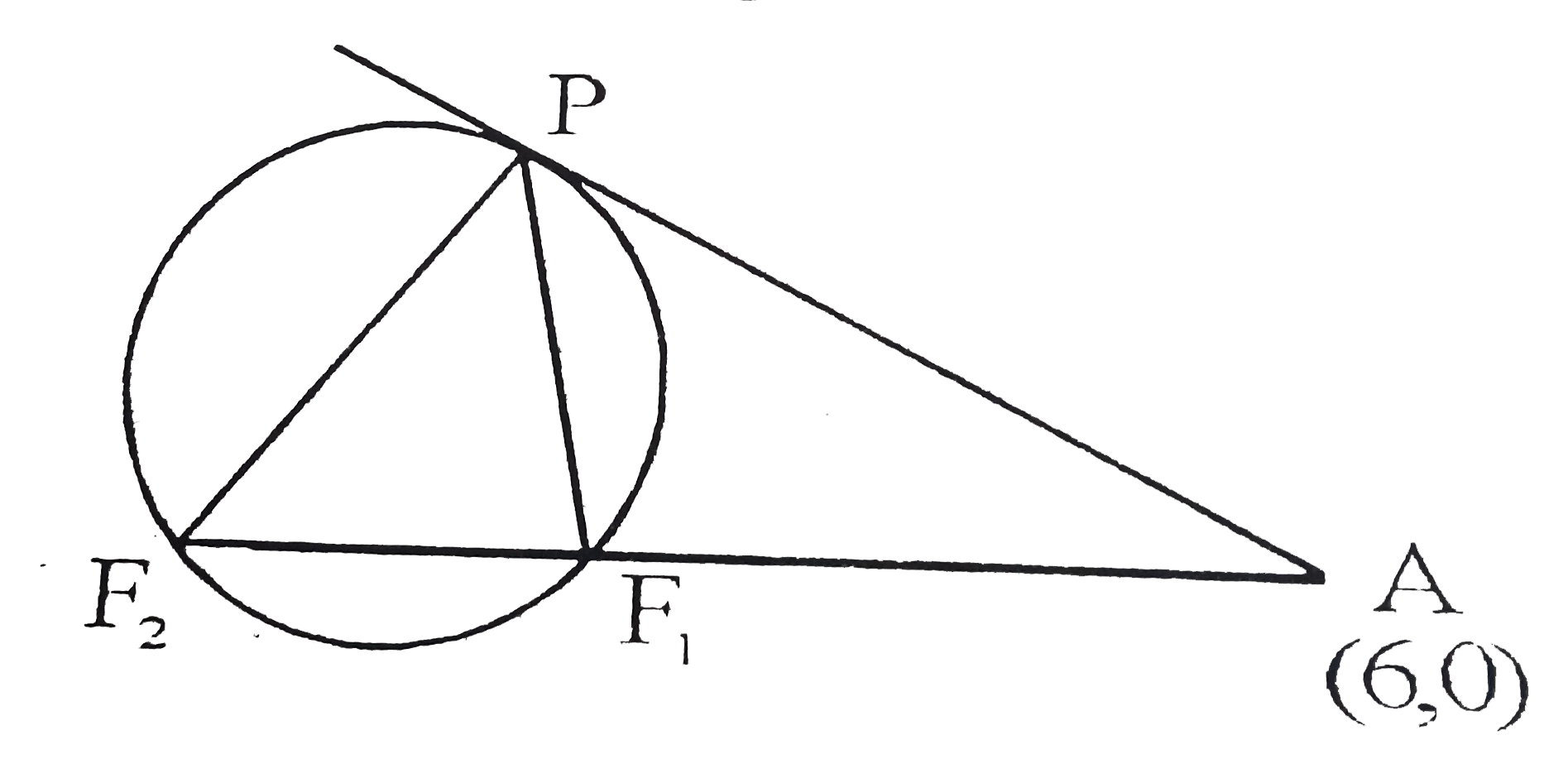
∠APF1=∠AF2ΠmpliesΔAPF1~ΔAF2P
⇒F1PF2P=APAF2&AP2=AF1AF2
(PF2PF1)2=AF2AF1=3
Topper's Solved these Questions
- TEST PAPERSBOOK - ALLENCHAPTER - TEST PAPERSEXERCISE - part-2 Mathematics
48 Videos
- TEST PAPERSBOOK - ALLENCHAPTER - TEST PAPERSEXERCISE - MATHEMATICS
51 Videos
- TEST PAPERSBOOK - ALLENCHAPTER - TEST PAPERSEXERCISE - part-2 Mathematics
18 Videos
- TEST PAPERBOOK - ALLENCHAPTER - TEST PAPEREXERCISE - CHEMISTRY SECTION-II
8 Videos
- VECTOR ALGEBRABOOK - ALLENCHAPTER - VECTOR ALGEBRAEXERCISE - All Questions
1 Videos
Similar Questions
F1andF2 are the two foci of the ellipse x29+y24=1. Let P be a point on the ellipse such that |PF1|=2|PF2|, where F1andF2 are the two foci of the ellipse . The area of △PF1F2 is :
View SolutionF1andF2 are the two foci of the ellipse x29+y24=1. Let P be a point on the ellipse such that |PF1|=2|PF2|, where F1andF2 are the two foci of the ellipse . The area of △PF1F2 is :
View Solution
Knowledge Check
Consider an ellipse x213+y24=1 and a variaote point P(1-,2t) is takne in such a way that ∠S1PS2 is maximum where S1andS2 are the foci of the given ellipse then the value of S1PS2P is
A√2√3B1√5C1√3D1√2F1andF2 are the two foci of the ellipse x29+y24=1. Let P be a point on the ellipse such that |PF1|=2|PF2|, where F1andF2 are the two foci of the ellipse . The area of △PF1F2 is :
A3B4C√5D√132P is any variable point on the ellipse 4x2+9y2=36 and F1,F2 are its foci. Maxium area of △PF1F2 ( e is eccentricity of ellipse )
A9eB4eC6eD10e
P is any variable point on the ellipse 4x2+9y2=36 and F1,F2 are its foci. Maxium area of △PF1F2 ( e is eccentricity of ellipse )
View SolutionLet F1,F2 are the foci of the ellipse 4x2+9y2=36 and P is a point on ellipse such that PF1=2PF2, then the area of triangle PF1F2 is
View SolutionIf P is any point on the ellipse with eccentricity 23 and foci F1,F2 such that PF1+PF2=6 ,then the equation of the ellipse
View SolutionLet P be a point on the ellipse x2100+y225=1 and the length of perpendicular from centre of the ellipse to the tangent to ellipse at P be 5√2andF1andF2 be the foci of the ellipse, then PF1.PF2.
View SolutionIf the circle whose diameter is the major axis of the ellipse x2a2+y2b2=1(a>b>0) meets the minor axis at point P and the orthocentre of ΔPF1F2 lies on the ellipse, where F1andF2 are foci of the ellipse, then the square of the eccentricity of the ellipse is
View Solution
