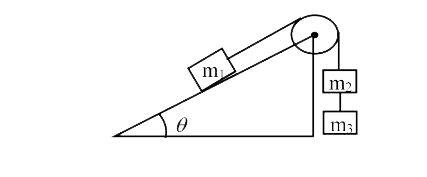
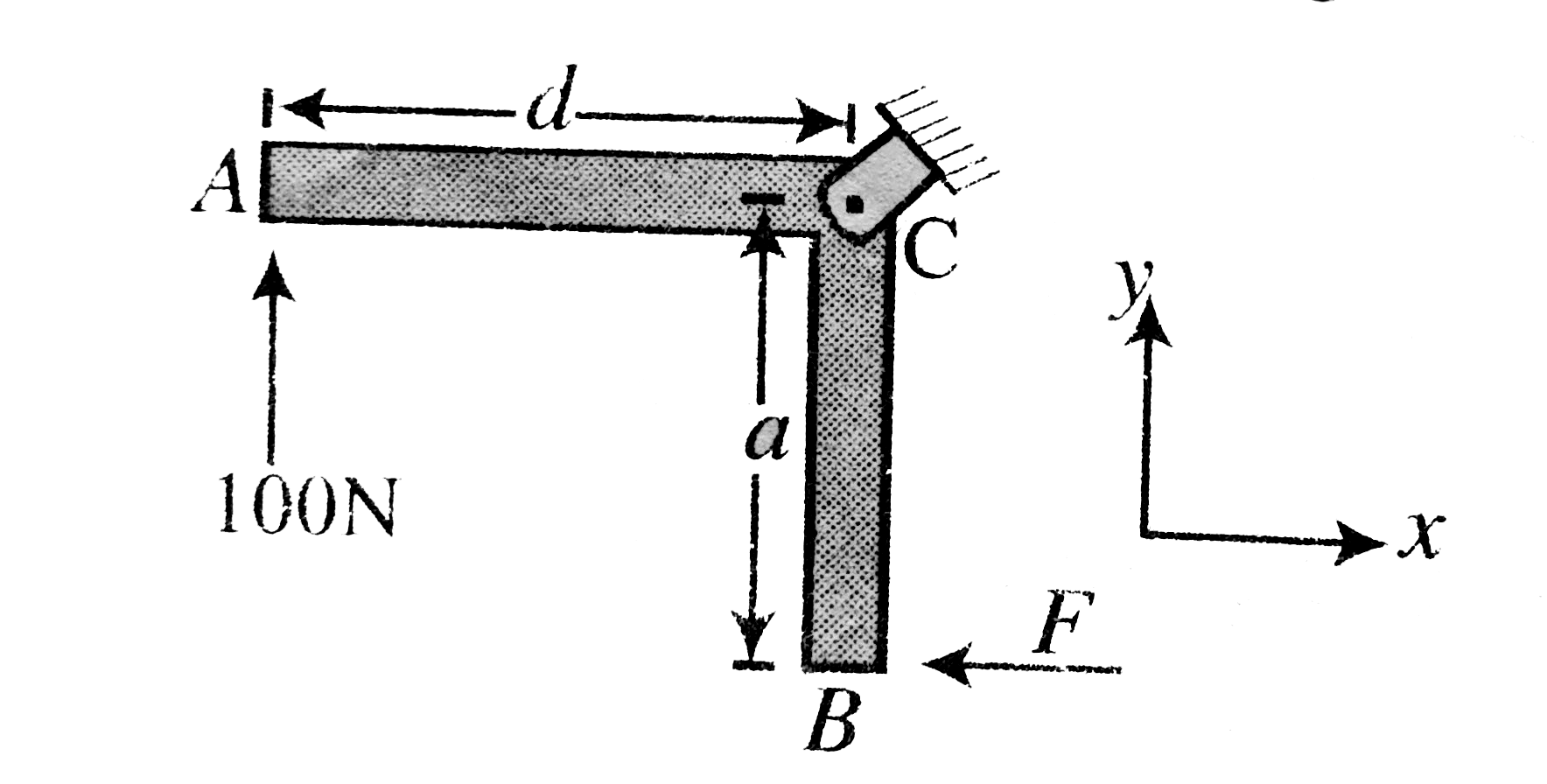
Find force underset('F')(rarr) at 'B' required to keep the system in equilibrium. The dimension of the system are d=0.3m and a=0.2m. Assuming the rods to be massless

150ˆi
150(−ˆk)
150(−ˆi)
It cannot be in equilibrium
The correct Answer is:A
ˆτc=−dˆi×100ˆj+(−aˆj)×(+Fˆi)=0
−100dˆk+aFˆk=0
⇒100d=aF⇒F=100×0.30.2=150
Topper's Solved these Questions
- TEST PAPERSBOOK - ALLENCHAPTER - TEST PAPERSEXERCISE - PHYSICS
18 Videos
- TEST PAPERSBOOK - ALLENCHAPTER - TEST PAPERSEXERCISE - MATHS
18 Videos
- TEST PAPERSBOOK - ALLENCHAPTER - TEST PAPERSEXERCISE - part-2 physics
63 Videos
- TEST PAPER 4BOOK - ALLENCHAPTER - TEST PAPER 4EXERCISE - PHYSICS
45 Videos
- UNIT & DIMENSIONS, BASIC MATHS AND VECTOR BOOK - ALLENCHAPTER - UNIT & DIMENSIONS, BASIC MATHS AND VECTOR EXERCISE - Exercise (J-A)
7 Videos
Similar Questions
Knowledge Check
Find force F required to keep the system in equilibrium. The dimensions of the system are d=0.3m and a=0.2m. Assume the rods to be massless.
 A150(ˆi)B150(−ˆk)C150(−ˆi)Dit cannot be in equilibrium
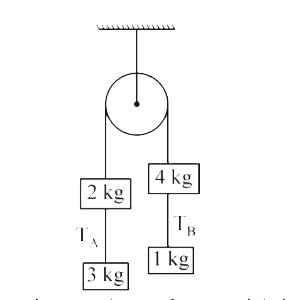
A150(ˆi)B150(−ˆk)C150(−ˆi)Dit cannot be in equilibriumIf system is in equilibrium then find relation between m1 and m2
 Am1m2=12Bm1m2=115Cm1m2=110Dm1m2=1
Am1m2=12Bm1m2=115Cm1m2=110Dm1m2=1Two masses M and m are suspended together by massless spring of force constant -k. When the masses are in equilibrium, M is removed without disturbing the system. The amplitude of oscillations.
AMgkBmgkC(M+m)gkD(M−m)gk
The situation shown in the figure, all surfaces are smooth. The mass of sphere is 7 kg if the force F required to keep the system in equilibrium is 9 n N. Then find the value of n.
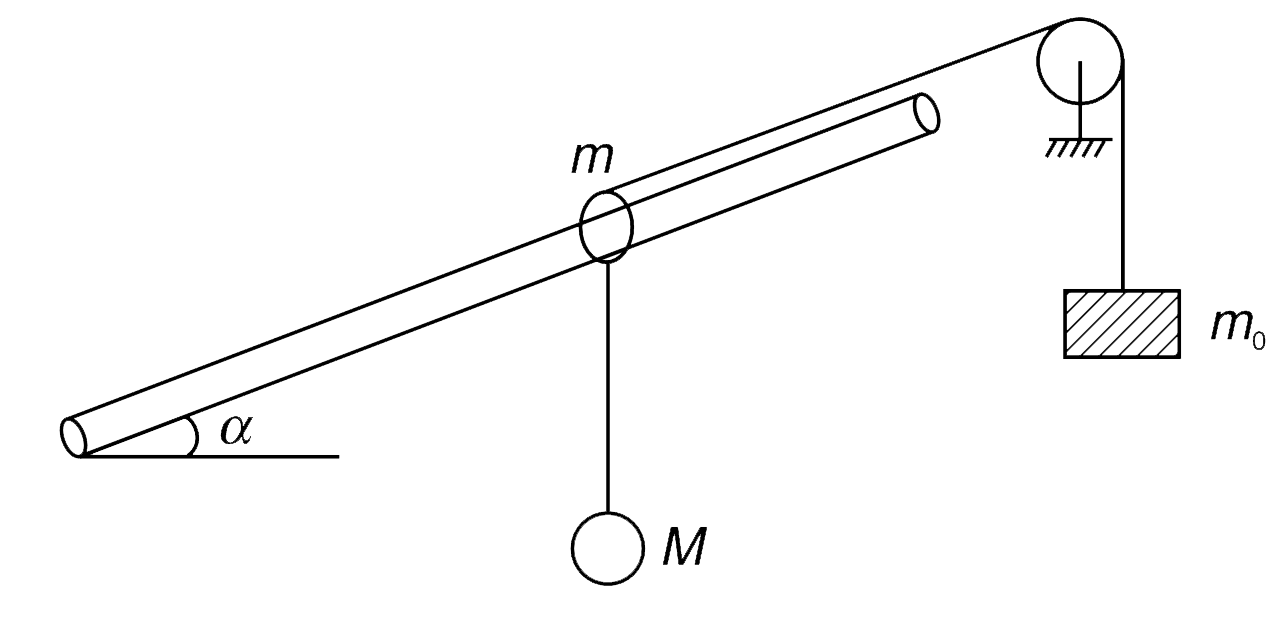
View SolutionA smooth rod is fixed at an angle α to the horizontal. A small ring of mass m can slide along the rod. A thread carrying a small sphere of mass M is attached to the ring. To keep the system in equilibrium, another thread is attached to the ring which carries a load of mass m0at its end (see figure). The thread runs parallel to the rod between the ring and the pulley. All threads and pulley are massless.
(a) Find m0 so that system is in equilibrium.
(b) Find acceleration of the sphere M immediately after the thread supporting m0 is cut.
View SolutionFind the magnitude of the horizontal force F required to keep the block of mass m stationary on the smooth inclined plane as shown in the figure.

View SolutionFind the direction cosine of given system of relation l + m + n = 0, 2 lm + 2 nl - mn = 0.
View SolutionTwo mass m1 and m2 are suspended from a massless spring of force constant k. When the masses are in equilibrium, m1 is removed without disturbing the system. Find the angular frequency and amplitude of oscillations.
View Solution