In the fig. shown, the pulley and string are mass less and the incline is frictionless. The segment AP of the string is parallel to the incline and the segment PB is perpendicular to the incline. End of the string is pulled with a constant force F.
(a) If the block is moving up the incline with acceleration while being in contact with the incline , then angle θ must be less than θ0. Find θ0
If θ=θ02 find the maximum acceleration with which the block can move up the plane without losing contact with the incline.
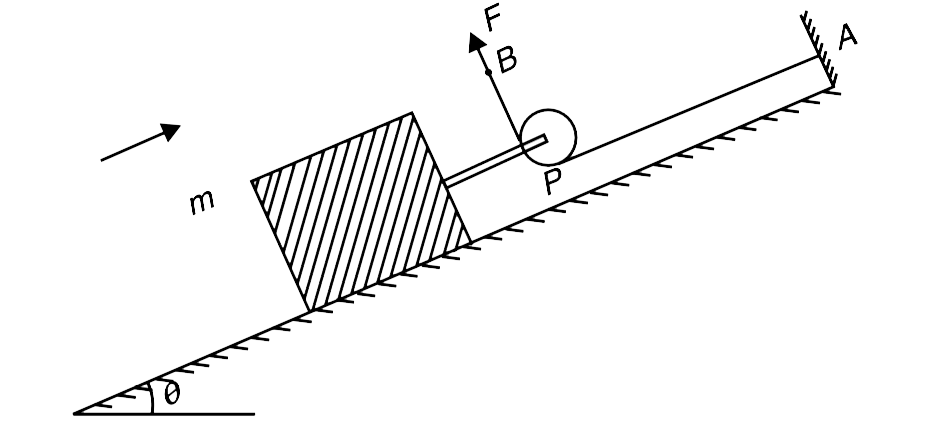
The correct Answer is:(a) θ0=π4
(b) amax=g[cos(π8)−sin(π8)]
Step by step video, text & image solution for In the fig. shown, the pulley and string are mass less and the incline is frictionless. The segment AP of the string is parallel to the incline and the segment PB is perpendicular to the incline. End of the string is pulled with a constant force F. (a) If the block is moving up the incline with acceleration while being in contact with the incline , then angle theta must be less than theta_(0). Find theta_(0) If theta = (theta_(0))/(2) find the maximum acceleration with which the block can move up the plane without losing contact with the incline. by Physics experts to help you in doubts & scoring excellent marks in Class 11 exams.
Updated on:21/07/2023Topper's Solved these Questions
Similar Questions
Knowledge Check
In the shown mass pulley system, pulleys and string are massless. The one end of the string is pulled by the force F = mg. The acceleration of the block will be :
 Ag/2B0CgD3g
Ag/2B0CgD3g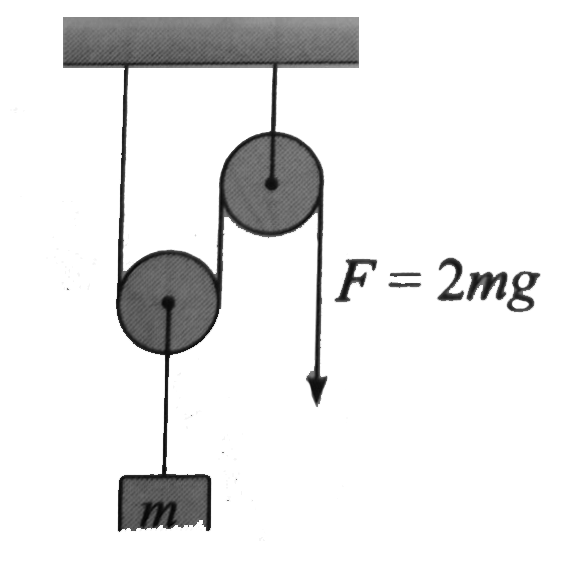
In the shown mass pulley system, pulleys and string are massless. The one end of the string is pulleed by the force F=2mg. The acceleration of the block will beAg2B0CGreater than →vD3gIn fig mass m is lifted up by attaching a mass 2m to the other end of the string while in fig
(b) m is lifted by pulling the other end of the string with a constant force F=2mg then:
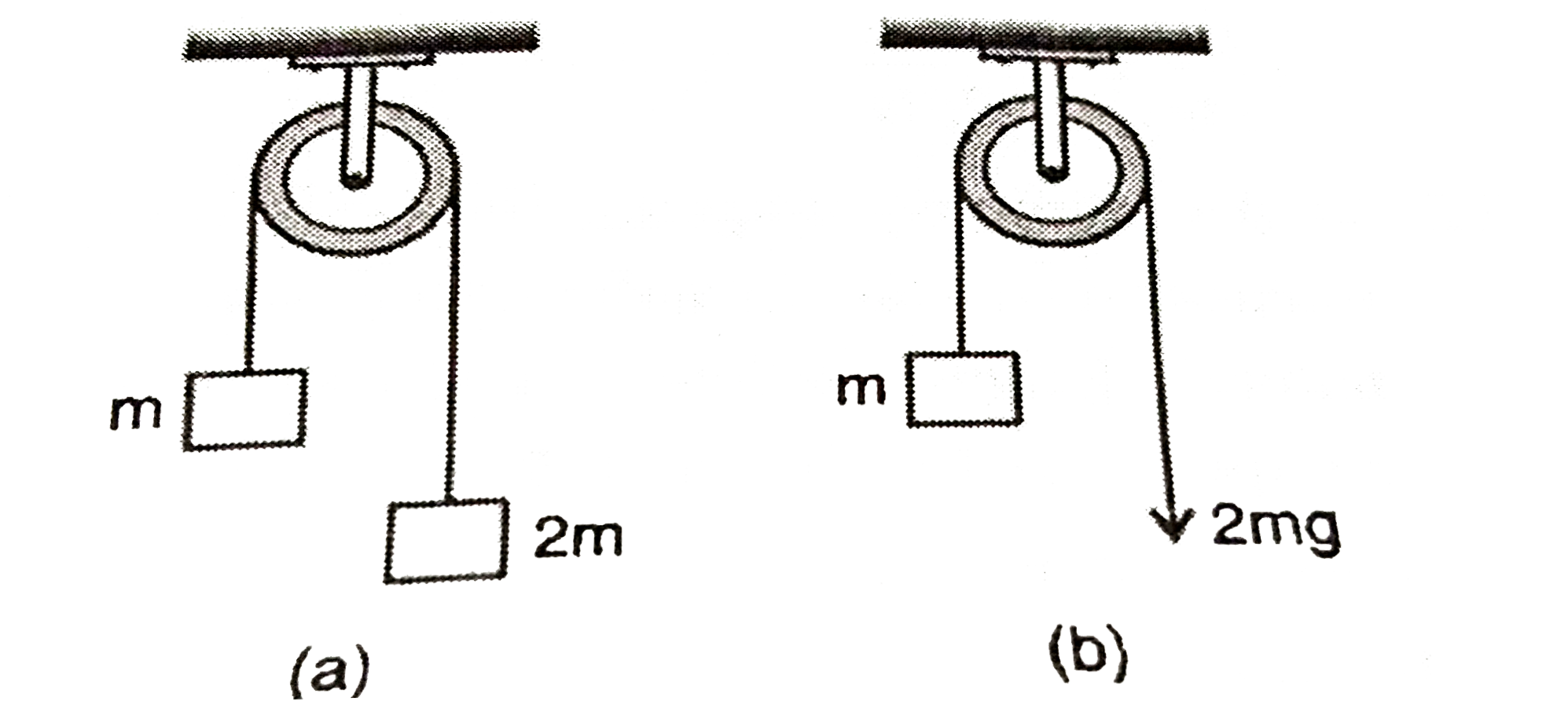 Aacceleration of m in the two situations is same and non - zeroBacceleration of m in situation
Aacceleration of m in the two situations is same and non - zeroBacceleration of m in situation
(a) is more than that of in situation(b)Cacceleration of m in situation
(a) is less than that of in situation
(b)Dacceleration of m in the two situations is same and equals to zero
A body of mass 2 kg is lyign on rough inclined plane of inclination 30 0 . Find the mgnitude of the force parallel to the incline needed to make tkheblock move a. up the incline b. down the incline. Coefficient of static friction =0.2
Repeat part a. of problem 6 if the push is applied horizontally and not parallel to the incline.
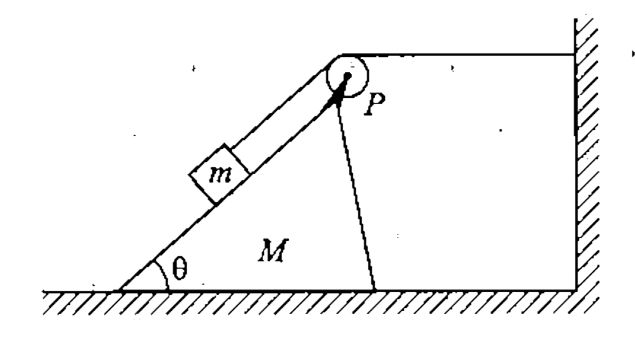
View SolutionIn the figure-2.61 a bar of mass m is on the smooth inclined face of the wedge of mass M,the inclination to the horizontal being 0.The wedge is resting on a smooth horizontal plane.Assuming the pulley to be smooth and the string is light and inextensible. Find the acceleration of M,when M and m are always in contact.
View SolutionA body of mass 2 kg is lyign on rough inclined plane of inclination 300. Find the mgnitude of the force parallel to the incline needed to make tkheblock move a. up the incline b. down the incline. Coefficient of static friction =0.2
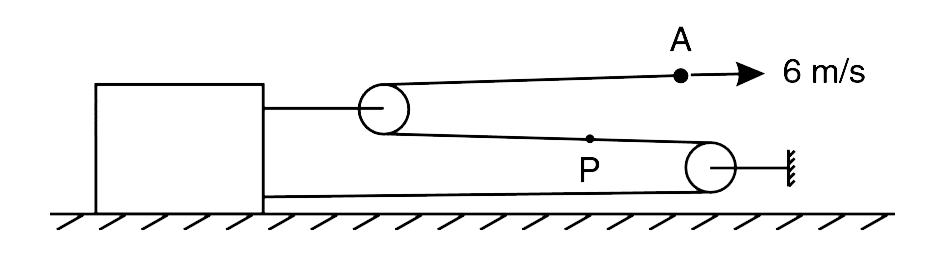
View SolutionIn the arrangement shown in the fig, end A of the string is being pulled with a constant horizontal velocity of 6m/s. The block is free to slide on the horizontal surface and all string segments are horizontal. Find the velocity of point P on the thread.
View SolutionA small sphere is suspended by a string from the ceiling of a car. If the car beings to move with a constant acceleration a, the inclination of the string to the vertical is:
View Solution