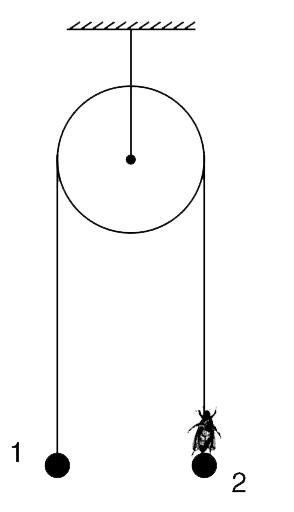
A light inextensible string, passing over a pulley, supports two particles 1 and 2 at its ends. An insect of mass m is sitting on particle 2 and the system is in equilibrium. The sum of masses of particles and the insect is M. Now the insect crawls a distance $x$ up relative to the string. Find the displacement of centre of mass of the system of two particles and the insect. In which direction does the centre of mass move and why ?

Video Solution

Text Solution

Verified by Experts

The correct Answer is: $\frac{m x}{M}$

Updated on:21/07/2023

Answer

Step by step video, text & image solution for A light inextensible string, passing over a pulley, supports two particles 1 and 2 at its ends. An insect of mass m is sitting on particle 2 and the system is in equilibrium. The sum of masses of particles and the insect is M. Now the insect crawls a distance x up relative to the string. Find the displacement of centre of mass of the system of two particles and the insect. In which direction does the centre of mass move and why ? by Physics experts to help you in doubts & scoring excellent marks in Class 11 exams.

Updated on:21/07/2023

Class 11 PHYSICS CENTRE OF MASS

Topper's Solved these Questions

Knowledge Check

The centre of mass of two particles system lies
Aat the midpoint on the line joining the two particles.
Bat one end of line joining the two particles
Con the line perpendicular to the line joining two particles
Don the line joining the two particles
If ${\to R}_{C M}$ is the position of the centre of mass of a system of two particles of masses $m_{1}$ and $m_{2}$ then ${\to R}_{C M}$ is given by :
A $\frac{m_{1} + m_{2}}{m_{1} {\to r}_{1} + m_{2} {\to r}_{2}}$
B $\frac{m_{1} m_{2}}{m_{1} {\to r}_{1} + m_{2} {\to r}_{2}}$
C $\frac{m_{1} {\to r}_{1} + m_{2} {\to r}_{2}}{m_{1} + m_{2}}$
D $\frac{m_{1} {\to r}_{1} + m_{2} {\to r}_{2}}{m_{1} m_{2}}$
Two masses m and M are attached to the strings as shown in the figure. If the system is in equilibrium, then

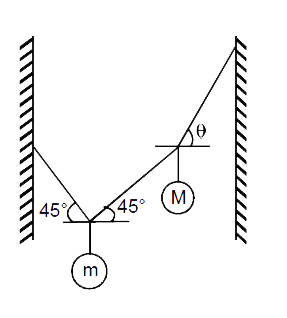A $tan θ = 1 + \frac{2 M}{m}$
B $tan θ = 1 + \frac{2 m}{M}$
C $cot θ = 1 + \frac{2 M}{m}$
D $cot θ = 1 + \frac{2 m}{M}$