Question

Match the boiling point with $K_b$ for $x,y$ and $z$, if molecular weight of $x,y$ and $z$ are same.
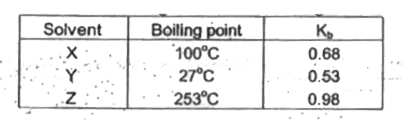
$\begin{array}{ccccc}& b.pt& & k_b& \\ x& 100& & 0.68& \\ y& 27& & 0.53& \\ z& 253& & 0.98& \end{array}$

Answer

`K_(b)` for `X=0.68,K_(b)` for `Y=0.53` and `K_(b)` for `Z=0.98`.

Explanation

`K_(b)=(RT_(b)^(2).M)/(1000xxDeltaH_(v))=(RT_(b).M)/(1000xxDeltaS_(v))`
Since, during vaporsiation entropy increases because randomness increases and thus `DeltaS_("vap")=S_(v)-S_(L)=+ve`. Also `DeltaS_("vap")` is almost constant for liquid `hArr` vapour even if the extent of disordemess varies from liquid to liquid because `DeltaS_("vap")` is very high. Thus, if `M` and `R` and constants, `K_(b)propT_(b)`.
Thus, `K_(b)` for `X=0.68, K_(b)` for Y=0.53` and `Z=0.98`.