A disc of mass M = 2m and radius R is pivoted at its centre. The disc is free to rotate in the vertical plane about its horizontal axis through its centre O. A particle of mass m is stuck on the periphery of the disc. Find the frequency of small oscillations of the system about its equilibrium position.
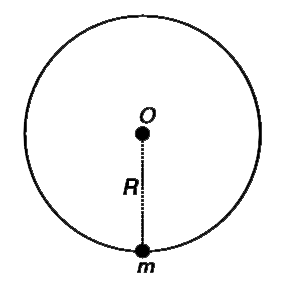
The correct Answer is:f=12π√g2R
Step by step video, text & image solution for A disc of mass M = 2m and radius R is pivoted at its centre. The disc is free to rotate in the vertical plane about its horizontal axis through its centre O. A particle of mass m is stuck on the periphery of the disc. Find the frequency of small oscillations of the system about its equilibrium position. by Physics experts to help you in doubts & scoring excellent marks in Class 11 exams.
Topper's Solved these Questions
- BOOK - ARIHANTCHAPTER - SIMPLE HARMONIC MOTIONEXERCISE - Level 233videos
SIMPLE HARMONIC MOTION
- BOOK - ARIHANTCHAPTER - SIMPLE HARMONIC MOTIONEXERCISE - Level 39videos
SIMPLE HARMONIC MOTION
- BOOK - ARIHANTCHAPTER - ROTATIONAL MOTIONEXERCISE - PHYSICS159videos
ROTATIONAL MOTION
- BOOK - ARIHANTCHAPTER - SURFACE TENSIONEXERCISE - Surface tension33videos
SURFACE TENSION
Similar Questions
One end of a metal rod of length L and mass M is pivoted to a fixed support and to its free end, a thin disc of mass m and radius r (L) is attached at its centre. In one case, the disc is attached to the rod in such a way that it is not free to rotate about its centre and in another case, teh disc is free to rotate about its centre. When the rod-disc system is displaced slightly from its equilibrium position and released. it peforms S.H.M. in vertical plane. Compare the restoring torque acting on the system and angular frequency in both the cases.
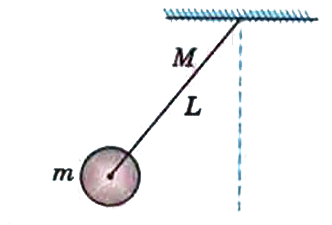 View Solution
View SolutionA disc of mass 'm' is suspended at a point R/2 above its centre. Find its period of oscillation.
View Solution
Knowledge Check
- Question 1 - Select One
A disc is free to rotate about a smooth horizontal axis passing through its centre of mass. A particle is fixed at the top of the disc. A slight push is given to the disc and it starts rotating. During the process.
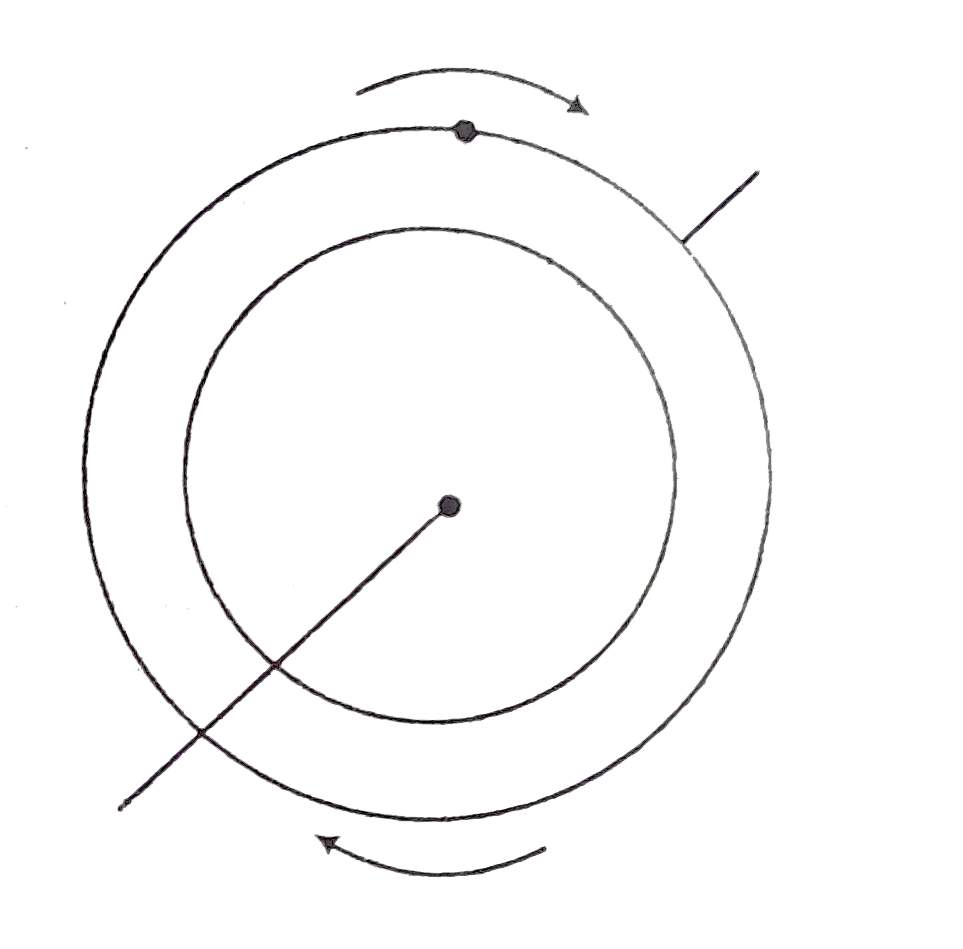 Aonly mechanical energy is conservedBonly angular momentum (about the axis of rotation) is conservedCboth mechanical energy and angular momentum are conservedDNeither the mechanical energy nor the angular momentum are conserved.
Aonly mechanical energy is conservedBonly angular momentum (about the axis of rotation) is conservedCboth mechanical energy and angular momentum are conservedDNeither the mechanical energy nor the angular momentum are conserved. - Question 2 - Select One
A disc of radius R is pivoted at its rim. The period for small oscillations about an axis perpendicular to the plane of disc is
A2π√RgB2π√2RgC2π√2π3gD2π√3R2g - Question 3 - Select One
A uniform disc of radius a and mass m, is rotating freely with angular speed ω in a horizontal plane. About a smooth fixed vertical axis through its centre. A particle, also of mass m, is suddently attached to the rim of the disc of the disc and rotates with it. The new angular speed is
Aω6Bω3Cω2Dω5
There is a ring or mass m and radius R is pivoted at a point O on its periphery. It is free to rotate about an axis perpendicular to its plane. What is the period of ring?
View SolutionA uniform disc of radius 'a' and mass 'm' is rotating freely with angular speed ω in a horizontal plane about a smooth fixed vertical axis through its centre. A particle of mass 'm' is then suddenly attached to the rim of the disc and rotates with it. The new angular speed is
View SolutionA disc is made to oscillate about a horizontal axis passing through mid point of its radius. Determine time period.
View SolutionA disc of mass m and radius R is free to rotate in a horizontal plane about a vertical smooth fixed axis passing through its centre. There is a smooth groove along the diameter of the disc and two small balls of mass m/2 each are placed in it on either side of the centre of the disc as shown in Fig. The disc is given an initial angular velocity ω0 and released.

The net work done by forces exerted by the disc on the ball for the duration ball remains on the disc isView SolutionA disc of mass m and radius R is free to rotate in a horizontal plane about a vertical smooth fixed axis passing through its centre. There is a smooth groove along the diameter of the disc and two small balls of mass m/2 each are placed in it on either side of the centre of the disc as shown in Fig. The disc is given an initial angular velocity ω0 and released.

The angular speed of the disc when the balls reach the end of the disc isView Solution

