(i) A particle of mass m executes SHM in xy- plane along a straight line AB. The points A (a, a) and B (- a, - a) are the two extreme positions of the particle. The particle takes time T to move from one extreme A to the other extreme B. Find the x component of the force acting on the particle as a function of time if at t = 0 the particle is at A. (ii) Two particle A and B are performing SHM along X-axis and Y-axis respectively with equal amplitude and frequency of 2 cm and 1 Hz respectively. Equilibrium positions for the particles A and B are at the coordinate (3, 0) and (0, 4) respectively. At t = 0, B is at its equilibrium position and moving toward the origin, while A is nearest to the origin. Find the maximum and minimum distances between A and B during their course of motion
The correct Answer is:(i) −4π2T2macos(2πTt)
(ii) 7 cm and 3 cm.
Step by step video, text & image solution for (i) A particle of mass m executes SHM in xy- plane along a straight line AB. The points A (a, a) and B (- a, - a) are the two extreme positions of the particle. The particle takes time T to move from one extreme A to the other extreme B. Find the x component of the force acting on the particle as a function of time if at t = 0 the particle is at A. (ii) Two particle A and B are performing SHM along X-axis and Y-axis respectively with equal amplitude and frequency of 2 cm and 1 Hz respectively. Equilibrium positions for the particles A and B are at the coordinate (3, 0) and (0, 4) respectively. At t = 0, B is at its equilibrium position and moving toward the origin, while A is nearest to the origin. Find the maximum and minimum distances between A and B during their course of motion by Physics experts to help you in doubts & scoring excellent marks in Class 11 exams.
Topper's Solved these Questions
- BOOK - ARIHANTCHAPTER - SIMPLE HARMONIC MOTIONEXERCISE - Level 39videos
SIMPLE HARMONIC MOTION
- BOOK - ARIHANTCHAPTER - SIMPLE HARMONIC MOTIONEXERCISE - Level 39videos
SIMPLE HARMONIC MOTION
- BOOK - ARIHANTCHAPTER - ROTATIONAL MOTIONEXERCISE - PHYSICS159videos
ROTATIONAL MOTION
- BOOK - ARIHANTCHAPTER - SURFACE TENSIONEXERCISE - Surface tension33videos
SURFACE TENSION
Similar Questions
A particle moves in xy plane with its position vector changing with time (t) as →r=(sint)ˆi+(cost)ˆj ( in meter)
Find the tangential acceleration of the particle as a function of time. Describe the path of the particle.View SolutionA particle can move along a straight line. It is at rest when a force (F) starts acting on it directed along the line. Work done by the force on the particle changes with time(t) according to the graph shown in the fig. Can you say that the force acting on the particle remains constant with time?
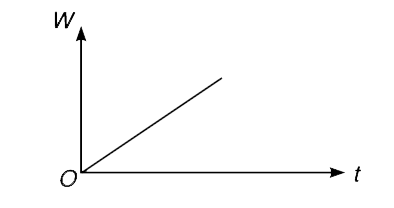 View Solution
View Solution
Knowledge Check
- Question 1 - Select One
It takes 1 s for the bob to moves from one extreme position to other extreme postion. Then the time period of the pendulum is ______ s.
A1B2 hC3D4 - Question 2 - Select One or More
Kinetic energy of a particle moving in a straight line is proportional to the time t. The magnitude of the force acting on the particle is :
ADirectly proportional to the speed of the particleBInversely proportional to √tCInversely proportional to the speed of the particleDDirectly proportional to √t - Question 3 - Select One
It takes 1 s for the bob to moves from one extreme position to other extreme postion. Then the time period of the pendulum is ______ s.
A1B2hC3D4
When a particle executing a linear S.H.M. moves from its extreme position to the mean position, its
View SolutionA particle of mass m is moving along the line y-b with constant acceleration a. The areal velocity of the position vector of the particle at time t is (u=0)
View SolutionParticle moves from extreme position to mean position, its
View SolutionTwo particles A and B execute SHM on a straight line of path length 2 m starting from the two extreme points simultaneously. If their respective time periods are 1 s and 2 s, the minimum time in which they meet is
View SolutionA particle executing SHM of amplitude 4 cm and T = 4s. The time taken by it to move from positive extreme position to half the amplitude is
View Solution

