Rectangle ABCD has area 200. An ellipse with area passes through A and C and has foci at B and D. If the perimeter of the rectangle is P. Then the value of is
2
4
8
None of these
The correct Answer is:C
To solve the problem, we need to find the perimeter P of rectangle ABCD given the area and the properties of the ellipse.
1. Understanding the Rectangle and Its Area:
- Let the length of rectangle ABCD be L and the width be W.
- The area of the rectangle is given by:
L×W=200
2. Ellipse Properties:
- The area of the ellipse is given by:
Area of ellipse=π×a×b=200π
where a is the semi-major axis and b is the semi-minor axis.
- From this, we can deduce:
a×b=200
3. Foci of the Ellipse:
- The foci of the ellipse are located at points B and D.
- The distance between the foci 2c is given by:
c=√a2−b2
4. Relating Rectangle Dimensions to Ellipse:
- The distance BD (the distance between the foci) can be expressed in terms of the rectangle's dimensions:
BD=√L2+W2
- Since B and D are the foci, we have:
2c=√L2+W2
5. Using the Area of the Rectangle:
- From the area equation L×W=200, we can express W in terms of L:
W=200L
6. Substituting into the Distance Equation:
- Substitute W into the equation for BD:
2c=√L2+(200L)2
- This simplifies to:
2c=√L2+40000L2
7. Finding the Value of c:
- We know from the ellipse properties that:
c=√a2−b2
- Using the area relation a×b=200 and substituting a=200b:
c=√(200b)2−b2
8. Perimeter of the Rectangle:
- The perimeter P of the rectangle is given by:
P=2(L+W)=2(L+200L)
9. Finding P/20:
- We need to find P20:
P20=2(L+200L)20=L+200L10
10. Final Calculation:
- To find the specific value of P20, we can assume L=20 and W=10 (since 20×10=200):
P=2(20+10)=60
P20=6020=3
Final Answer:
P20=3
- Let the length of rectangle ABCD be L and the width be W.
- The area of the rectangle is given by:
L×W=200
- The area of the ellipse is given by:
Area of ellipse=π×a×b=200π
where a is the semi-major axis and b is the semi-minor axis.
- From this, we can deduce:
a×b=200
- The foci of the ellipse are located at points B and D.
- The distance between the foci 2c is given by:
c=√a2−b2
- The distance BD (the distance between the foci) can be expressed in terms of the rectangle's dimensions:
BD=√L2+W2
- Since B and D are the foci, we have:
2c=√L2+W2
- From the area equation L×W=200, we can express W in terms of L:
W=200L
- Substitute W into the equation for BD:
2c=√L2+(200L)2
- This simplifies to:
2c=√L2+40000L2
- We know from the ellipse properties that:
c=√a2−b2
- Using the area relation a×b=200 and substituting a=200b:
c=√(200b)2−b2
- The perimeter P of the rectangle is given by:
P=2(L+W)=2(L+200L)
- We need to find P20:
P20=2(L+200L)20=L+200L10
- To find the specific value of P20, we can assume L=20 and W=10 (since 20×10=200):
P=2(20+10)=60
P20=6020=3
P20=3
Topper's Solved these Questions
Similar Questions
Knowledge Check
- Question 1 - Select One
Rectangle ABCD has area 200 sq. unit.An ellipse with area 200π sq.unit passes through A and C has focii at B and D. Then perimeter of the rectangle ABCD is
A80 unitB40 unitC20 unitD60 unit - Question 2 - Select One
Rectangle ABCD has area 200 sq. unit. An ellipse with area 200π. unit passes through A and C has focii at B and D. Then perimeter of the rectangle ABCD is
A80 unitB40 unitC20 unitD60 unit - Question 3 - Select One
The diagonal of a rectangle is √41cm and its area is 20 sq. cm. The perimeter of the rectangle must be (a) 9 cm (b) 18 cm (c) 20 cm (d) 41 cm
A9 cmB18 cmC41 cmD20 cm
The given figure shows a rectangle ABCD.
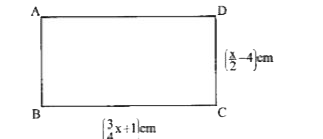
If perimeter of rectangle ABCD is 54 cm, then what is its area?View SolutionThe area of rectangle is twice the area of a triangle. The p"rimeter of the rectangle is 58 cm. What is the area of the triangle ?
View SolutionAn ellipse has foci (4, 2), (2, 2) and it passes through the point P (2, 4). The eccentricity of the ellipse is
View SolutionABCD is a rectangle and there are four equilateral triangles . Area of ΔASD equals to area of ΔBQC and area of ΔDRC equals to area of ΔAPB. The perimeter of the rectangle is 12 cm. Also the sum of the areas of the four triangles is 10√3cm2 then the total area of the figures thus formed :
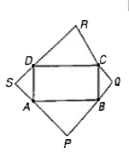 View Solution
View SolutionIf the length of a rectangle is increased by 40%, and the breadth is decreased by 20%. then the area of the rectangle increases by x%. Then the value of x is:
View Solution

