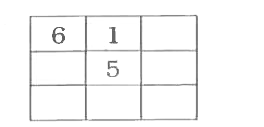
In a “magic square”, the sum of the numbers in each row, in each column and along the diagonal is the same. Is this a magic square? TABLE

Video Solution

Answer

Step by step video & image solution for In a “magic square”, the sum of the numbers in each row, in each column and along the diagonal is the same. Is this a magic square? TABLE by Maths experts to help you in doubts & scoring excellent marks in Class 7 exams.

Updated on:21/07/2023

Class 7 MATHS FRACTIONS AND DECIMALS

Topper's Solved these Questions

EXPONENTBOOK - RD SHARMACHAPTER - EXPONENTEXERCISE - All Questions
124 Videos
INTEGERSBOOK - RD SHARMACHAPTER - INTEGERSEXERCISE - All Questions
52 Videos

Knowledge Check

In a $4 \times 4$ matrix the sum of each row, column and both the main diagonals is $α$ . Then the sum of the four corner elements
Ais also $α$
Bmay not be $α$
Cis never equal to $α$
Dnone of these