In Figure, line n is a transversal to line l and m. Identify the following: i) Alternate and corresponding angles in Fig. 58 (i)
To solve the problem of identifying alternate and corresponding angles in the given figure where line n is a transversal to lines l and m, we can follow these steps:
1. Identify the Lines and Angles:
- Recognize that lines l and m are parallel lines, and line n is the transversal that intersects them. This creates eight angles at the intersection points.
2. Label the Angles:
- For clarity, label the angles formed at the intersections. Let's denote the angles as follows:
- At the intersection of line n with line l: Angles 1, 2, 3, and 4.
- At the intersection of line n with line m: Angles 5, 6, 7, and 8.
3. Identify Alternate Angles:
- Alternate Interior Angles: These are the pairs of angles that are on opposite sides of the transversal (line n) and inside the parallel lines (lines l and m).
- Angle 3 and Angle 5 are alternate interior angles.
- Angle 4 and Angle 6 are alternate interior angles.
- Alternate Exterior Angles: These are the pairs of angles that are on opposite sides of the transversal and outside the parallel lines.
- Angle 1 and Angle 7 are alternate exterior angles.
- Angle 2 and Angle 8 are alternate exterior angles.
4. Identify Corresponding Angles:
- Corresponding angles are the angles that are in the same relative position at each intersection.
- Angle 1 corresponds to Angle 5.
- Angle 2 corresponds to Angle 6.
- Angle 3 corresponds to Angle 7.
- Angle 4 corresponds to Angle 8.
Summary of Findings:
- Alternate Angles:
- Alternate Interior Angles: (3, 5) and (4, 6)
- Alternate Exterior Angles: (1, 7) and (2, 8)
- Corresponding Angles:
- (1, 5), (2, 6), (3, 7), and (4, 8)
- Recognize that lines l and m are parallel lines, and line n is the transversal that intersects them. This creates eight angles at the intersection points.
- For clarity, label the angles formed at the intersections. Let's denote the angles as follows:
- At the intersection of line n with line l: Angles 1, 2, 3, and 4.
- At the intersection of line n with line m: Angles 5, 6, 7, and 8.
- Alternate Interior Angles: These are the pairs of angles that are on opposite sides of the transversal (line n) and inside the parallel lines (lines l and m).
- Angle 3 and Angle 5 are alternate interior angles.
- Angle 4 and Angle 6 are alternate interior angles.
- Alternate Exterior Angles: These are the pairs of angles that are on opposite sides of the transversal and outside the parallel lines.
- Angle 1 and Angle 7 are alternate exterior angles.
- Angle 2 and Angle 8 are alternate exterior angles.
- Corresponding angles are the angles that are in the same relative position at each intersection.
- Angle 1 corresponds to Angle 5.
- Angle 2 corresponds to Angle 6.
- Angle 3 corresponds to Angle 7.
- Angle 4 corresponds to Angle 8.
- Alternate Angles:
- Alternate Interior Angles: (3, 5) and (4, 6)
- Alternate Exterior Angles: (1, 7) and (2, 8)
- Corresponding Angles:
- (1, 5), (2, 6), (3, 7), and (4, 8)
Topper's Solved these Questions
Similar Questions
Knowledge Check
Condier the following statement
if a transversal line cuts two parallel line then
1 Each pair of corresponding angles are equal
2 Each pair of alternalte angles are unequal
Among these true statements areAonly 1Bonly 2Cboth 1 and 2Dneigher 1 nor 2Two parallel lines are cut by a transversal, then which of the following are true?
1. Pair of alternate interior angles are equal.
2. Pair of corresponding angles are equal.
3. Pair of interior angles on the same side of the transversal are supplementaryA2 and 3B1 and 3C1 and 2DAll of these
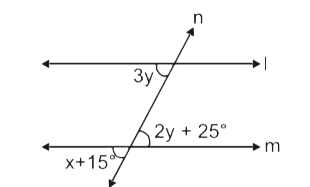
In the given figure l||m and n is the transversal , find x.
View SolutionIn Fig. 62, line l∣∣m and n is a transversal. If ∠1=400, find all the angles and check that all corresponding angles and alternate angles are equal.
View SolutionExplain how a transversal could intersect two other lines so that corresponding angles are not congruent.
View SolutionIf two parallel lines are intersected by a transversal; the bisector of any two corresponding angles are parallel.
View SolutionIn the figure, if line l || line m and line n is the transversal, then find the values of a and b.

View SolutionRead the following statements which are taken as axioms
(i) If a transversal intersects two parallel lines, then corresponding angles are not necessarily equal.
(ii) If a transversal intersect two parallel lines, then alternate interior angles are equal.
Is this system of axioms consistent ? Justify your answer.
View Solution
