Given a function, f(x), such that f(x) = f(|x|). Which one of the following could be the graph of f(x) ?
Text Solution
Since f(x) must = f(|x|), the graph must be symmetric about the y-axis.
|
Topper's Solved these Questions
- PIECEWISE FUNCTIONSBOOK - ENGLISH SATCHAPTER - PIECEWISE FUNCTIONSEXERCISE - EXERCISES
8 Videos
- PASSPORT TO ADVANCED MATHBOOK - ENGLISH SATCHAPTER - PASSPORT TO ADVANCED MATHEXERCISE - EXERCISE
80 Videos
- PLANE GEOMETRY/ TRIGONOMETRYBOOK - ENGLISH SATCHAPTER - PLANE GEOMETRY/ TRIGONOMETRYEXERCISE - EXERCISE
12 Videos
Similar Questions
Knowledge Check
If f(x)=4x+2, which of the following is the graph of f(x)?
A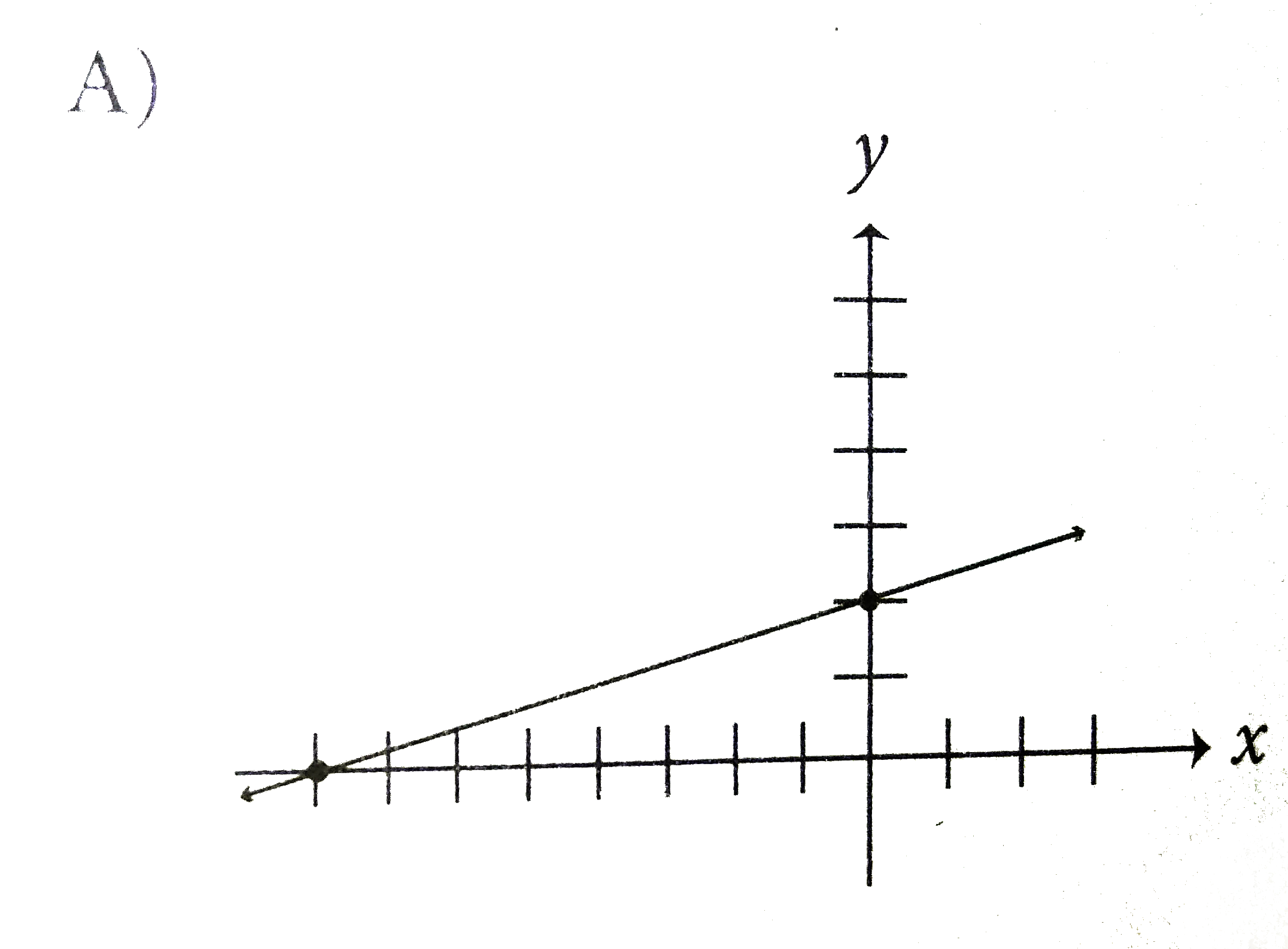 B
B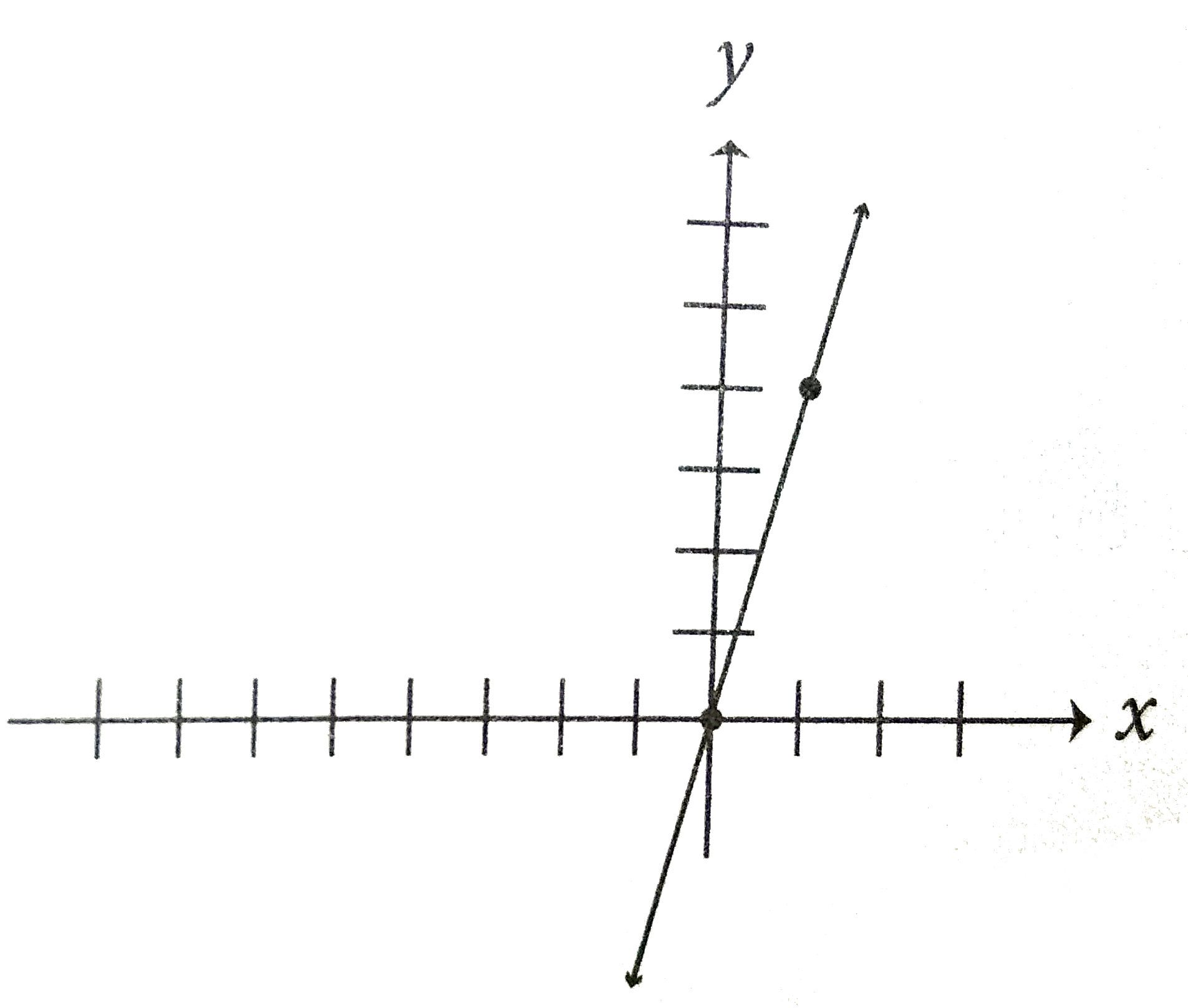 C
C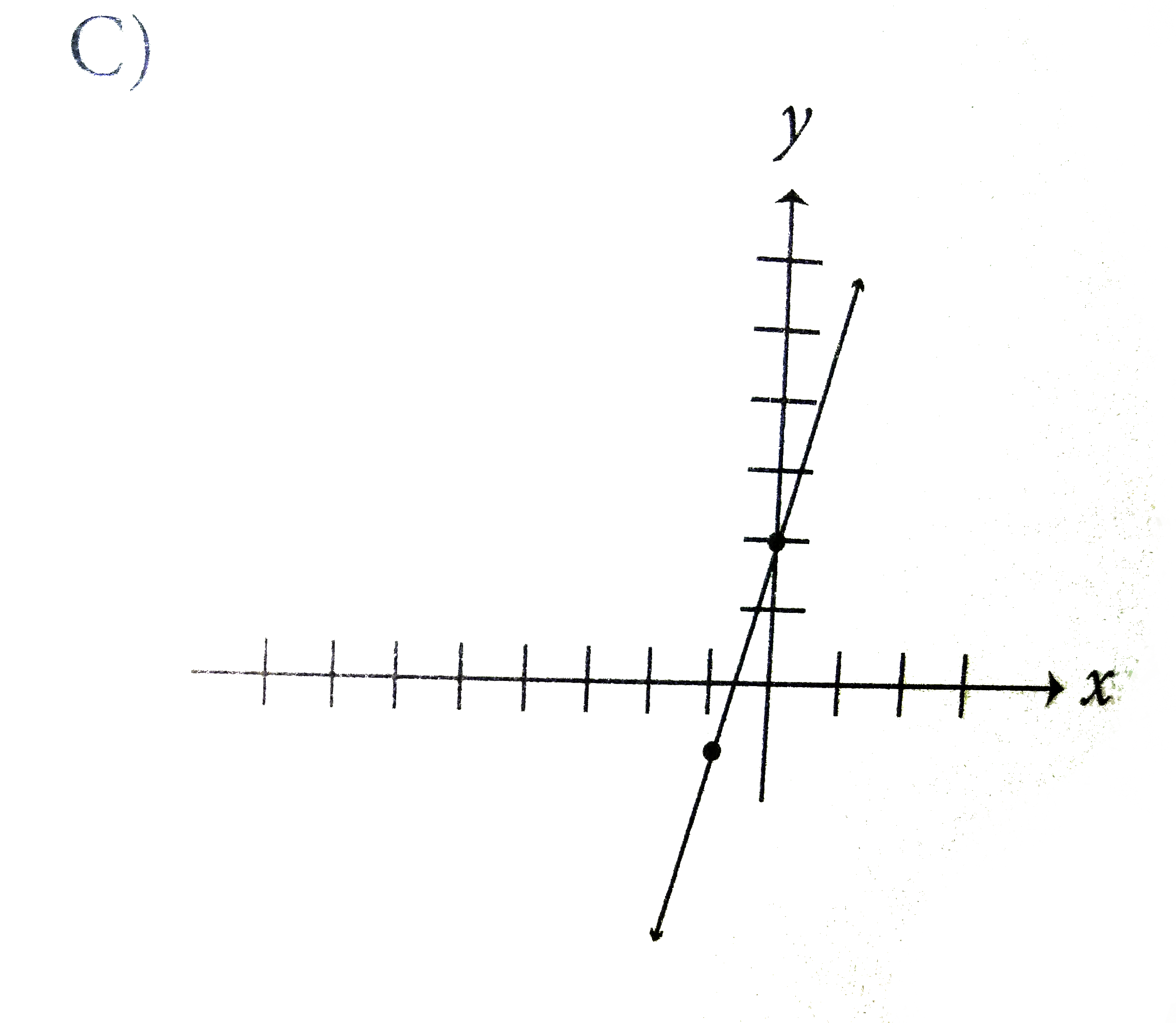 D
D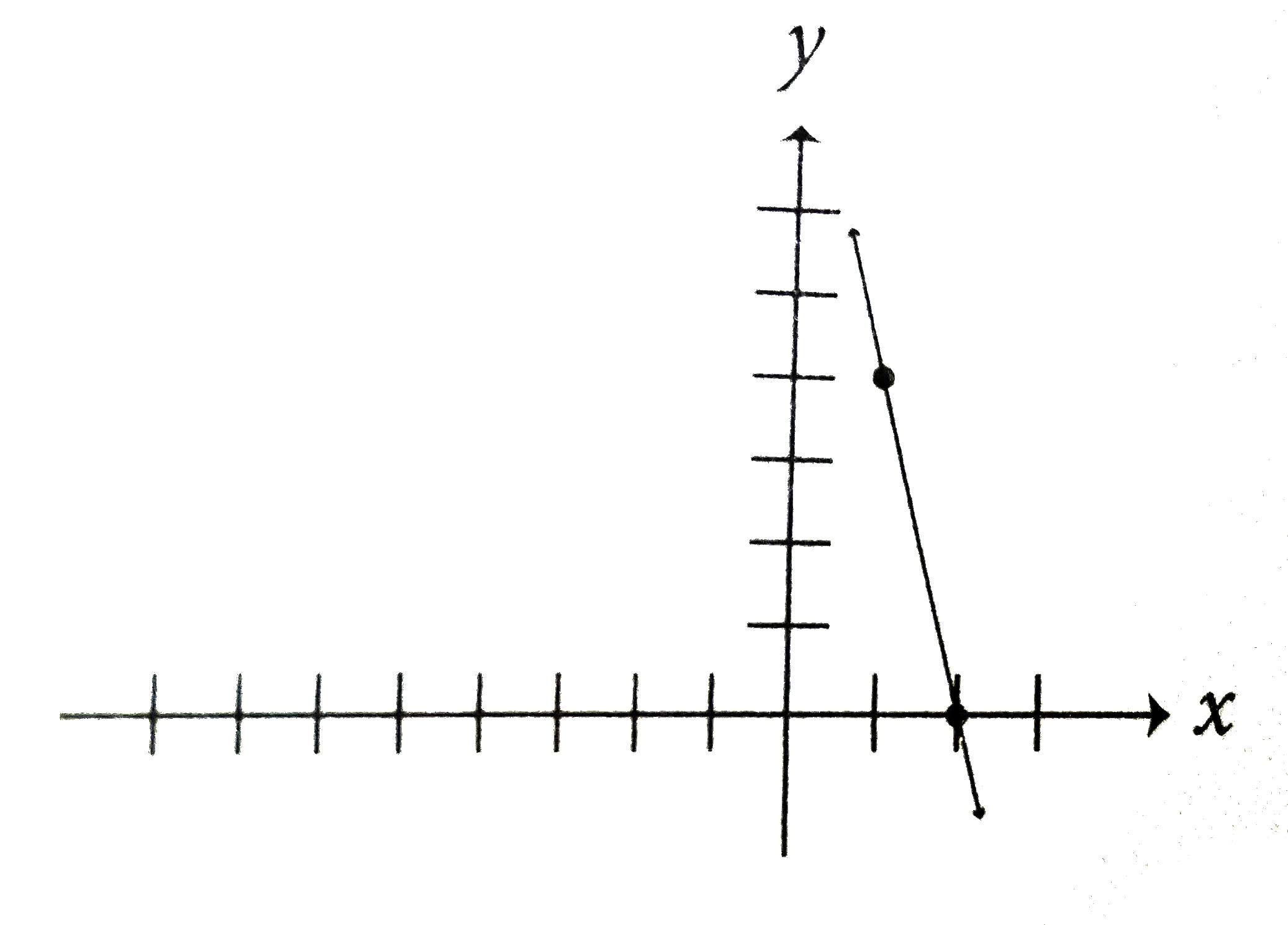
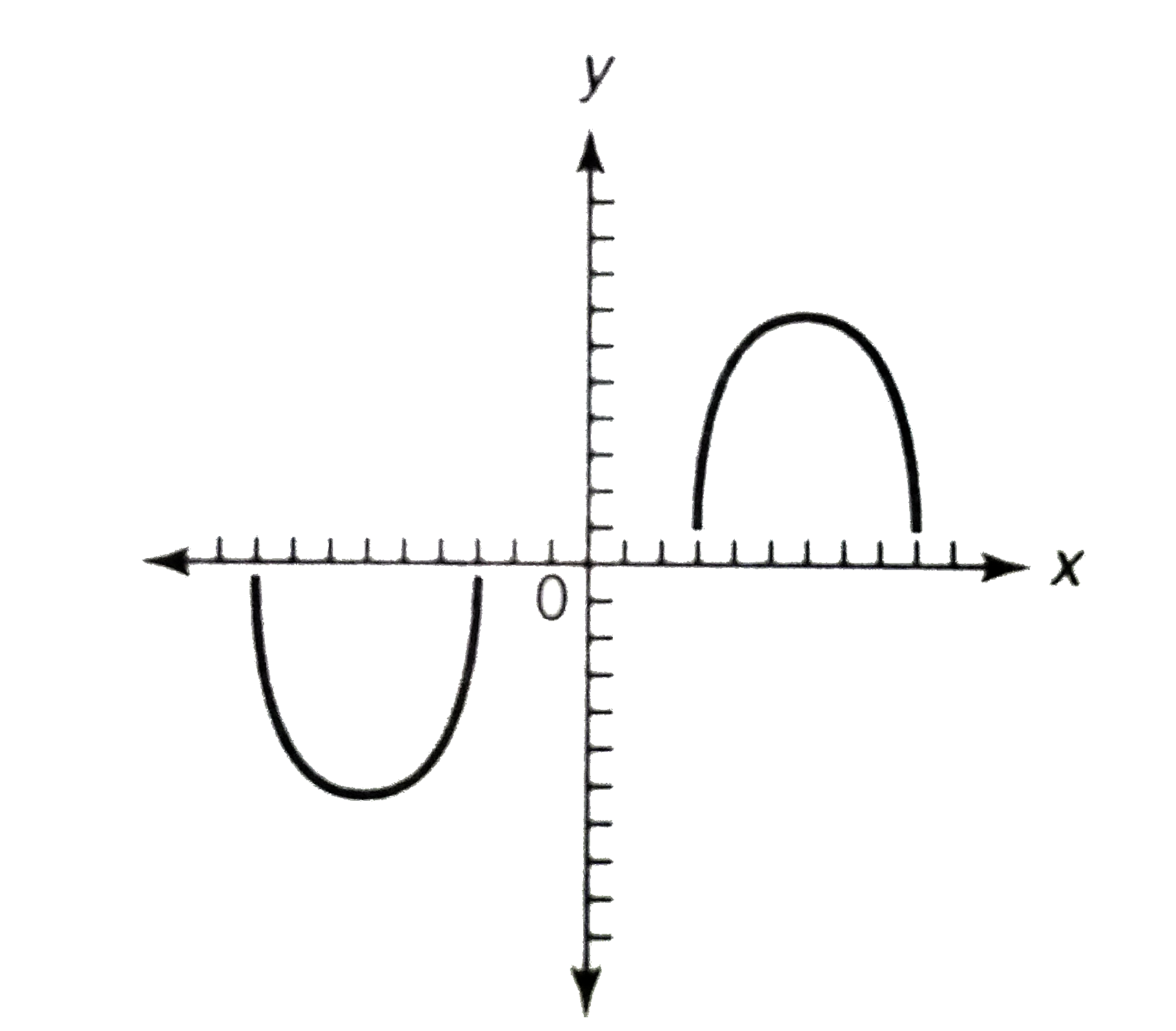
The graph of the function f is shown above. Which of the following could represent the graph of y=|f(x)|?A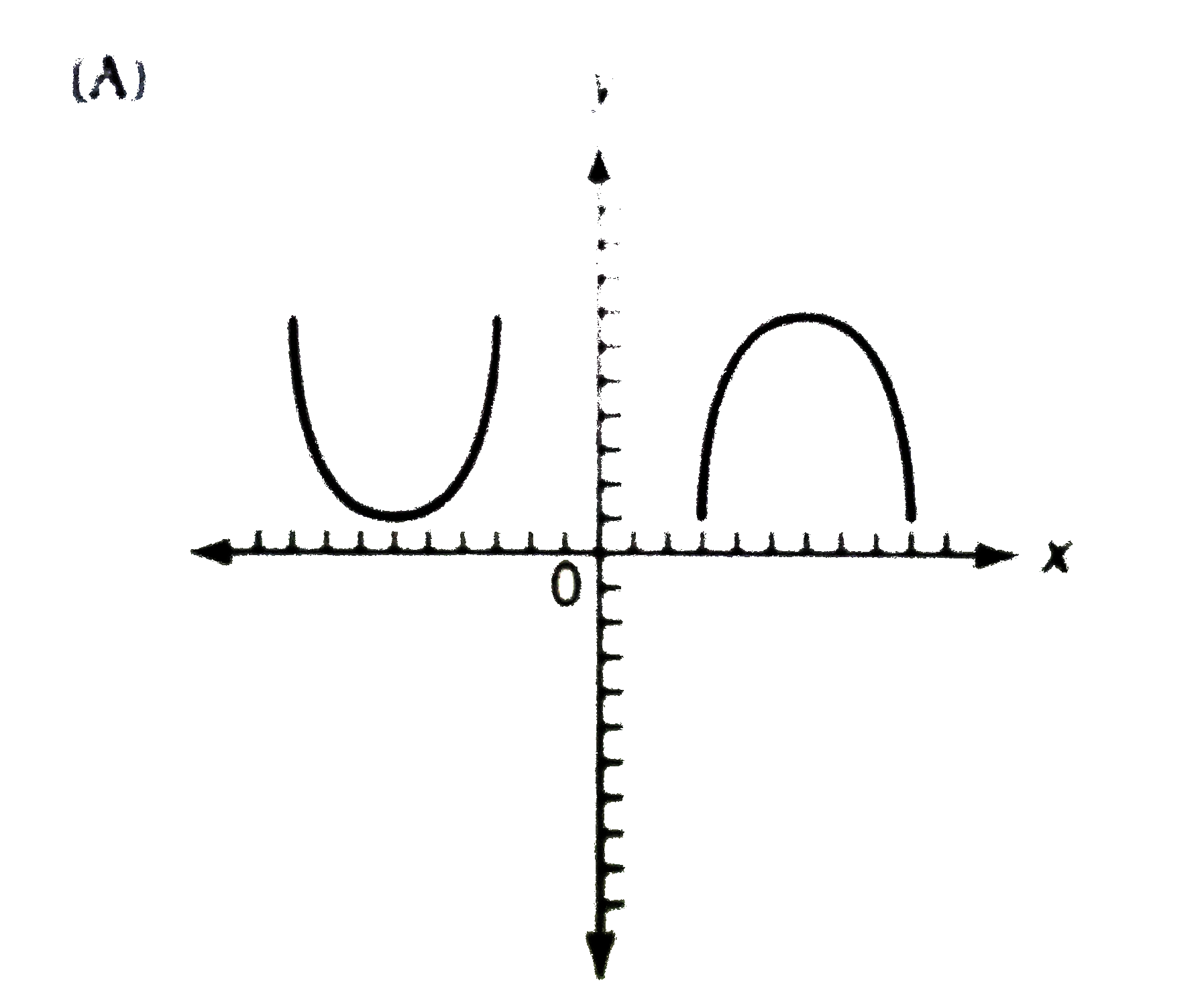 B
B C
C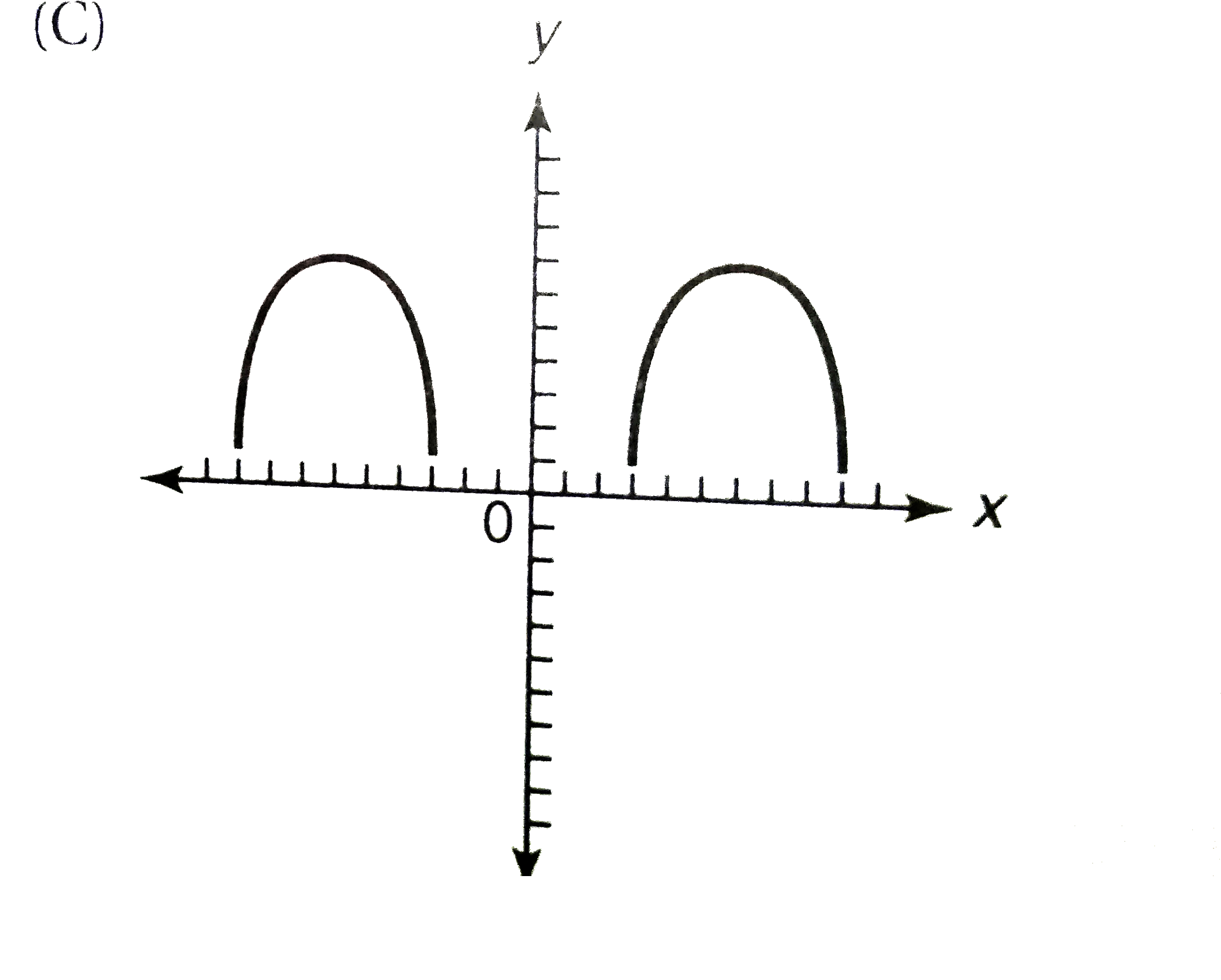 D
D
The graph of y=f(x) is shown to the right. If f(−1)=v, then which one of the following could be the value of f(v) ?
 A0B1C2D2.5
A0B1C2D2.5
The graph of the function y=f(x). Then which of the following curve represent the graph of the function y=|f(x)|
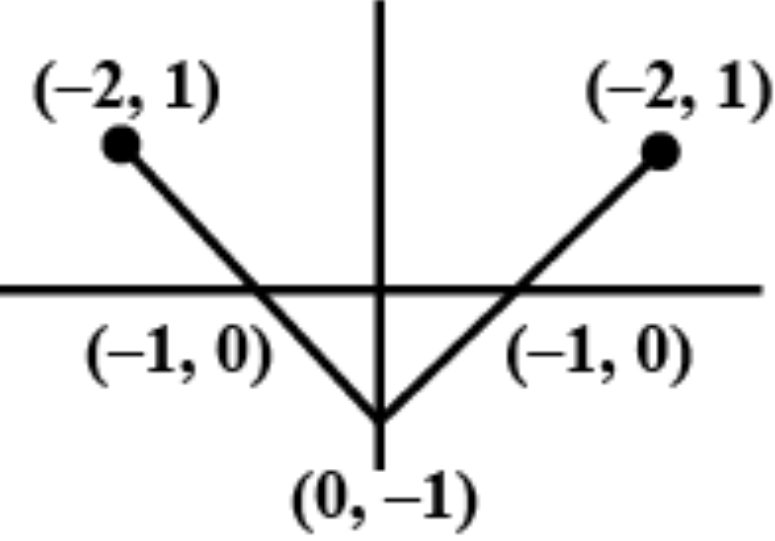
View SolutionThe graph of y=f(x) is shown below.
Which of the following could represents the graph of y=f(x−2)+1?
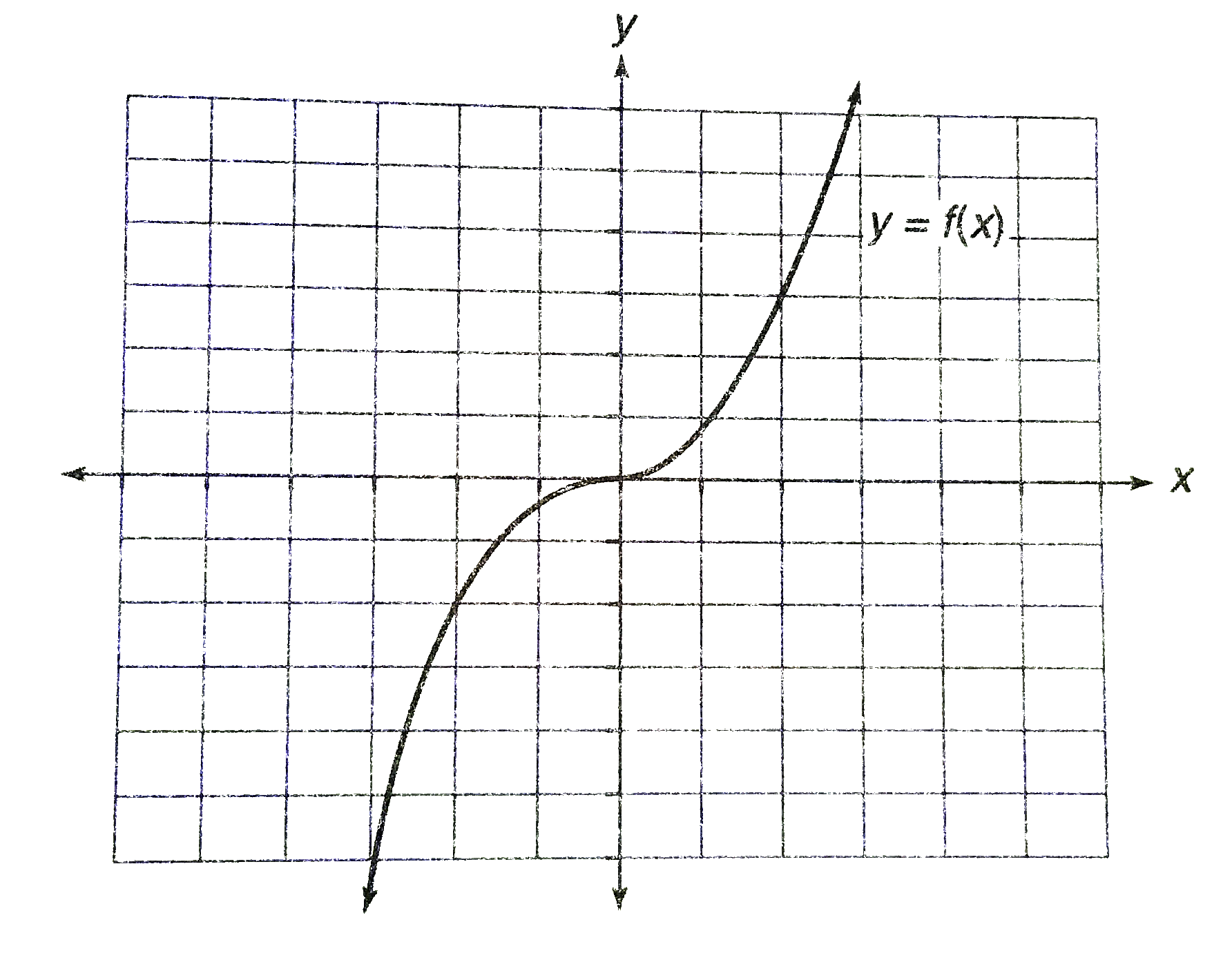
View Solution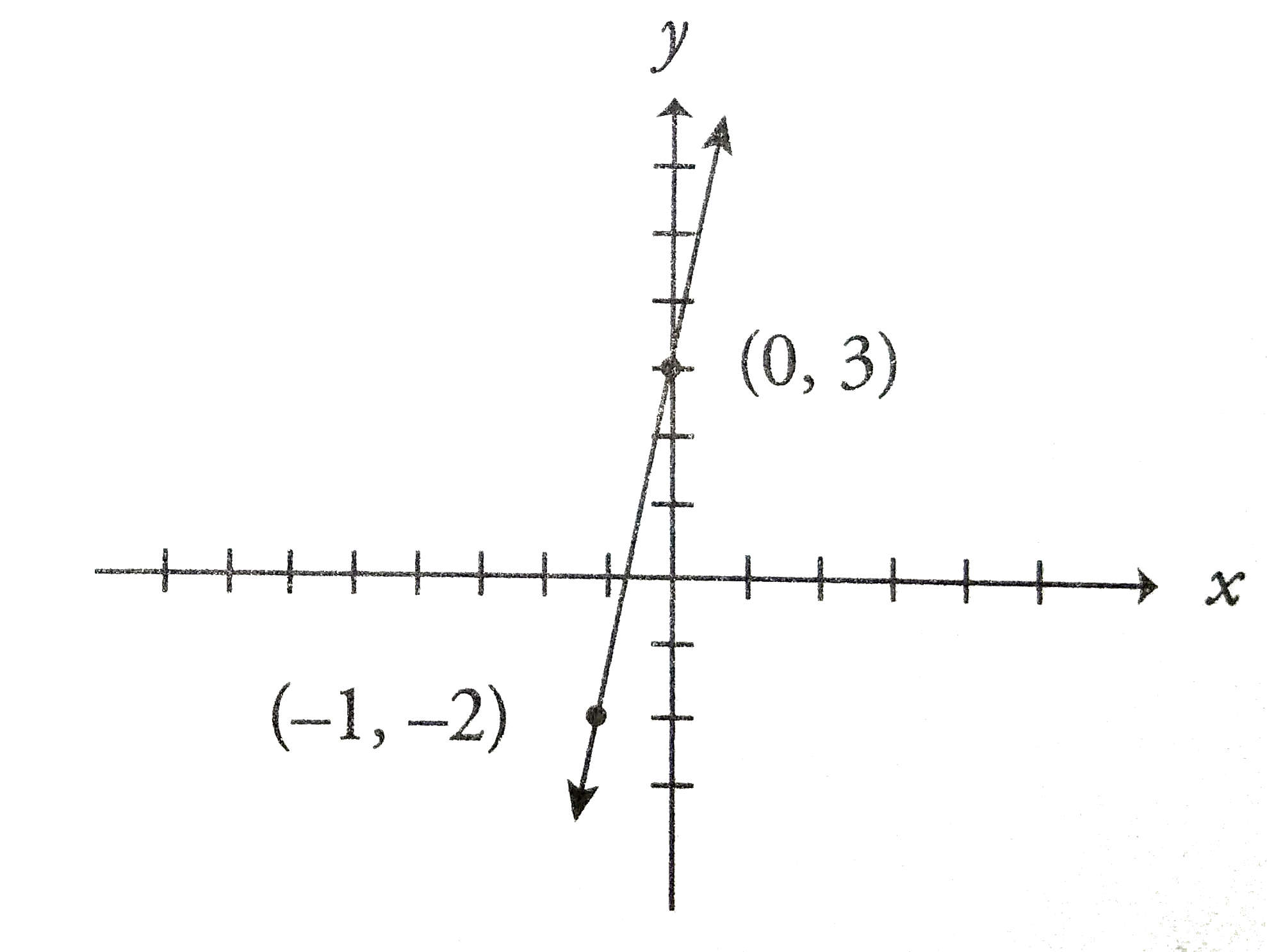
If the graph above is that of f(x), which of the following could be f(x)?
View Solution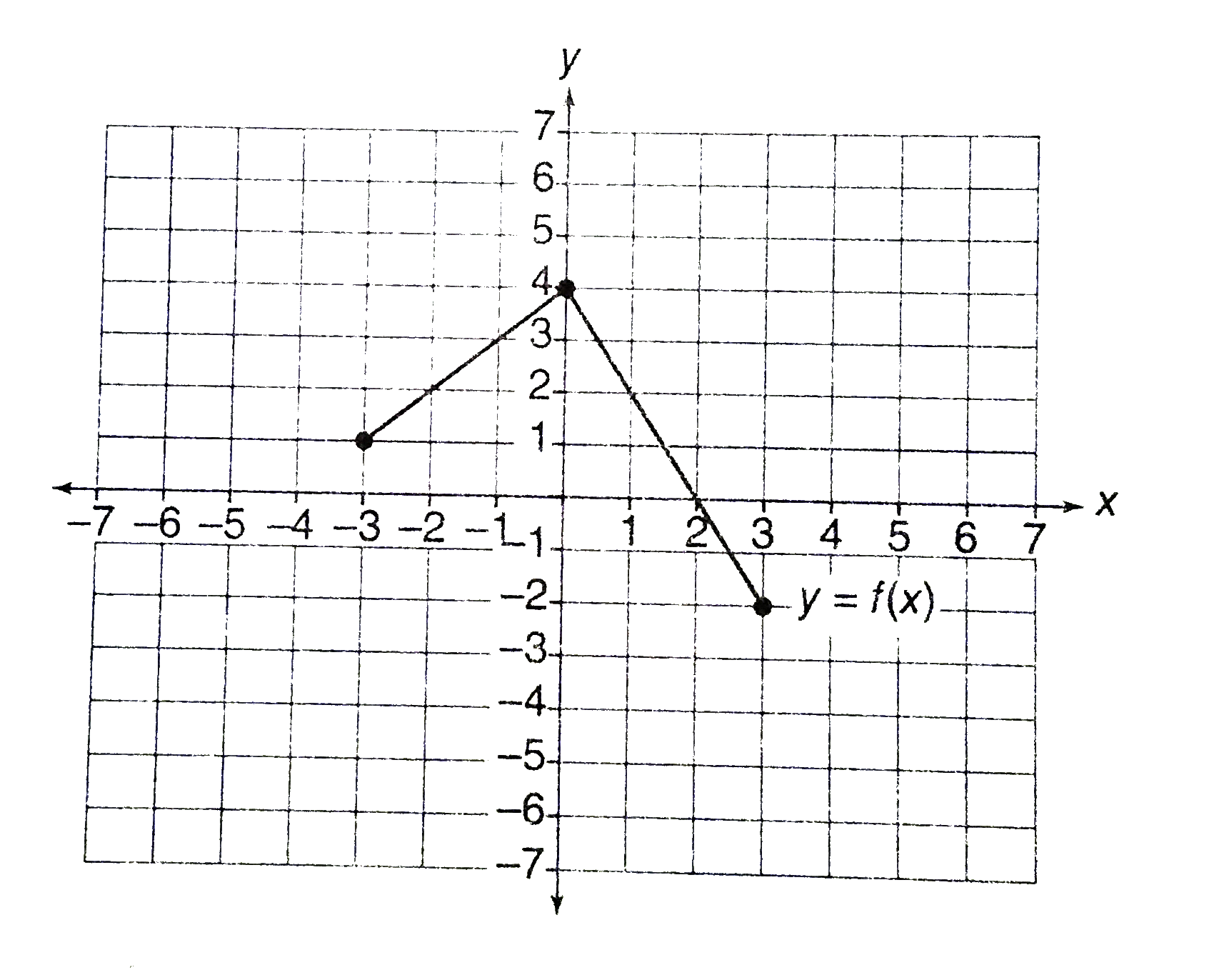
If the accomanying figure above shows the graph of function f, which of the following could represent the graph of y=f(x+1)?
View SolutionIn the xy-plane, the graph of function f has x-intercepts at -3, -1, and 1. Which of the following could define f ?
View Solution
