A sphere of radius 10cm and density 500kg/m3 is under water of density 1000kg/m3 The acceleration of the sphere is 9.80m/s2 upward viscosity of water is 1.0 centipoise if g=9.81m/s2 the velocity of the sphere is
9m/s
10m/s
11m/s
12m/s
The correct Answer is:C
100×43πr3g−500×43πr3g−6πηrv=500×43πr3a
0.23=6×1×10−3
20018=v
11m/sAns
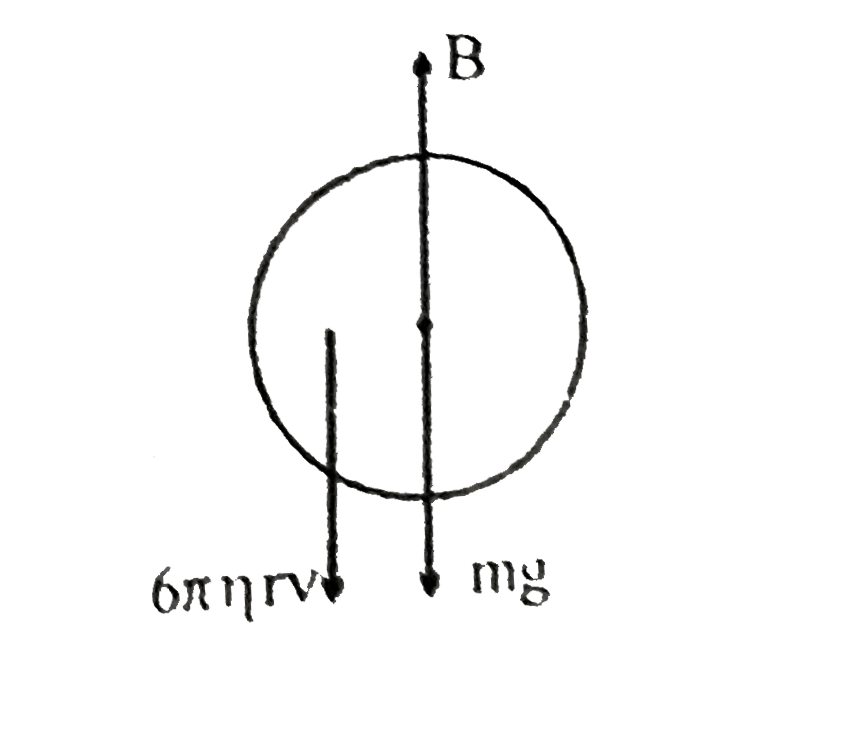
Topper's Solved these Questions
- MASTER PRACTICE PROBLEMBOOK - BANSALCHAPTER - MASTER PRACTICE PROBLEMEXERCISE - Comphrehension
134 Videos
- MASTER PRACTICE PROBLEMBOOK - BANSALCHAPTER - MASTER PRACTICE PROBLEMEXERCISE - Reasoning
75 Videos
- FLUID MECHANICSBOOK - BANSALCHAPTER - FLUID MECHANICSEXERCISE - PYQS AIEEE
10 Videos
- SEMICONDUCTORSBOOK - BANSALCHAPTER - SEMICONDUCTORSEXERCISE - CBSE Question
32 Videos
Similar Questions
Knowledge Check
An iceberg of density 900kg/m3 is floating in water of density 1000kg/m3. The percentage of volume of ice cube outside the water is
A20%B35%C10%D25%A cube of ice of side length 10 cm is floating in water of density 1000 kg/m3. Then pick up the correct statement (density of ice = 900 kg/m3)
A1 cm of the cube will be out of waterB9 cm of the cube will be out of waterC9 cm of the cube will be in waterD1 cm of the cube will be in waterA tank is filled with water of density 103kg/m3 and oil of density 9×103kg/m3.The height of water layer is 1m and that of the oil layer is 4M. The velocity of efflux front an opening in the bottom of the tank is
A√85m/sB√88m/sC√92m/sD√98m/s
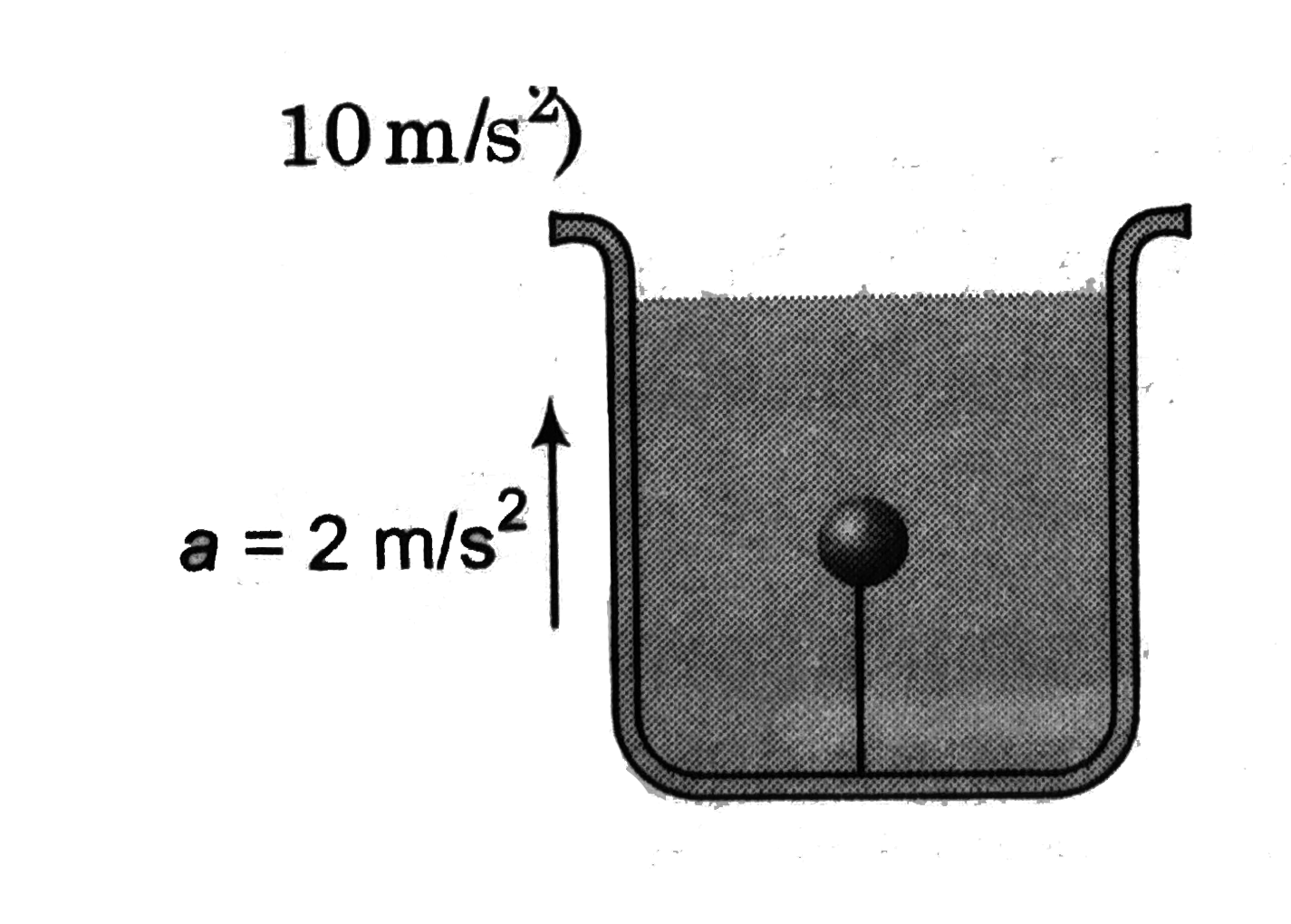
A solid sphere of mass m=2kg and density ρ=500kg/m3 is held stationary relative to a tank filled with water. The tank is acceerating upward with acceleration 2m/s2. Calculate
(a) Tension in the thread connected between the sphere and the bottom of the tank.
(b) If the thread snaps, calculate the acceleration of sphere with respect to the tank. (Density of water=1000kg/m3,g=10m/s2)
View SolutionA metallic sphere of density 5 g cm−3 is projected upward from the bottom of a pond with a velocity 10 m s−1. The velocity of the sphere on reaching 10 m s−1. The velocity of the sphere on reaching the surface of the water is found to be 8 m s−1.
Determine the pressure exerted by water at the bottom of the pond. (Neglect the viscous force acting on the sphere).
View SolutionA small wooden block of density 400kg/m3 is released from rest inside a swimming pool filled with water of density 103kg/m3. The acceleration of the block just after it is released is __________ m/s2(g=10m/s2)
View SolutionA uniform solid sphere has radius 0.2 m and density 8×103kg/m2. Fing the moment of inertia about the tangent to its surface. (π=3.142)
View SolutionCalculate the molarity of water if its density is 1000kg/m3.
View Solution
