A rocket drifting sideways in outer spaces from position 'a' to position 'b' with constant velocity. At 'b' the rocket's engine starts to produce constant thrust at right angles to line 'ab'. The engine turns off again as the rocket reaches some point 'c'. Assume that rocket is subjected to no other forces.

The path of rocket from point b to c will be 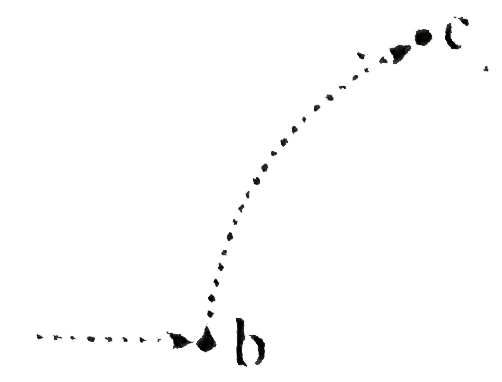
The path of rocket from point b to c will be 
The path of rocket beyond c will be
The speed continuously increases from b to c.
The correct Answer is:B, C, D
(B) Acceleration is upward with horizontal initial velocity so trajectory is parabolic.
(C) Acceleration is zero so velocity is constant.
(D) Due to acceleration speed increase
Topper's Solved these Questions
- BOOK - BANSALCHAPTER - MASTER PRACTICE PROBLEMEXERCISE - Subjective107videos
MASTER PRACTICE PROBLEM
- BOOK - BANSALCHAPTER - MASTER PRACTICE PROBLEMEXERCISE - Additional topic70videos
MASTER PRACTICE PROBLEM
- BOOK - BANSALCHAPTER - MASTER PRACTICE PROBLEMEXERCISE - Reasoning75videos
MASTER PRACTICE PROBLEM
- BOOK - BANSALCHAPTER - FLUID MECHANICSEXERCISE - PYQS AIEEE10videos
FLUID MECHANICS
- BOOK - BANSALCHAPTER - SEMICONDUCTORSEXERCISE - CBSE Question32videos
SEMICONDUCTORS
Similar Questions
A double stage rocket has an initial mass Mi, gas is exhausted front he rocket at a constant rate of dm/dt and with an exhaust velocity u relative to the rocket. When the mass of the rocket reaches the value μ, the first stage of mass m of
Which fuel is exhausted is disengaged from the rocket and then the rocket continues to the second stage at teh same rate and exhaust velocity as int eh first stage, until it reaches a mass Mf
(a). Calculate the rocket velocity at the end of the first stage, given that it is started at rest.
(b). Calculat ethe rocket velocity at the end of the second stage. ltbr> (c). What is the final velocity of a one stage rocket of the same initial Mi mass and the same amount of fuel ? it is greater or less than final velocity of the double stage rocket?View Solution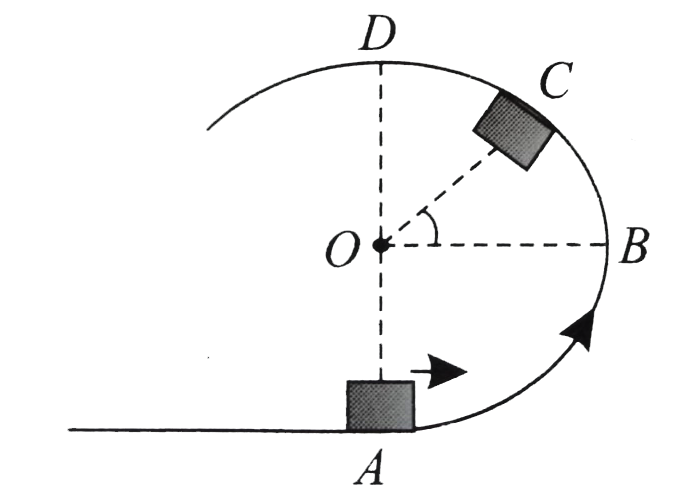
A small block of mass m is pushed on a smooth track from position A with a velocity 2√5 times the minimum velocity required to reach point D. The block will leave the contact with track at the point where normal force between them becomes zero.
Q. When the block reaches the point B, what is the direction (in terms of angle with horizontal) of acceleration of the block?View Solution
Knowledge Check
- Question 1 - Select One
A model rocket rests on a frictionless horizontal surface and is joined by a string of length l to a fixed point so that the rocket moves in a horizontal circular path of radius l. The string will break if its tensiion exceeds a value T. The rocket engine provides a thrust F of constant magnitude along the rocket's direction of motion. the rocket has a mass m that does not change with time. Answer the following questions based on the above passage.
What distance does the rocket travel between the time t1 when the string breaks and the time 2t1? The rocket engine continues of operate after the string breaks.A3lT2FB2lT3FClT2FD2lTF - Question 2 - Select One
A model rocket rests on a frictionless horizontal surface and is joined by a string of length l to a fixed point so that the rocket moves in a horizontal circular path of radius l. The string will break if its tensiion exceeds a value T. The rocket engine provides a thrust F of constant magnitude along the rocket's direction of motion. the rocket has a mass m that does not change with time. Answer the following questions based on the above passage.
Starting from rest at t=0 at what later time t1 is the rocket travelling so fast that the string breaks. Ignnore any air resistance.A(2mlTF2)1/2B(mlTF2)1/2C(mlT2F2)1/2D(mlFT2)1/2 - Question 3 - Select One
A model rocket rests on a frictionless horizontal surface and is joined by a string of length l to a fixed point so that the rocket moves in a horizontal circular path of radius l. The string will break if its tensiion exceeds a value T. The rocket engine provides a thrust F of constant magnitude along the rocket's direction of motion. the rocket has a mass m that does not change with time. Answer the following questions based on the above passage.
What was the magnitude of instantaneous net acceleration at time t1/2? Obtain answer in terms of F,T and m.A[T2+8F2]1/2mB[T+4F2]1/22mC[T2+16F2]1/24mDnone of these
A prototype of a rocket is fired from the ground. The rocket rises vertically up with a uniform acceleration of 54m/s2. 8 second after the start a small nut gets detached from the rocket. Assume that the rocket keeps rising with the constant acceleration.
(a) What is the height of the rocket at the instant the nut lands on the ground
(b) Plot the velocity – time graph for the motion of the nut after it separates from the rocket till it hits the ground. Plot the same velocity– time graph in the reference frame of the rocket. Take vertically upward direction as positive and g=10m/s2View SolutionA point moving with constant acceleration from A to B in the straight line AB has velocities u and v at and B respectively. Find its velocity at C, the mid point of AB. Also show that if the time from A to C is twice that from C to B, then v=7u.
View SolutionA square loop of edge a has N turns and a total resistance R . The loop moves with constant velocity v through a region of constant magnetic field B . The loop enters in magnetic field at t=0. Discuss the vartion of (a) Flux passing through the loop (b) the induced emf and (c) the external force acting on the loop a function of position of the loop in the field.
 View Solution
View Solution
A small block of mass m is pushed on a smooth track from position A with a velocity 2√5 times the minimum velocity required to reach point D. The block will leave the contact with track at the point where normal force between them becomes zero.
Q. When the block reaches the point B, what is the direction (in terms of angle with horizontal) of acceleration of the block?View SolutionA drag racing car starts from rest at t=0 and moves along a straight line with velocity given by v=bt2, where b is a constant. The expression for the distance traveled by this car from its position at t=0 is
View Solution

