The figure shows electronic wave function for a hydrogen atom.
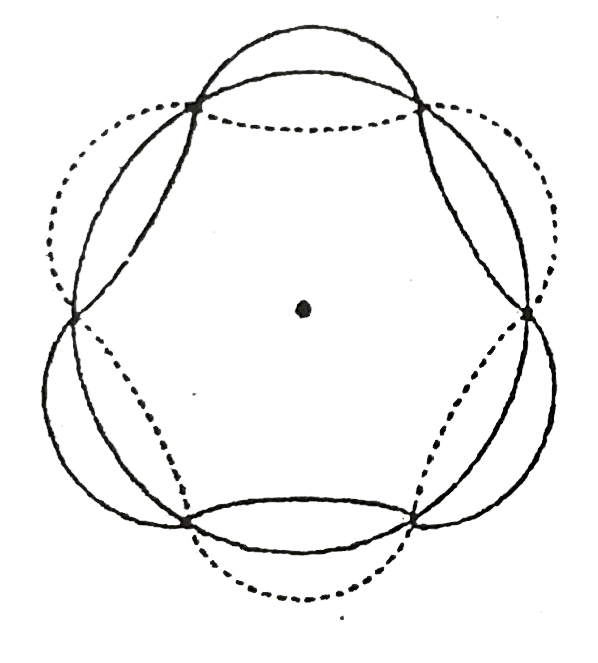
The quantum number of this state is 6
The wavelength of this electron is 6πr0 (r_(0) is radius of ground state)
It can go to ground state by emitting 3 different photons
On deexcitation it emits at least one line in infra red region of spectrum.
The correct Answer is:B, C
(A) n=3
(B) 2πr=nλ⇒2π32r0=nλ⇒λ=6πr0
(C) No =3C2=3
(D) all lines in Balmer or lyman series.
Topper's Solved these Questions
- MASTER PRACTICE PROBLEMBOOK - BANSALCHAPTER - MASTER PRACTICE PROBLEMEXERCISE - Subjective
107 Videos
- MASTER PRACTICE PROBLEMBOOK - BANSALCHAPTER - MASTER PRACTICE PROBLEMEXERCISE - Additional topic
70 Videos
- MASTER PRACTICE PROBLEMBOOK - BANSALCHAPTER - MASTER PRACTICE PROBLEMEXERCISE - Reasoning
75 Videos
- FLUID MECHANICSBOOK - BANSALCHAPTER - FLUID MECHANICSEXERCISE - PYQS AIEEE
10 Videos
- SEMICONDUCTORSBOOK - BANSALCHAPTER - SEMICONDUCTORSEXERCISE - CBSE Question
32 Videos
Similar Questions
Knowledge Check
The wave motion of electron in a Bohr's orbit of hydrogen is as shown in diagram.The potential energy of electron in the given orbit of hydrogen atom is :
 A−3.4eVB+3.4eVC3.02eVD−1.51eV
A−3.4eVB+3.4eVC3.02eVD−1.51eVThe diagram shows the energy level for an electron in a hydrogen atom. Which transition, shown here, represents the emissions of a photon of maximum energy?
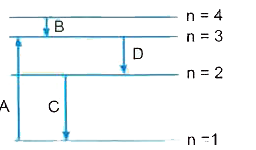 AABBCCDD
AABBCCDDNegative electron gain energy for hydrogen atom means that
Athe energy of an electron in an atom is lower than the energy of free electron at rest which is taken as zero.Bwhen the electron is free from the influence of nucleus, it has a negative value which becomes more negative.Cwhen the electron is attracted by the nucleus , the energy is absorbed which means a negative value.Denergy is released by hydrogen atom in the ground state .
The wave motion of electron in a Bohr's orbit of hydrogen is as shown in diagram.The potential energy of electron in the given orbit of hydrogen atom is :

View SolutionThe wave motion of an electron in a Bohr's orbit of hydrogen atom is an shown in diagram. The orbit number is :
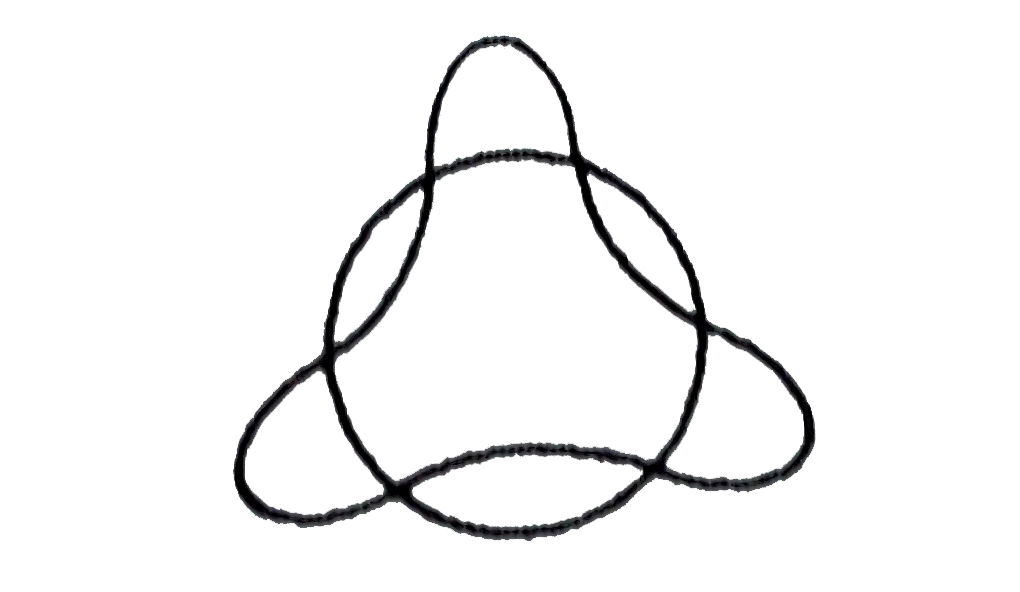
View SolutionThe velocity of an electron in the orbit of hydrogen atom is 5.47×105m/sec. Total number of waves formed by the electron in one complete revolution is
View SolutionThe velocity of an electron in the orbit of hydrogen atom is 5.47×105m/sec. Total number of waves formed by the electron in one complete revolution is
View SolutionA wave function for an electron in an atom is called
View Solution
