A satellite is a circular orbit radius 7000 km around the Earth. If it is transferred to circular orbit of double the radius,
$\begin{matrix} Column I & Column II (A) & Angular momentum & (P) & increase. (B) & Area of Earth covered by satellite signal & (Q) & decreases. (C) & potential energy & (R) & becomes double. (D) & kinetic energy & (S) & becomes half. \end{matrix}$

Video Solution

Text Solution

Verified by Experts

(P) Pressure above surface xx area
$π R^{2} \times ρ g h$
(B) Pressure below bottom xx Area
$= (2 ρ g h + 2 ρ g h) π R^{2} = 4 π R^{2} ρ g h$
(C) $F_{v} = h \int 0 ρ g (h + x) d x \times 2 R = ρ R g^{3} h^{2}$
$F_{r} = h \int 0 ((2 ρ g h) + (2 ρ g x)) 2 R d x$
$= 4 ρ g h \frac{3 h^{2}}{h} \Rightarrow 6 ρ R g h^{2}$
$\to t a l = 9 ρ R g h^{2}$

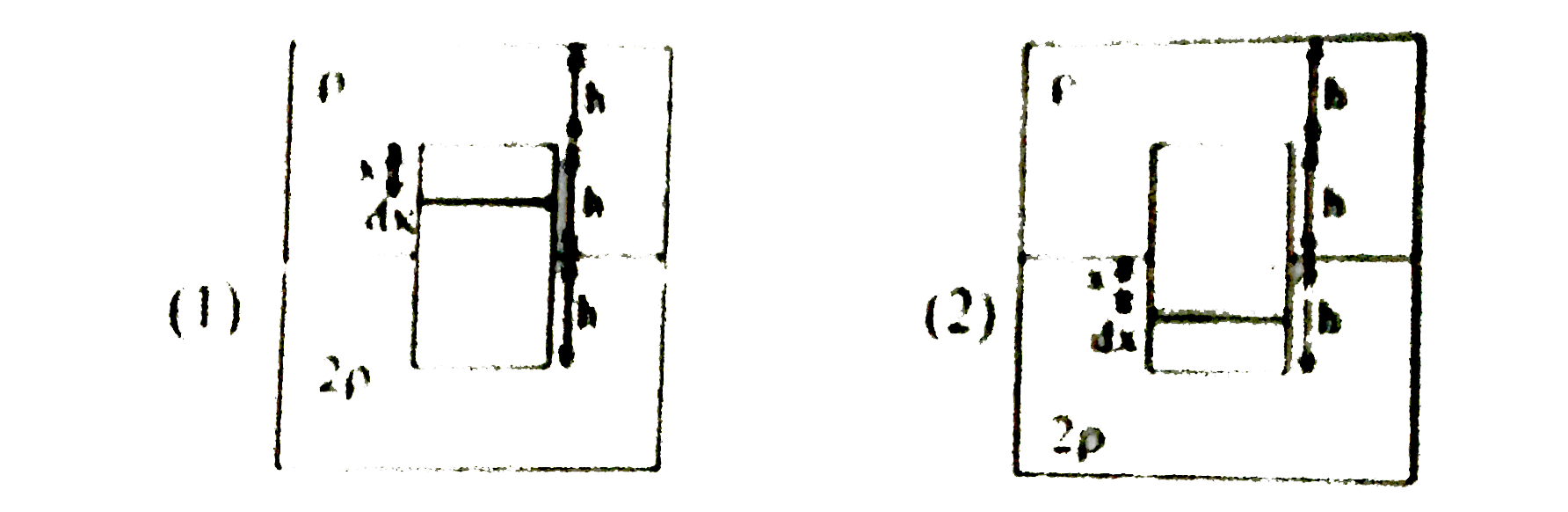
$F_{B u o y a n c y} = ρ α p R^{2} h g + 2 ρ π R^{2} h g$
$= 3 ρ π R^{2} h g$

Updated on:21/07/2023