One mole of an ideal monoatomic gas is taken from state A to state B through the process It is found that its temperature increases by 100 K in this process. Now it is taken from state B to C through a process for which internal energy is related to volume as Find the total work performed by the gas (in Joule) if it is given that volume at B is and at C it is [Use ]
The correct Answer is:
To solve the problem, we need to calculate the total work done by the gas during the two processes: from state A to state B and from state B to state C.
Step 1: Work Done from A to B
The process from state A to state B is defined by the equation P=32T1/2. We know that for an ideal gas, the pressure P can also be expressed as:
P=nRT
where n is the number of moles and R is the universal gas constant. Given that n=1 mole and R=8.3J/mol-K, we can equate the two expressions for pressure:
32T1/2=RT
Step 2: Relate Volume and Temperature
From the ideal gas law, we can express volume V in terms of temperature T:
PV=nRT⟹V=nRTP
Substituting P:
V=nRT32T1/2=2nR3T1/2
Step 3: Differentiate Volume with Respect to Temperature
To find the work done, we need to express dV in terms of dT:
Differentiating V:
dV=2nR3⋅12T−1/2dT=nR3T−1/2dT
Step 4: Substitute into Work Integral
The work done WAB from A to B is given by:
WAB=∫PdV
Substituting P and dV:
WAB=∫32T1/2⋅nR3T−1/2dT=∫nR2dT
Step 5: Evaluate the Integral
The temperature increases by 100K, so we can integrate from TA to TB:
WAB=nR2∫TBTAdT=nR2(TB−TA)=1⋅8.32⋅100=415J
Step 6: Work Done from B to C
Now, we need to find the work done from state B to state C, where the internal energy U is related to volume as:
U=12V1/2
The work done WBC can be calculated using the relationship between internal energy and work:
WBC=ΔU=UC−UB
Step 7: Calculate Internal Energies
At volume VB=100m3:
UB=12(100)1/2=12⋅10=5J
At volume VC=1600m3:
UC=12(1600)1/2=12⋅40=20J
Step 8: Calculate Work Done from B to C
Thus, the work done from B to C is:
WBC=UC−UB=20J−5J=15J
Step 9: Total Work Done
The total work done by the gas is:
Wtotal=WAB+WBC=415J+15J=430J
Final Answer
The total work performed by the gas is:
430J
Topper's Solved these Questions
- BOOK - BANSALCHAPTER - MASTER PRACTICE PROBLEMEXERCISE - Additional topic70videos
MASTER PRACTICE PROBLEM
- BOOK - BANSALCHAPTER - MASTER PRACTICE PROBLEMEXERCISE - Multiple objective131videos
MASTER PRACTICE PROBLEM
- BOOK - BANSALCHAPTER - FLUID MECHANICSEXERCISE - PYQS AIEEE10videos
FLUID MECHANICS
- BOOK - BANSALCHAPTER - SEMICONDUCTORSEXERCISE - CBSE Question32videos
SEMICONDUCTORS
Similar Questions
One mole of an ideal monoatomic gas is taken from state A to state B through the process P=32T1/2 It is found that its temperature increases by 100 K in this process. Now it is taken from state B to C through a process for which internal energy is related to volume as U=12V1/2 Find the total work performed by the gas (in Joule) if it is given that volume at B is 100m3 and at C it is 1600m3 [Use R=8.3J/mol−K]
View SolutionI mole of an ideal monoatomic gas is expanded till the temperature of the gas is doubled under the process V2T=contant. The initial temperature of the gas is 400K. In terms of R find total work done in the process.
View Solution
Knowledge Check
- Question 1 - Select One
One mole of an ideal monoatomic gas is taken through a polytropic process P2T = constant. The heat required to increase the temperature of the gas by ΔT is
ARΔTB3RΔTC5RΔTD6RΔT - Question 2 - Select One
One mole of an ideal monoatomic gas is taken through the thermodynamic process shown in the P−V diagram. The heat supplied to the system is
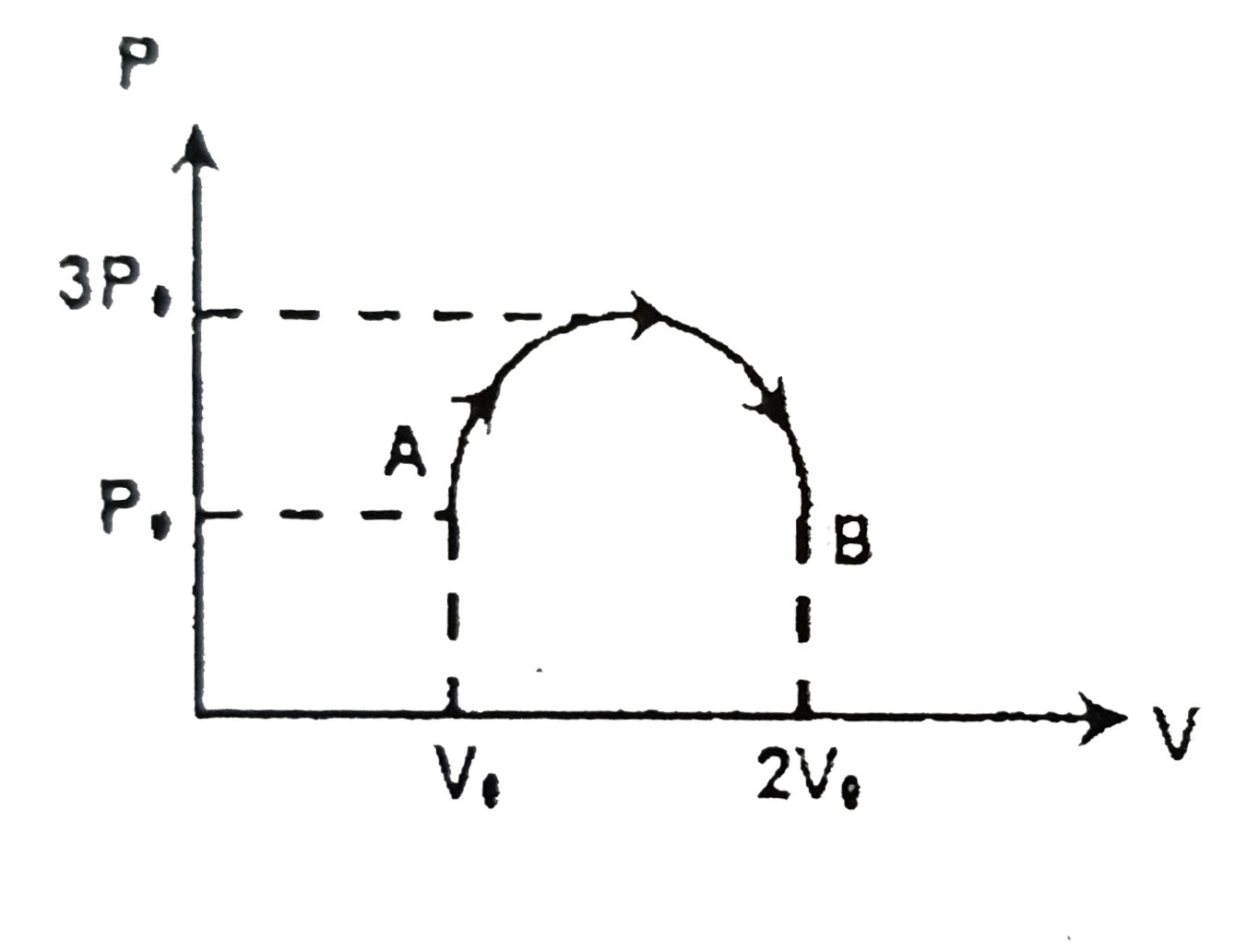 AP0V0(3+π2)BP0V0(2π−12)CP0V0(1+π)DP0V0(5+π2)
AP0V0(3+π2)BP0V0(2π−12)CP0V0(1+π)DP0V0(5+π2) - Question 3 - Select One
One mole of an ideal gas is taken from state A to state B by three different processes (a) ACB, (b) ADB and (c ) AEB as shown in the P−V diagram. The heat absorbed by the gas is
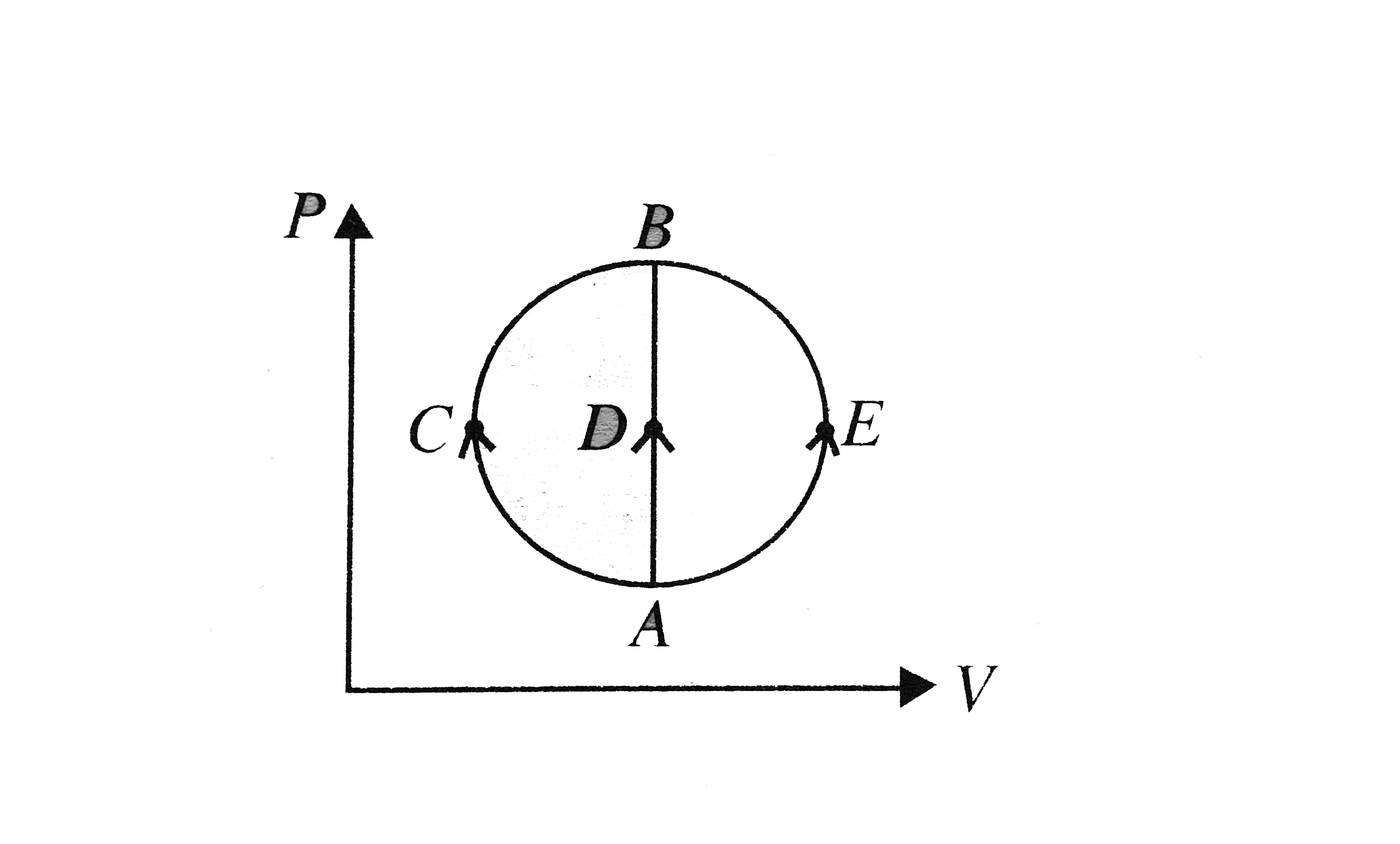 Agreater in process (ii) than in (i)Bthe least in process (ii)Cthe same in (i) and (iii)Dless in (iii) than in (ii)
Agreater in process (ii) than in (i)Bthe least in process (ii)Cthe same in (i) and (iii)Dless in (iii) than in (ii)
One mole of an ideal gas going from state-A to state-B through different processes. Column-I shows graph of the process and column-II shows change in parameter in these process. Match the following:
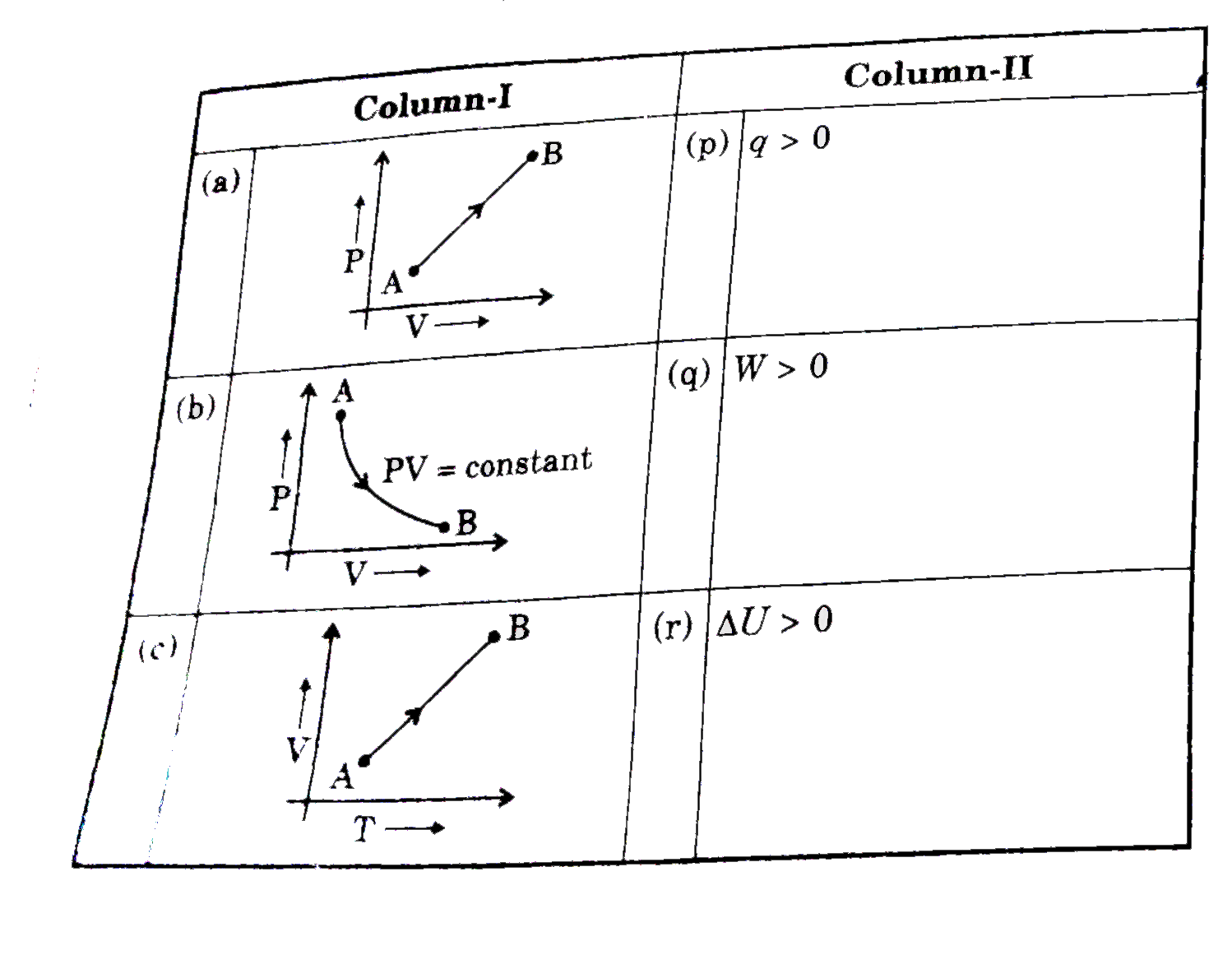 View Solution
View SolutionOne mole of an ideal monoatomic gas is taken at a temperature of 300K. Its volume is doubled keeping its pressure constant. Find the change in internal energy.
View SolutionOne mole of an ideal gas is taken from state A to state B by three different processes (a) ACB, (b) ADB and (c ) AEB as shown in the P−V diagram. The heat absorbed by the gas is
 View Solution
View SolutionOne mole of an ideal monoatomic gas under goes a process V=aT2. The temperature of the gas increases by 100K. Which of the following is incorrect ?
View SolutionOne mole of an ideal diatomic gas is taken through a process whose P−V diagram is shown in the figure. The work done by the gas is
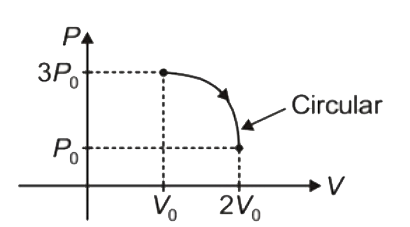 View Solution
View Solution

