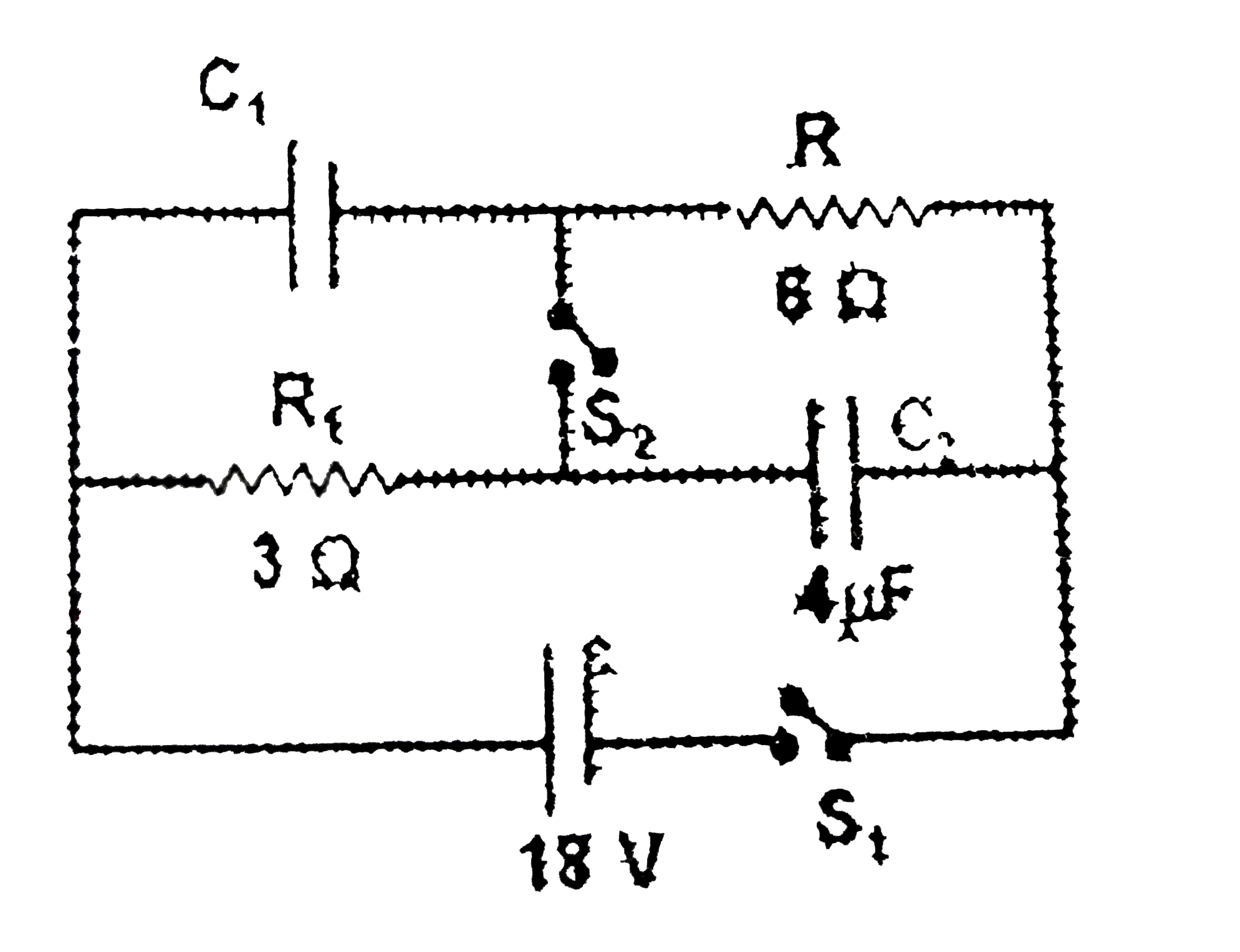
Consider the shown network. Initially both swiches are open and the capacitor are uncharged. Both the switches are then closed simultaneously at t=0
(a) Obtain the current through switch S2 as function of time.
(b) If switch S2 is opened again after a long time interval, find the total heat that would dissipate in the resistor and the charge that would flow through switch S1 after S2 is opened?
Consider the charges on capacitors and currents through various branches, as shown in the figure
For loop 1, we have
R1(I2+I3−I1)=q1C1 (1)
For loop2, R2i3=q2C1 (2)
For the outer loop, i3R2+q1C1=ε (3)
Also, i1=dq1dt and i2=dq2dt (4)
Putting the values of i1 and i2 from Eq. (4) and of i3 from Eq. (2) in (1), we get
ddt(q2−q1)=q1R1C1−q2R2−C2 (5)
From Eqs. (2) and (3), we get
q2C2+q1C1=ε (6)
From Eqs. (5) and (6), we get
∫q20dq2(εC2R2R1+R2)−q2=∫t0dtReq(C1+C2), where Req=R1R2R1+R2
⇒q2=εR2C2(R1+R2)[1−e1Req(C1+C2)]
Similarly, q1=εR1C1(R1+R2)[1−e1Req(C1+C2)]
⇒i1=dq1dt=εC1R2(C1+C2)e1Req(C1+C2)
Similarly, i2=εC2R1(C1+C2)e−1Req(C1+C2)
From equation (1)
Current through S2=(i1−i3)=i2−q1R1C1
Putting the values, we get
q1=(12μC)(1−e112μ), q2=(48μC)(1−e112μ)
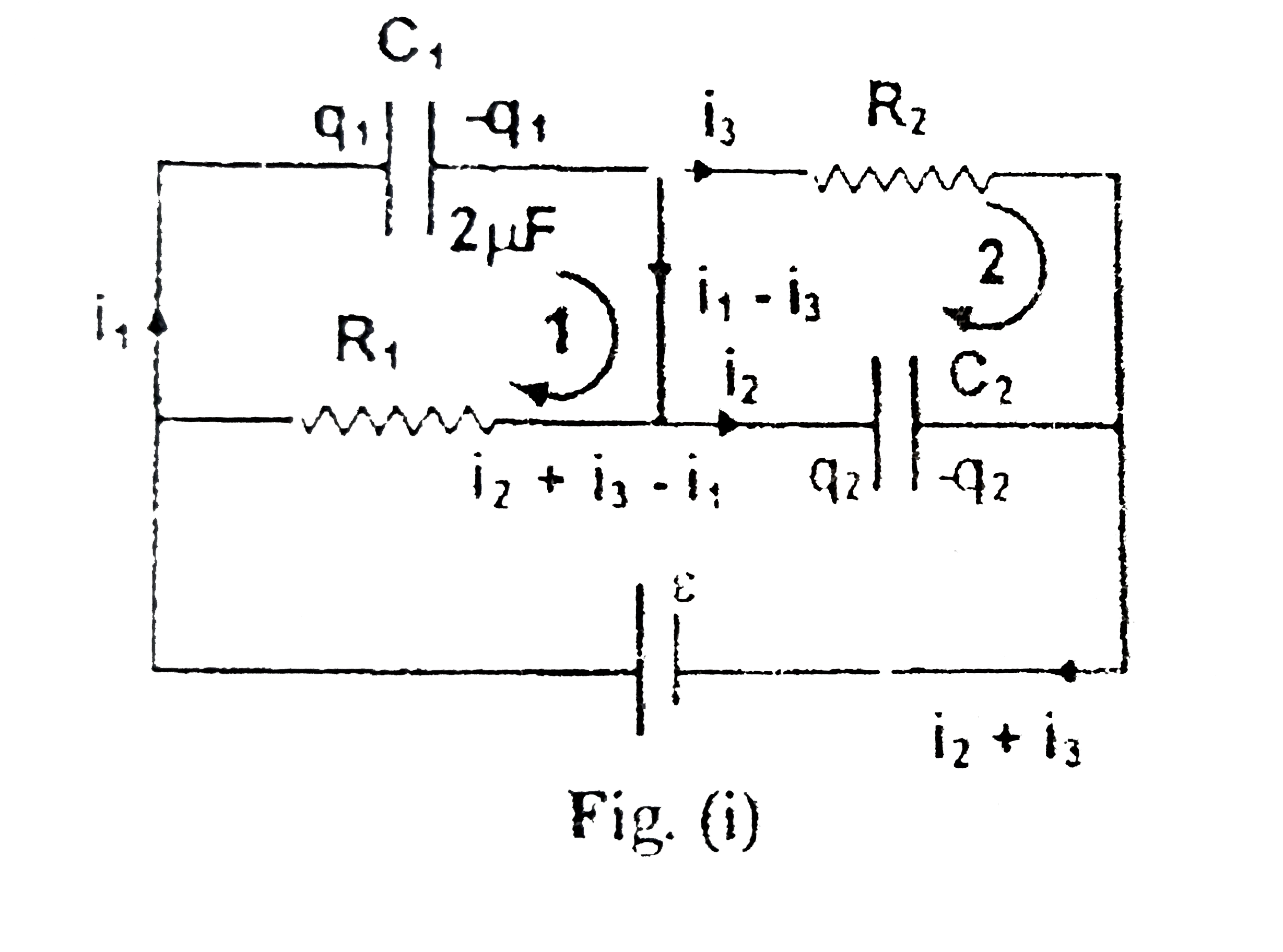
i1=(1A)e112μ, i2=(4A)e112μ
Current through switch s2=−[2−e112μ]A along the indicated direction as shown in fig (i),
With both the switches closed the steady state charges and currents are as shown in Fig (ii).
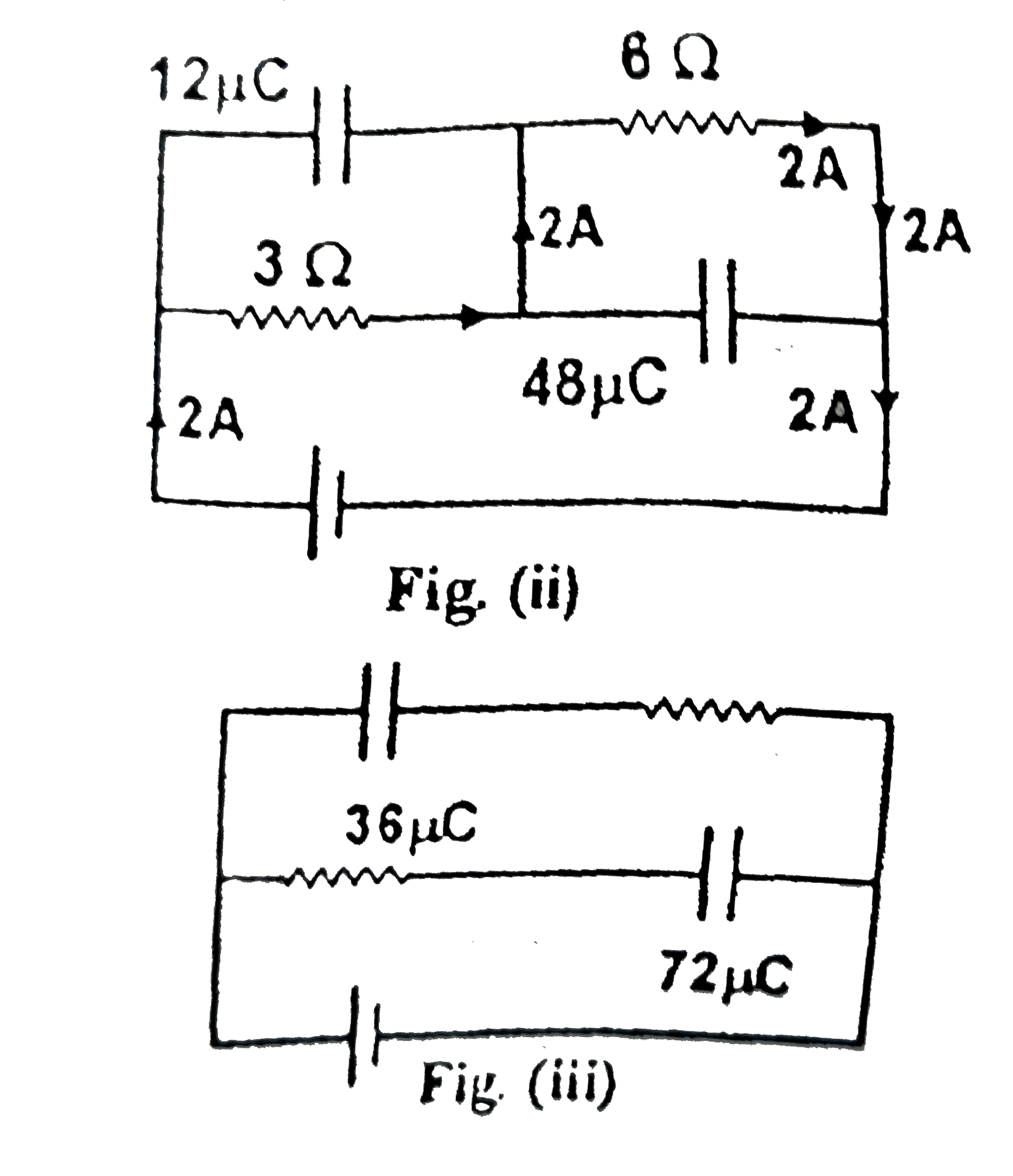
With switch S2 open and s1 closed, the steady state charges are as shown in Fig.(iii).
Hence, the charge flown through switch S1=[(36+72)−(12+48)]μC=48μC.
Total heat dissipated in the resistors = [Initial energy + work done by battery when 48μC flows through it after switch S2 is opened ] − [final energy]
={12C1V21+12C2V22}+ε(ΔQ)−{12C1V'21+12C2V'22}=136μJ.
Topper's Solved these Questions
- CURRENT ELECTRICITYBOOK - FIITJEECHAPTER - CURRENT ELECTRICITYEXERCISE - Illustration
10 Videos
- CURRENT ELECTRICITYBOOK - FIITJEECHAPTER - CURRENT ELECTRICITYEXERCISE - SOLVED PROBLEMS (SUBJECTIVE)
10 Videos
- COLLISION BOOK - FIITJEECHAPTER - COLLISION EXERCISE - (NUMERICAL BASED QUESTIONS)
4 Videos
- ELASTICITY AND WAVES BOOK - FIITJEECHAPTER - ELASTICITY AND WAVES EXERCISE - Assignment Problems (Objective) Level-II
15 Videos
Similar Questions
Knowledge Check
Find heat produced in the capacitors after long time on closing the switch S
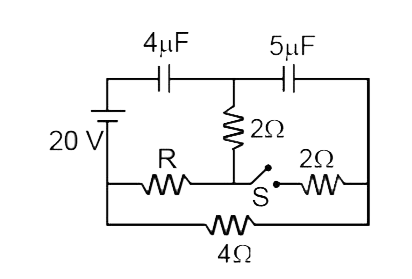 A0.0002JB.0005JC0.00075JDzero
A0.0002JB.0005JC0.00075JDzeroThe circuit was in the shown state from a long time. Now the switch S is closed. The charged that flows through the switch is
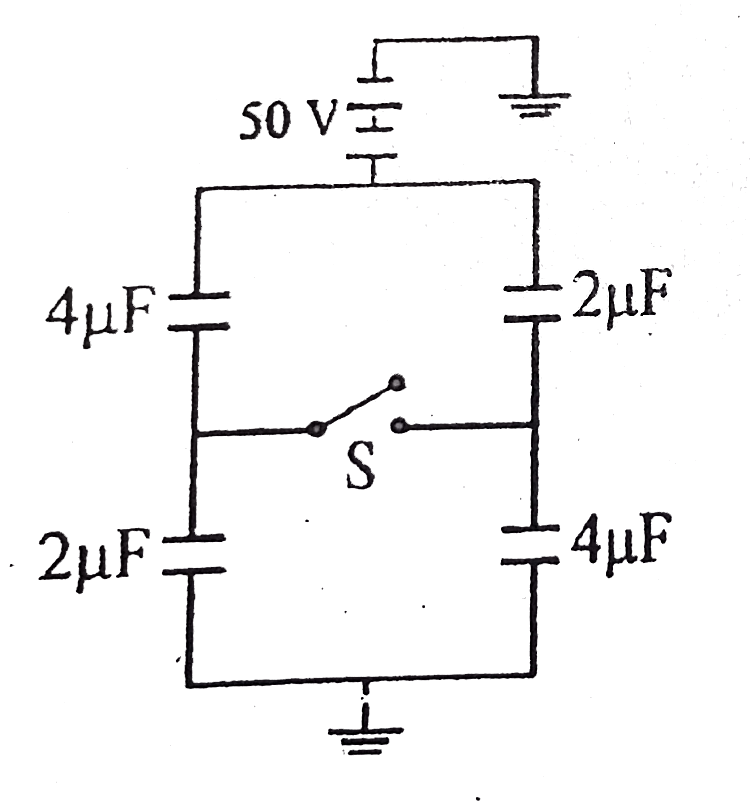 A4003μCB100μCC50μCD1003μC
A4003μCB100μCC50μCD1003μCThe circuit was in the shown state for a long time. Now if the switch S is closed then the net charge that flows through the switch S, will be
 A4003μCB100μCC1003μCD50μC
A4003μCB100μCC1003μCD50μC
Determine the current through the battery in the circuit shown in figure.
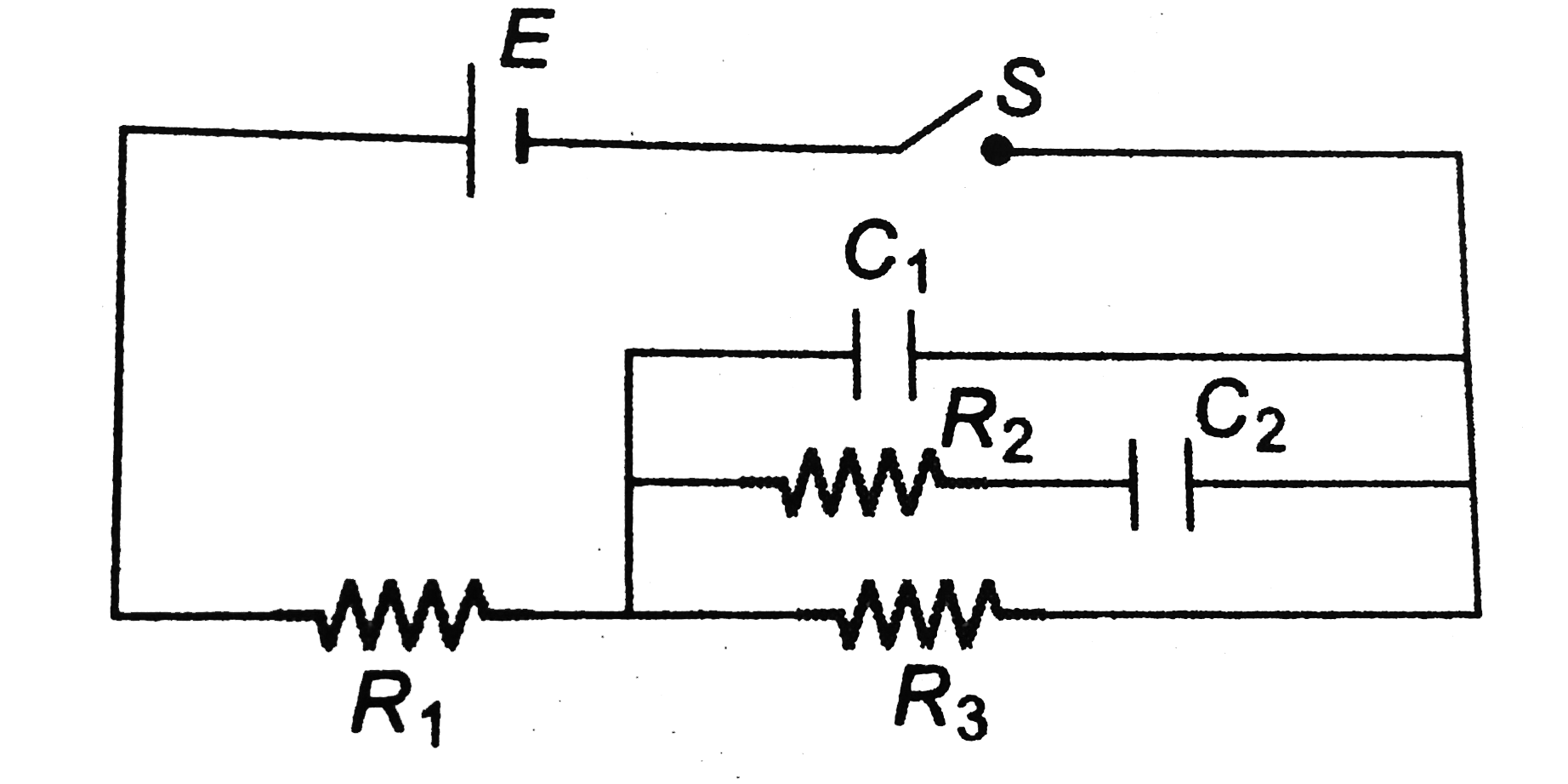
(a) immediately after the switch S is closed
(b) after a long time.
View SolutionDetermine the current through the battery in the circuit shown in figure.

(a) immediately after the switch S is closed
(b) after a long time.
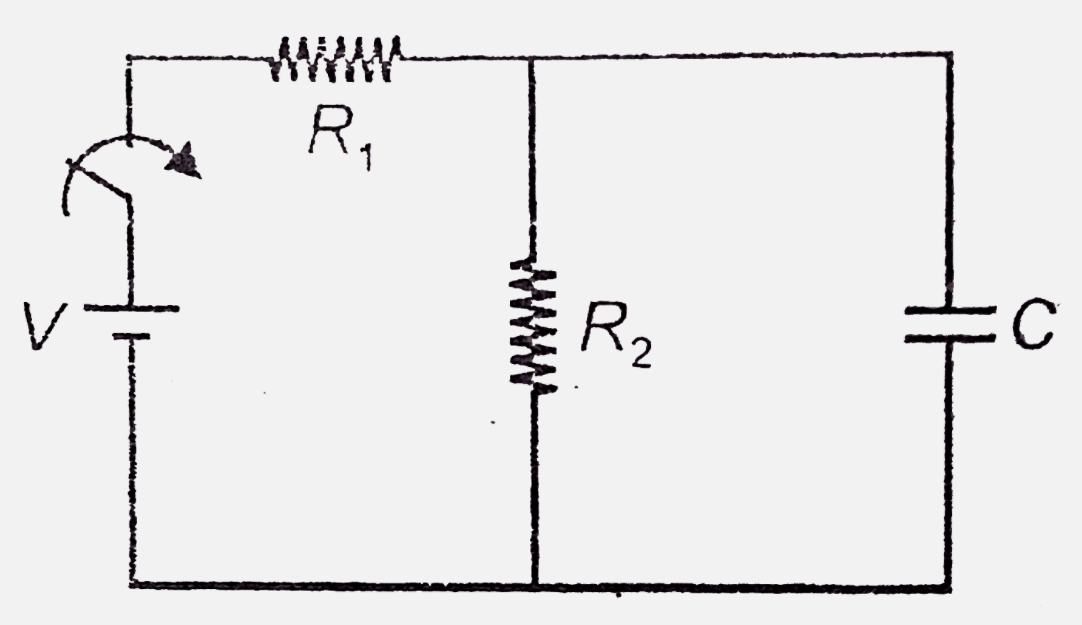
View SolutionConsider the circuit shown. Switch is closed at t=0. Find the current through the battery as a function of time. Initially capacitor was uncharged.
View SolutionIn the circuit shown, the switch S has been close for a long time. At time t=0 the switch is opened. It remains open for a long time T, after which it is closed again.

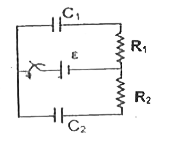
View SolutionIf the capacitors are uncharged prior to the switch being closed, the amount of heat dissipated in resistors R1 and R2 after the switch is closed would be in the ratio of
View Solution

