A source emitting sound of frequency 165Hz is placed in frount of a wall at a distance of 2m from it. A detector is also placed in fornt of the wall at the same distance from it. Find the distance between the source and the detector for which the detector detects phase difference of 2π between the direct and reflected wave. Speed of sound in air =330m/s.
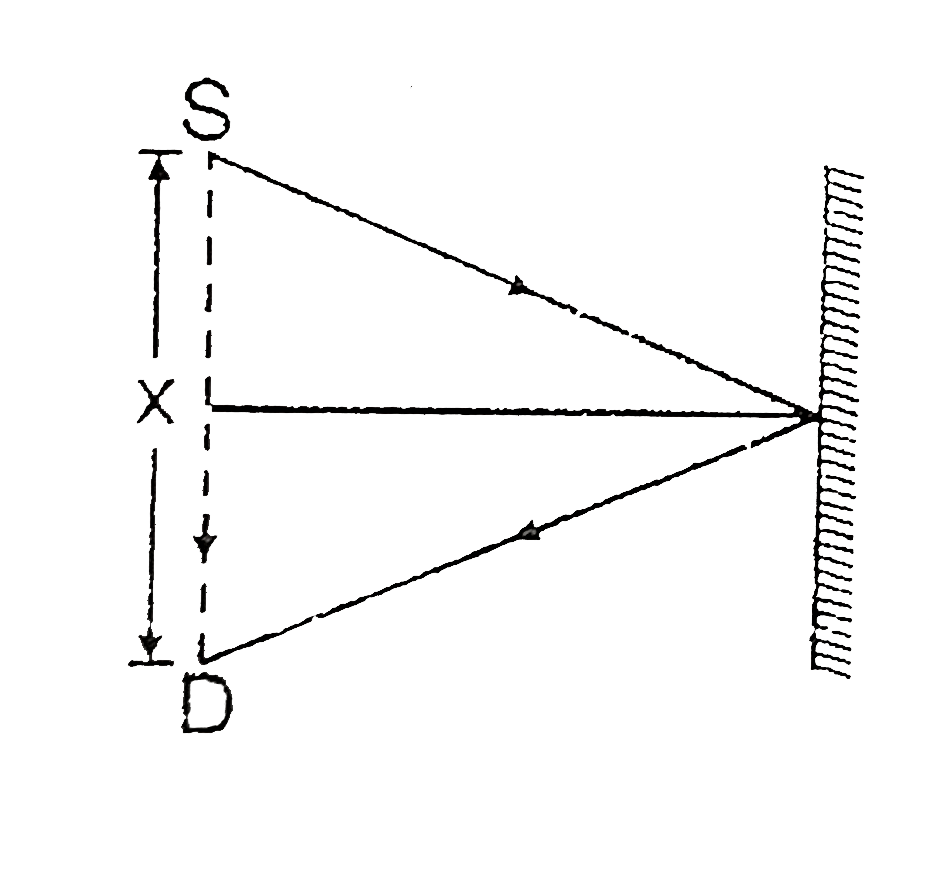
The situation is shown in figure. Suppose the detector is placed at a distance of x meter from the source. The direct wave received from the source travels a distance of x meter. The wave reaching the detector after reflection from the wall has travelled a distance of 2[(2)2+x2/4]1/2 meter. The path difference between the two waves is
Δ={2[(2)2+x24]1/2−x}meter.
Δ=λ for Δϕ=2π...(i)
Thus, by (i)2[(12)2+x24]1/2−x=2
or, [4×x22] or, 4+x24=1+x24+x
or, x=3
Thus, the dector should be placed at a distance of 3m from the source, Note that there is no abrupt phase change.
Topper's Solved these Questions
- BOOK - RESONANCECHAPTER - SOUND WAVESEXERCISE - Board Level Exercise33videos
SOUND WAVES
- BOOK - RESONANCECHAPTER - SOUND WAVESEXERCISE - Exercise- 1 PART - I34videos
SOUND WAVES
- BOOK - RESONANCECHAPTER - SOUND WAVESEXERCISE - Exercise- 3 PART - II1videos
SOUND WAVES
- BOOK - RESONANCECHAPTER - SIMPLE HARMONIC MOTIONEXERCISE - Exercise28videos
SIMPLE HARMONIC MOTION
- BOOK - RESONANCECHAPTER - STRING WAVESEXERCISE - Exercise32videos
STRING WAVES
Similar Questions
(a) A source of sound S and a detector D are placed at some distance from one another. A big cardboard is placed near the detector and perpendicular to the line SD as shown in figure. It is gradually moved away and it is found that the intensity changes from a maximum to a minimum as the board is moved through a distance of 20cm. Find the frequency of the sound emitted. Velocity of sound in are is 336m/s.

(b) A source emitting sound of frequency 180Hz is placed in front of a wall at a distance of 2m from it. A detector is also placed in front of the wall at the same distance from it. find the minimum distance between the source and the detector for which the detector detects a maximum of sound . Speed of sound in air =360m/s.View SolutionA source emitting sound of frequency 180 Hz is alced in front of a wall at a distance of 2 m from it. A detector is also placed in front of the weal at the same distance from it. Find the minimum distance between the source and the detector for which the detector detects a maximum of sound. Speed of sound in air =360ms−1.
 View Solution
View Solution
Knowledge Check
- Question 1 - Select One
A source emitting sound of frequency 180Hz is placed in front of a wall at distance of 2m from it . A detector is also placed in front of the wall at the same distance from it. Find the minimum distance between the source and the detector for which the detector detects a maximum of sound. Speed of sound in air =360m/s.
A3mB2mC3/2mDzero - Question 2 - Select One
A soure of sound of frequency 165 hz is placed in front of a wall at a distance 2 m from it. A dtector is also placed in front of the wall at the same distance from it. Find the minimum distance between the source and detector for which maximum sound is recorded int he detector . the speed of sound is 330 m/s
A4mB3 mC1 mD2m - Question 3 - Select One
When a sound wave is reflected from a wall the phase difference between the reflected and incident pressure wave is:
A0BpCp/2Dp/4
A source emitting sound of frequency 100 Hz . It is placed in front of a wall at a distance of 2m from it. Also, if a detector is also placed in front of the wall at the same distance from it, then the minimum distance between the source and the detector for which detector detects a maximum of sound is
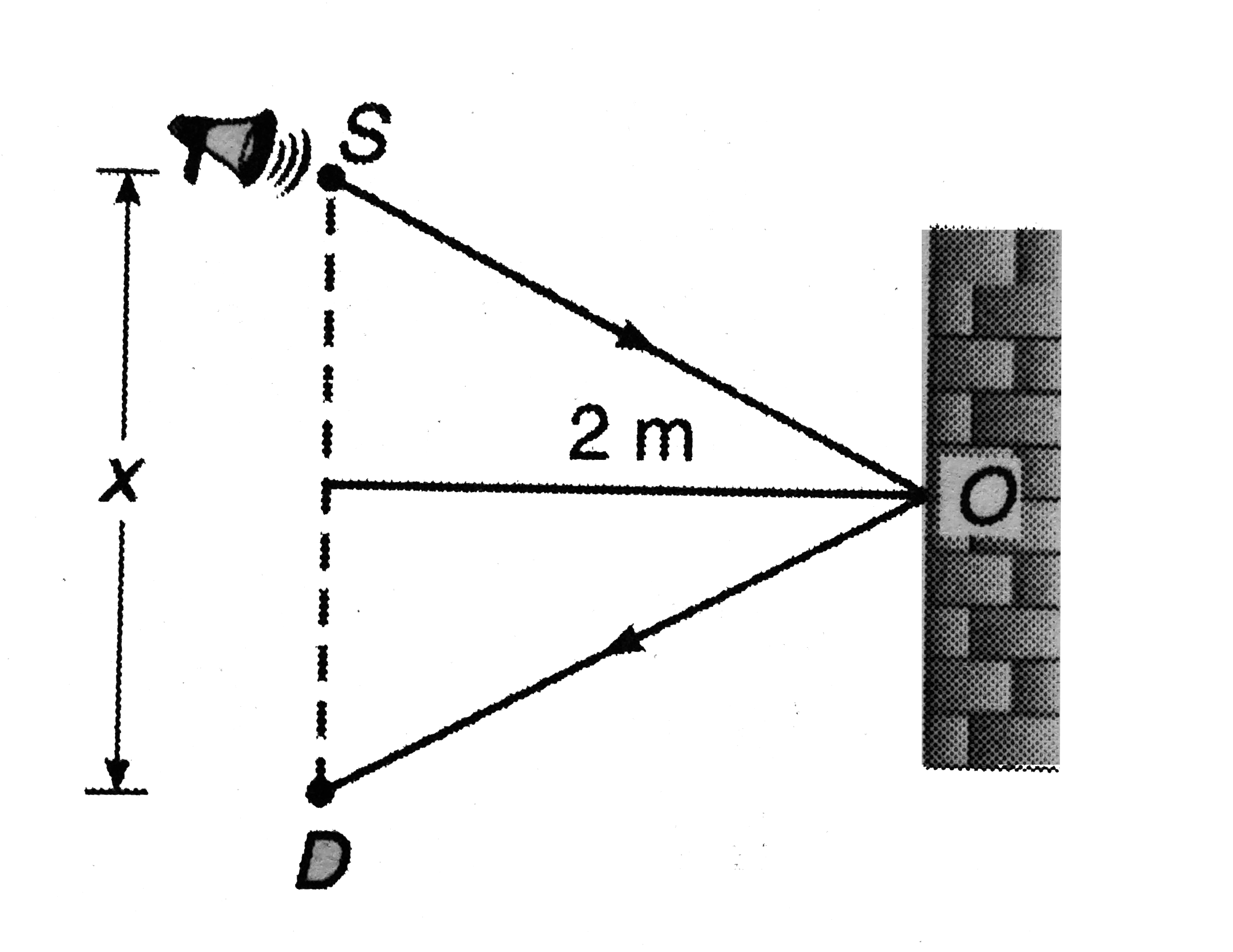
________ m(vsound=360m/s)View SolutionA source of sound emitting waves at 360HZ is placed in front of a vertical wall, at a distance 2m from it. A detector is also placed in front of the wall at the same distance from it. Find the minimum distance between the source and the detestor for which the detector detects a maximum of sound. Take speed of sound in air=360m/s. Assume that there is not phase change in reflected wave.
 View Solution
View SolutionA source of sound emitting a 1200 Hz note travels along a straight line at a speed of 170ms−1. A detector is placed at a distance of 200 m from the line of motion of the source. (a) Find the frequency of sound received by the detector at the instant when the source gets closest to it. (b) Find the distance between the source and the detector at the instant it detects the frequency 1200 Hz. Velocity of sound in air=340ms−1.
View SolutionWhen a sound wave is reflected from a wall the phase difference between the reflected and incident pressure wave is:
View SolutionAn echo is returned in 3s. What is the distance of the reflecting surface from the source, given that the speed of sound is 342m/s.
View Solution

