When 1m long metallic wire is stressed, an extension of 0.02m is produced. An organ pipe 0.5m long and open at both ends, when sounded with this stressed metallic wire, produced 8 beats in its fundamental mode. By decreasing the stress in the wire, the number of beats are found to decrease. Find the Young's modulus of the wire. The density of metallic wire is 104kg/m3 and velocity of sound in air is 292m/s.
The correct Answer is:Y=1.76×1011N/m2
The fundamental frequency of open pipe is n=v2l=2922×.5=292Hz
Let L be the initial length and L+ΔL
the stressed length and L+Δ=.98+.02=1m
The fundamental frequency of stressed wire n=12(L+ΔL)√Tπr2d
where r is the radius and d is the density of wire.
The stressed wire and the organ pipe (frequency 292Hz) produces 8 beats
∴n=292±8
By decreasesing tension velocity decreased At the same time number of beats also decreases.
∴n=292+8=300
by eqn. we have Tπr2=4n2d(L+ΔL)2
Y=Tπr2ΔLL=4n2d(L+ΔL)2(ΔLL)=4×(300)2×104×12(.02.98)=17.64×1010N/m2
Topper's Solved these Questions
- BOOK - RESONANCECHAPTER - SOUND WAVESEXERCISE - Exercise- 3 PART - II1videos
SOUND WAVES
- BOOK - RESONANCECHAPTER - SOUND WAVESEXERCISE - Exercise- 2 PART - IV8videos
SOUND WAVES
- BOOK - RESONANCECHAPTER - SIMPLE HARMONIC MOTIONEXERCISE - Exercise28videos
SIMPLE HARMONIC MOTION
- BOOK - RESONANCECHAPTER - STRING WAVESEXERCISE - Exercise32videos
STRING WAVES
Similar Questions
When 0.98m long metallic wire is stressed, an extension of 0.02 m is produced. An organ pipe 0.5 m long and open at both ends, when sounded with this stressed metallic wire, produces 8 beats in its fundamental mode. By decreasing the stress in the wire, the number of beats are found to decrease. Find the young's modulus of wire. The density of metallic wire is 104kg/m3 and sound velocity in the air is 292 m/s.
View Solution(a) A wire 4m long and 0.3 mm, calculate the potential energy stored in the wire.
Young's modulus for the material of wire is 2.0×1011N/m2.View Solution
Knowledge Check
- Question 1 - Select One
If stress is 1012 times the strain produced in a wire, then its Young's modulus will be
A1012 unitsB10−12 unitsC1024 unitsD10−24 units - Question 2 - Select One
What will be the stress required to double the length of a wire of Young's modulus Y?
AYB2YC3YD4Y - Question 3 - Select One
A steel wire 0.5 m long has a total mass of 0.02 kg and is stretched with a tension of 800N. The frequency when it vibrates in its fundamental mode is
A2HzB141HzC100HzD200Hz
Which one of the following is the Young's modulus (in N/m) for the wire having the stress-strain curve shown in the figure
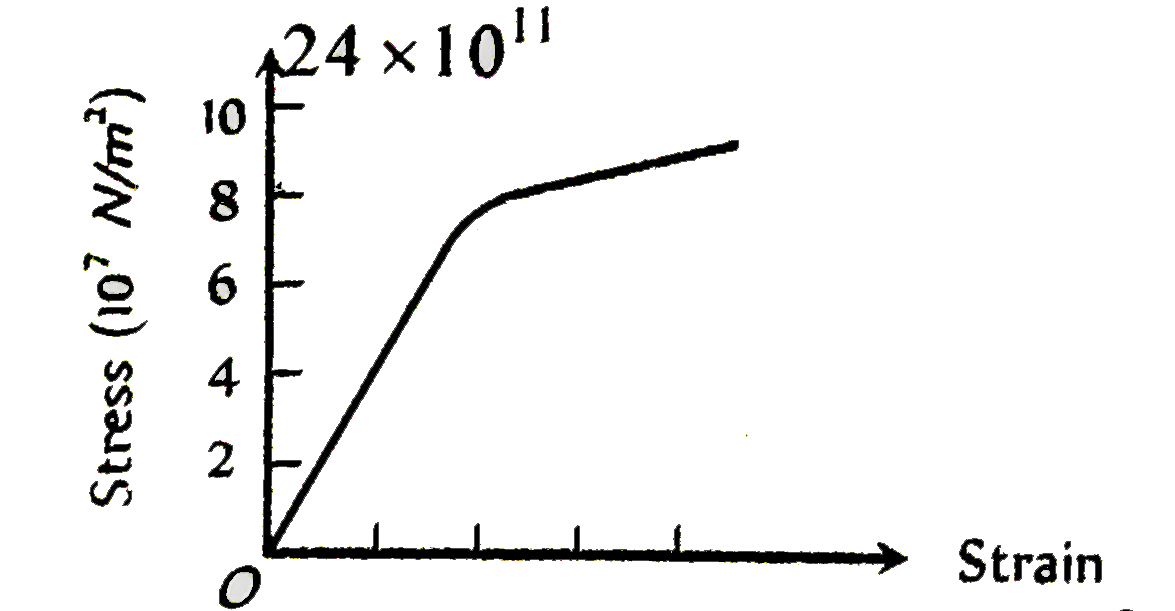 View Solution
View SolutionA sonometer wire of length 0.95 m can bear a maximum stress of 9×108N/m2. Calculate the fundamental frequency produced in the wire if density of wire is 8×103kg/m3.
View SolutionA tuning fork of frequency 250 Hz produces a beat frequency of 10 Hz when sounded with a sonometer vibrating in its fundamental frequency. When the tuning fork is filed, the beat frequency decreases. If the length of sonometer wire is 0.5 m, the speed of transverse wave is
View SolutionIf the stress in a wire is (1/200)th of Young's modulus, then the strain produced will be
View SolutionA load of 3kg produces an extension of 1.5mm in a wire of length 3m and diameter 2mm. Young's modulus of the material of the wire is
View Solution

