Two transverse sine waves, each of amplitudes 4mm wavelength 2m and time period 1s and in phase at x=0,t=0 are travelling along the x−axis in opposite direction. Obtain the equation of the resultant wave and comment on its nature calculate the maximum displacement at x=2.333m. Also locate the antinodes and nodes.
The correct Answer is:y1+y2=4×10−3[sin2π(t−x2)+sin2π(t+x2)]
At t=0 and x=0,y1+y2=0
At x=2.333,y1+y2=4×10−3m
Attinodes at x=1,2
Nodes at x=12,13
y1=4×10−3sin2π(t−x2)
y2=4×103sin2π(t+x2)
y1+y2=4×10−3[sin2π(t−x2)+sin2π(t+x2)]
=8×10−3cosπxt
At t=0, and x=0,y1+y2=0
At x=2.333,y1+y2=8×10−3cos0.333π
=4×10−3m
Antinodes at x=12,32
Topper's Solved these Questions
- BOOK - RESONANCECHAPTER - SOUND WAVESEXERCISE - Exercise- 3 PART - II1videos
SOUND WAVES
- BOOK - RESONANCECHAPTER - SOUND WAVESEXERCISE - Exercise- 2 PART - IV8videos
SOUND WAVES
- BOOK - RESONANCECHAPTER - SIMPLE HARMONIC MOTIONEXERCISE - Exercise28videos
SIMPLE HARMONIC MOTION
- BOOK - RESONANCECHAPTER - STRING WAVESEXERCISE - Exercise32videos
STRING WAVES
Similar Questions
A transverse wave of amplitude 2 m, wavelength 4 m and frequency 6 Hz is propagating in a string toward positive y-direction. The equation of the wave may be (y is in m and t is in s)
View SolutionThe equation of simple harmonic progressive wave, having amplitude 5×10−2m, wavelength 30 cm, speed 50 m/s and travelling along the positive direction of the x-axis is given by
View Solution
Knowledge Check
- Question 1 - Select One
A transverse wave of amplitude 0.5m and wavelength 1 m and frequency 2 Hz is propagating in a string in the negative x - direction. The expression for this wave is [
Ay(x,t)=0.5sin(2πx−4πt)By(x,t)=0.5cos(2πx+4πt)Cy(x,t)=0.5sin(πx+2πt)Dy(x,t)=0.5cos(2πx+2πt) - Question 2 - Select One
A transverse wave, whose amplitude is 0.5 m wavelength is 1 m and frequency is 2Hz. is travelling along positive x direction. The equation of this wave will be-
Ay=0.5cos(2πx−4πt)By=0.5cos(2πx−4πt)Cy=0.5sin(πx−2πt)Dy=0.5cos(2πx−2πt) - Question 3 - Select One
A transverse wave of amplitude 0.5m and wavelength 1 m and frequency 2 Hz is propagating in a string in the negative x - direction. The expression for this wave is
Ay(x,t)=0.5sin(2πx−4πt)By(x,t)=0.5cos(2πx+4πt)Cy(x,t)=0.5sin(πx−2πt)Dy(x,t)=0.5cos(2πx+2πt)
The equation of a simple harmonic progressive wave having amplitude 5 mm, period 2 second and wavelength 2m and travelling along the positive direction of the x-axis is given by
View Solutionin a sine wave ,positive of different particles at time t=0 is shown in figure. The equation for this wave if it is travelling along positive x -axis can be
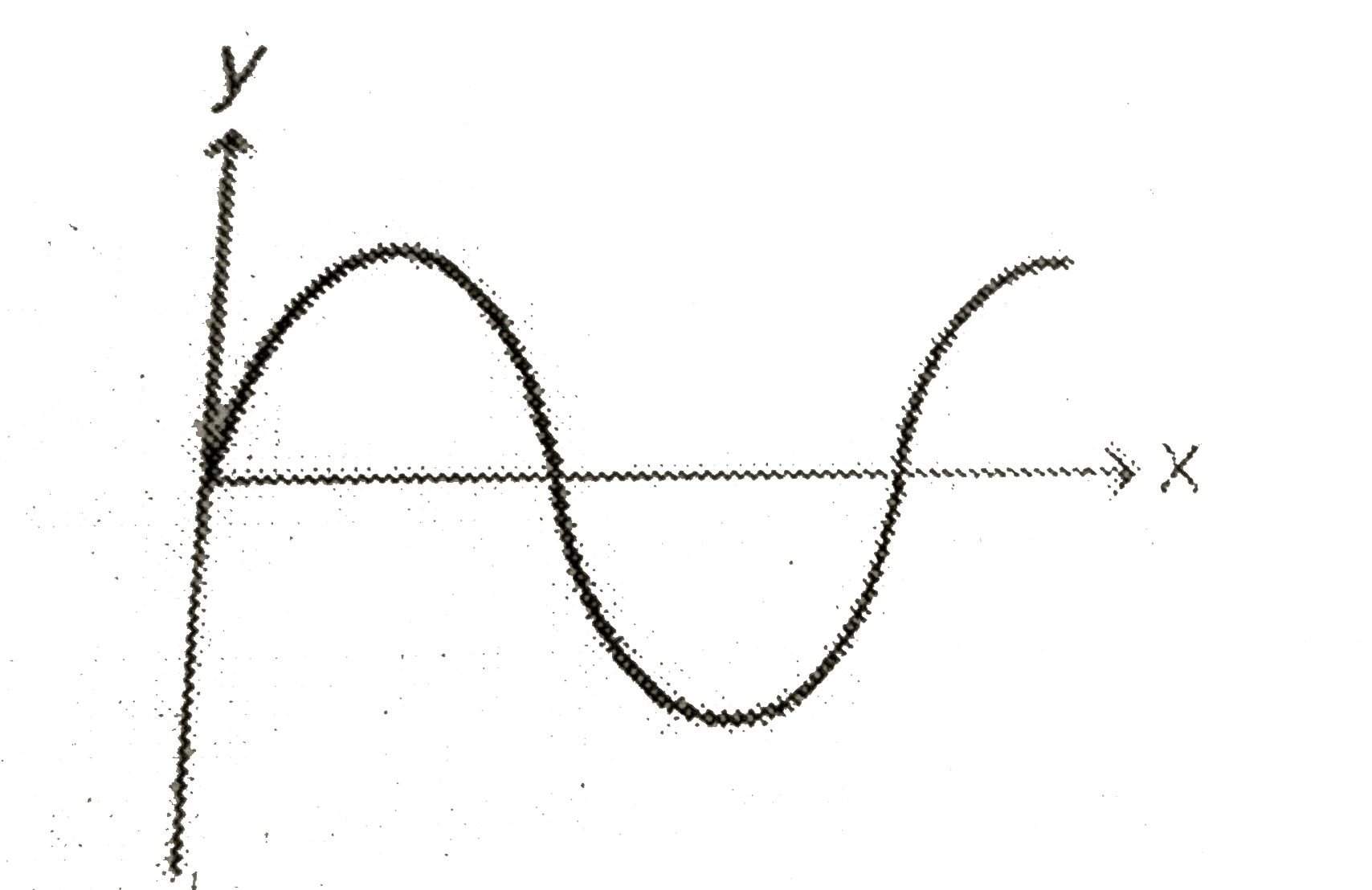 View Solution
View SolutionTwo sound waves each of frequency 500Hz are travelling along the same line in opposite direction . If the speed of sound in air is 350 m/s. find the distance between the consecutive antinodes of the resulting stationary wave.
View SolutionA progressive wave having amplitude 5m and wavelength 3m. If the maximum average velocity of particle in half time period is 5m/s and waves is moving in the positive x-direction then find which may be the correct equation (s) of the wave ? [wave x in meter].
View SolutionA wave travelling along the x-axis is described by the equation y (x, t) = 0.005 sin (αx−βt). If the wavelength and time period of the wave are 0.08 m and 2 s respectively, then α,β in appropriate units are
View Solution

