A certain volume of a gas (diatomic) expands isothermally at 20∘C until its volume is doubled and then adiabatically until its volume is again doubled. Find the final temperature of the gas, given g=1.4 and that there is 0.1mo≤ of the gas. Also calculate the work done in the two coses R=8.3Jmo≤−1K−1
The correct Answer is:1.4×102J
We require T-V relation to calculate the final temperature.
We haveTVγ−1=constant/(273+20)Vg−1=(273+t)(2V)γ−1
or 273+t=29321.4−1=293(2)0.4
log(273+t)=log293−0.4log2−0.4log2=log293−0.4×0.3010
=2.4669−0.1204
or log(273+t)=2.3465
or 273+t=antilog2.3465
or 273+t=222.1
t=−50.9∘C
(i) Work done in isothermal process
=nRTloge(V2)v1=8.310×293loge(2V)V∴(n=110)
=0.83×293×2.3log210(∵logex=2.3log10x)
=0.83×293×2.3×0.3010=1.684×102J
(ii) Work done in adiabatic processs=nR(T−T')γ−1=0.83293−222.11.4−1
=0.83×70.90.4=1.47×102J
Topper's Solved these Questions
- BOOK - BANSALCHAPTER - KINETIC THEORY OF GASESEXERCISE - Exercise-435videos
KINETIC THEORY OF GASES
- BOOK - BANSALCHAPTER - KINETIC THEORY OF GASESEXERCISE - Section-B13videos
KINETIC THEORY OF GASES
- BOOK - BANSALCHAPTER - KINETIC THEORY OF GASESEXERCISE - Matrix Type2videos
KINETIC THEORY OF GASES
- BOOK - BANSALCHAPTER - HEAT TRANSFEREXERCISE - Exercise89videos
HEAT TRANSFER
- BOOK - BANSALCHAPTER - UNIT DIMENSION, VECTOR & BASIC MATHSEXERCISE - Exercise10videos
UNIT DIMENSION, VECTOR & BASIC MATHS
Similar Questions
A certain volume of a gas (diatomic) expands isothermally at 20∘C until its volume is doubled and then adiabatically until its volume is again doubled. Find the final temperature of the gas, given γ= 1.4 and that there is 0.1 mole of the gas. Also calculate the work done in the two cases. R=8.3Jmole−1K−1
View SolutionA certain volume of a gas (diatomic) expandds isothermally at 20∘C until its volume is doubled and then adiabatically until its volume is again doubled. Find the final temperature of the gas, given γ=1.4 and that there is 0.1 mole of the gas. Also calculate the work done in the two cases. R=8.3Jmole−1K−1
View SolutionA gass of given mass at a pressure of 105Nm−2 expands isothermally until its volume is doubled and then adiabatically until volume is again double. Find the final pressure of the gas. (γ=1.4)
View SolutionA litre of hydrogen at 127∘C and 106dy≠cm−2 pressure expands isothermally until its volume is doubled and then adiabatically until its volume is redoubled. Find the final pressure of the gas. Given γ=1.4.
View SolutionOne mole of perfect gas, initially at a pressure and temperture of 105Nm−2 and 300 K, respectively, expands isothermally until its volume is doubled and then adiabatically until its volume is again doubled. Find the final pressure of the gas. Given γ=1.4.
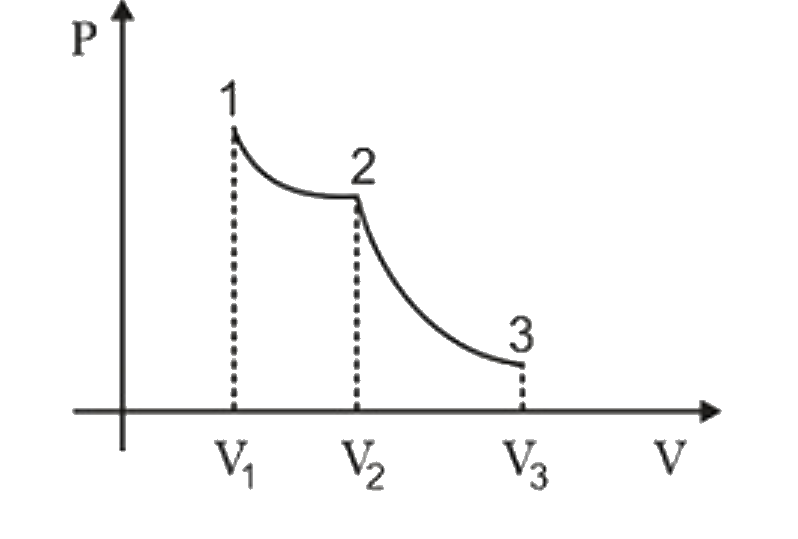 View Solution
View SolutionA gram molecule of a gas at 127∘C expands isothermally until its volume is doubled. Find the amount of work done and heat absorbed.
View SolutionA volume of gas at 15∘C expands adiabatically until its volume is doubled. Find the resultant temperture given that the ratio of specific heat capacity of the gas =1.4.
View SolutionOne mole of a perfect gas, initally at a pressure and temperature of 105N/m2 and 300 K, respectively, expands isothermally unitl its volume is doubled and then adiabatically until its volume is again doubled. Find final pressure and temperature of the gas Find the total work done during the isothermal and adiabatic processes. Given γ=1.4. Also draw the P-V diagram for the process.
 View Solution
View SolutionOne mole of a perfect gas, initally at a pressure and temperature of 105N/m2 and 300 K, respectively, expands isothermally unitl its volume is doubled and then adiabatically until its volume is again doubled. Find final pressure and temperature of the gas Find the total work done during the isothermal and adiabatic processes. Given γ=1.4. Also draw the P-V diagram for the process.
 View Solution
View SolutionA gram mole of a gas at 27∘C expands isothermally until its volume is doubled. Calculate the amount of work done. (R=8Jmol−1K−1)
View Solution
