Component of −10ˆj in the direction of 3ˆi−4ˆj is :-
8
2
-4
1
The correct Answer is:A
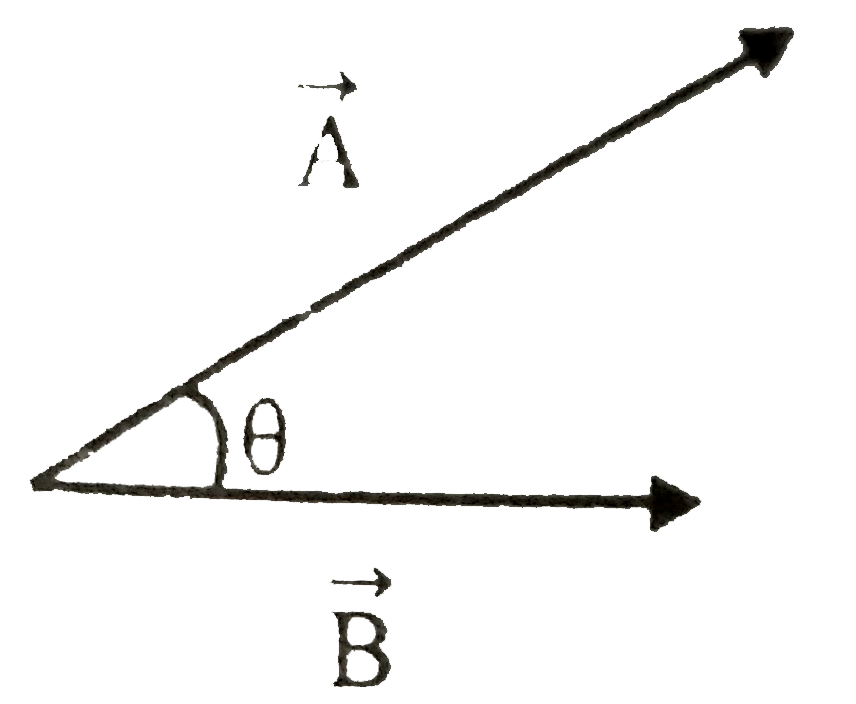
→A.→B=ABcosθ
→A.→BB=Acosθ
(Acosθ)=(−10ˆj).(3ˆi−4ˆj)√32+42
=+405=8
Topper's Solved these Questions
- BOOK - BANSALCHAPTER - UNIT DIMENSION, VECTOR & BASIC MATHSEXERCISE - EXERCISE - 2 [MULTIPLE CORRECT CHOICE TYPE]6videos
UNIT DIMENSION, VECTOR & BASIC MATHS
- BOOK - BANSALCHAPTER - UNIT DIMENSION, VECTOR & BASIC MATHSEXERCISE - EXERCISE - 2 [MATRIX TYPE]1videos
UNIT DIMENSION, VECTOR & BASIC MATHS
- BOOK - BANSALCHAPTER - UNIT DIMENSION, VECTOR & BASIC MATHSEXERCISE - EXERCISE- 3 (SECTION - B)1videos
UNIT DIMENSION, VECTOR & BASIC MATHS
- BOOK - BANSALCHAPTER - KINETIC THEORY OF GASESEXERCISE - Section-B13videos
KINETIC THEORY OF GASES
Similar Questions
Knowledge Check
- Question 1 - Select One
The component of vector A=2ˆi+3ˆj along the vector ˆi+ˆj is
A5√2B10√2C5√2D5 - Question 2 - Select One
What is the component of 3ˆi+4ˆjalongˆi+ˆj:
A12(ˆi+ˆj)B32(ˆi+ˆj)C52(ˆi+ˆj)D72(ˆi+ˆj) - Question 3 - Select One
IF →A=5ˆi+12ˆj and →B=3ˆi+4ˆj, find component of →A in the direction of →B.
A635(5ˆi+12ˆj)B3225(3ˆi+4ˆj)C6325(3ˆi+4ˆj)D6325(3ˆi−4ˆj)
Statement 1: A component of vector →b=4ˆi+2ˆj+3ˆk in the direction perpendicular to the direction of vector →a=ˆi+ˆj+ˆkisˆi−ˆj. Statement 2: A component of vector in the direction of →a=ˆi+ˆj+ˆk is 2ˆi+2ˆj+2ˆk.
View SolutionFind the components of vector →a=3ˆi+4ˆj along the direction of vectors ˆi+ˆj & ˆi−ˆj
View Solutionˆi and ˆj are unit vectors along x-and y-axes respectively. What is the magnitude and the direction of the vectors ˆi+ˆj and ˆi−ˆj? What are the components of a vector →A=2ˆi+3ˆj along the direction ˆi+ˆjand ˆi−ˆj?
View SolutionFind the component of a vector →A=3ˆI+4ˆj along the direction of 2ˆI−3ˆj.
View SolutionFind the component of a vectro →A=3ˆI+2ˆj along the direction of (ˆI+ˆj)
View Solution

