A charged particle of charge q and mass m enters perpendiculalry in a magnetic field B. Kinetic energy of particle E, then frequency of rotation is
qBmπ
qB2πm
qBE2πm
qB2πE
The correct Answer is:b
To find the frequency of rotation of a charged particle with charge q and mass m entering perpendicularly into a magnetic field B, we can follow these steps:
Step 1: Determine the Magnetic Force
When a charged particle moves in a magnetic field, it experiences a magnetic force given by:
F=q(v×B)
Since the particle enters the magnetic field perpendicularly, the magnitude of this force simplifies to:
F=qvB
Step 2: Relate Magnetic Force to Centripetal Force
As the charged particle moves in a circular path due to the magnetic force, this force acts as the centripetal force required to keep the particle in circular motion. The centripetal force is given by:
Fc=mv2r
where r is the radius of the circular path.
Step 3: Set the Forces Equal
Setting the magnetic force equal to the centripetal force, we have:
qvB=mv2r
Step 4: Solve for Velocity
Rearranging the equation to solve for v:
qvB=mv2r⟹qB=mvr⟹v=qBrm
Step 5: Determine Angular Velocity
The angular velocity ω is related to the linear velocity v and the radius r by the equation:
v=rω
Substituting the expression for v:
qBrm=rω
Dividing both sides by r (assuming r≠0):
ω=qBm
Step 6: Calculate Frequency
The frequency f of rotation is related to the angular velocity ω by:
ω=2πf
Substituting for ω:
2πf=qBm
Now, solving for f:
f=qB2πm
Final Answer
Thus, the frequency of rotation of the charged particle is:
f=qB2πm
---
When a charged particle moves in a magnetic field, it experiences a magnetic force given by:
F=q(v×B)
Since the particle enters the magnetic field perpendicularly, the magnitude of this force simplifies to:
F=qvB
As the charged particle moves in a circular path due to the magnetic force, this force acts as the centripetal force required to keep the particle in circular motion. The centripetal force is given by:
Fc=mv2r
where r is the radius of the circular path.
Setting the magnetic force equal to the centripetal force, we have:
qvB=mv2r
Rearranging the equation to solve for v:
qvB=mv2r⟹qB=mvr⟹v=qBrm
The angular velocity ω is related to the linear velocity v and the radius r by the equation:
v=rω
Substituting the expression for v:
qBrm=rω
Dividing both sides by r (assuming r≠0):
ω=qBm
The frequency f of rotation is related to the angular velocity ω by:
ω=2πf
Substituting for ω:
2πf=qBm
Now, solving for f:
f=qB2πm
Thus, the frequency of rotation of the charged particle is:
f=qB2πm
Topper's Solved these Questions
Similar Questions
A charge q , and mass m , is fired perpendicular to a magnetic field (B) with a velocity v . The frequency of revolution of the charge is
View SolutionFour charged particles are projected perpendicularly into the magnetic field with equal velocity. Which will have minimum frequency of rotation?
View Solution
Knowledge Check
A particle of charge q and mass m, is fired perpenducular to a magnetic field (B) with a velocity v. What is the frequency of revolution of the particle?
A2πBqB2πBqmC2πmBqDBq2πmIf a charged particle enters perpendicular in the uniform magnetic field then
AEnergy remains constant but moment changesBEnergy and momentum both remains constantCMomentum remains constant but energy changesDNeither energy nor momentum remains constantA charged particle enters a uniform magnetic field perpendicular to it. The magnetic field
Aincreases the K.E. of the particleBdecreases the K.E. of the particleCchanges the direction of motion of the particleDdoes both (a) and ( c)
A charged particle of charge q and mass m is shot into a uniform magnetic field of induction B at an angle θ with the field. The frequency of revolution of the particle
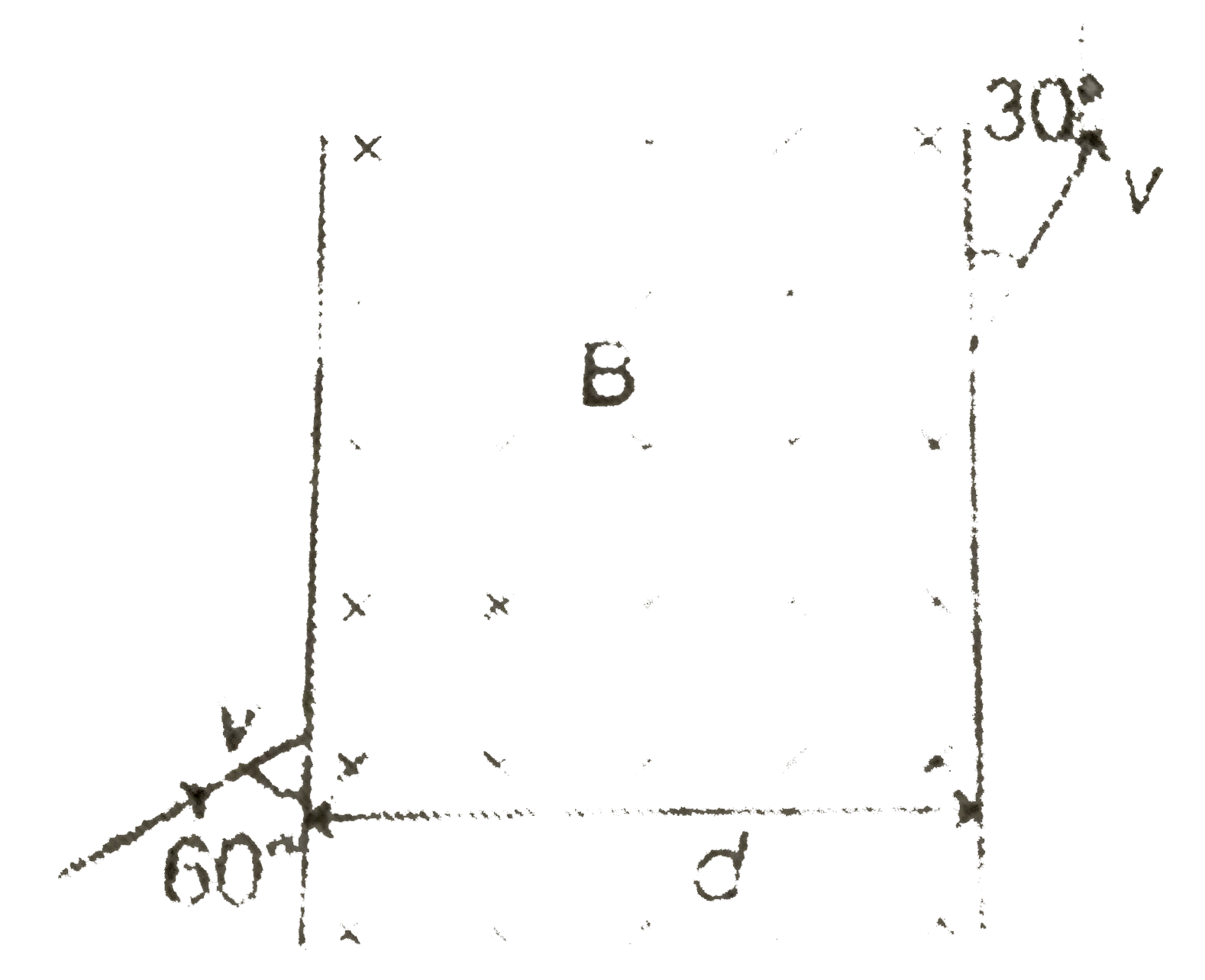
View SolutionA charge particle of charge 'q' and mass 'm' enters in a given magnetic field 'B' and perpendicular to the magnetic field as shown in the figure. It enters at an angle of 60∘ with the boundary surface of magnetic field and comes out at an angle of 30∘ with the boundary surface of the magnetic field as shown in the figure. Find width 'd' in which magnetic field exist.
View SolutionIf a charge particle of charge q , mass m , moves in a circular path in magnetic field of induction B , under a potential of V volts then radius of circular path is
View SolutionWhen a charged particle enters a uniform magnetic field its kinetic energy
View SolutionWhen a charged particle enters a uniform magnetic field its kinetic energy
View Solution
