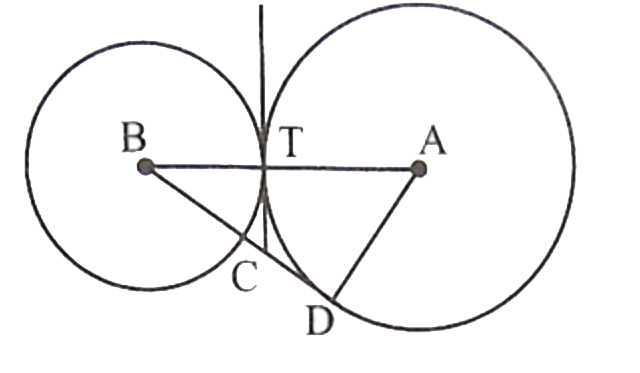
Two circles with unequal radii,centred at A and B respectively,touch each other externally at T.If BD is tangent to the first circle at D and TC is transverse common tangent meeting BD at C.Also,AT has the length 3 and BT has length 2. Then the length of CD is equal to
2
52
32
12
The correct Answer is:C

BT=2 , AT=3=AD
∴ AB=5 , AD=3
∴ BD=4
From triangle BTC: cosθ=BTBC=2BC….(1)
From triangle BAD : cosθ=BDBA
cosθ=45 ….(2)
From (1) and (2)
2BC=45⇒BC=52
∴ CD=BD-BC
=4−52
=32
Topper's Solved these Questions
Similar Questions
Two circles with centres at A and B touch each other externally at T. Let BD is the tangent at D and TC is a common tangent. If AT has length 3 units and BT has length 2 units, then the length (in units ) of CD is
View SolutionTwo circles with centres at A and B, touch at T. BD is the tangent at D and TC is a common tangent. AT has length 3 and BT has length 2. The length of CD is:
View Solution
Knowledge Check
Two circles with centres at A and B touch each other externally at T. Let BD is the tangent at D and TC is a common tangent. If AT has length 3 units and BT has length 2 units, then the length (in units ) of CB is
 A43B52C53D74
A43B52C53D74Two circles with radii 25 cm and 9 cm touch each other externally. Find the length of the direct common tangent.
A32 cmB50 cmC40 cmD30 cmTwo circles of radii 8 cm and 6 cm touch each other externally. The length of the direct common tangent is:
8 सेमी तथा 6 सेमी त्रिज्याओं वाले दो वृत्त एक-दूसरे को बाहर से स्पर्श करते हैं | प्रत्यक्ष उभयनिष्ठ स्पर्श रेखा की लंबाई कितनी है ?A20B24C13.86D10.12
Two circles with radii a and b respectively touch each other externally . Let c be the radius of a circle that touches these two circles as well as a common tangent to the circles. Then
View SolutionTwo circles with radii 25 cm and 9 cm touch each other externally. The length of the direct common tangent is
View SolutionTwo circles with radii a and b touch each other externally such that θ is the angle between their direct common tangent(a>b≥2), then
View SolutionTwo circles touch each other internally. Show that the tangents drawn to the two circles from any point on the common tangent, are equal in length.
View SolutionTwo circles of radii 8 cm and 2 cm respectively touch each other externally at the point A. PQ is the direct common tangent of those two circles of centres O1 and O2 respectively. Then length of PQ is equal to
View Solution
