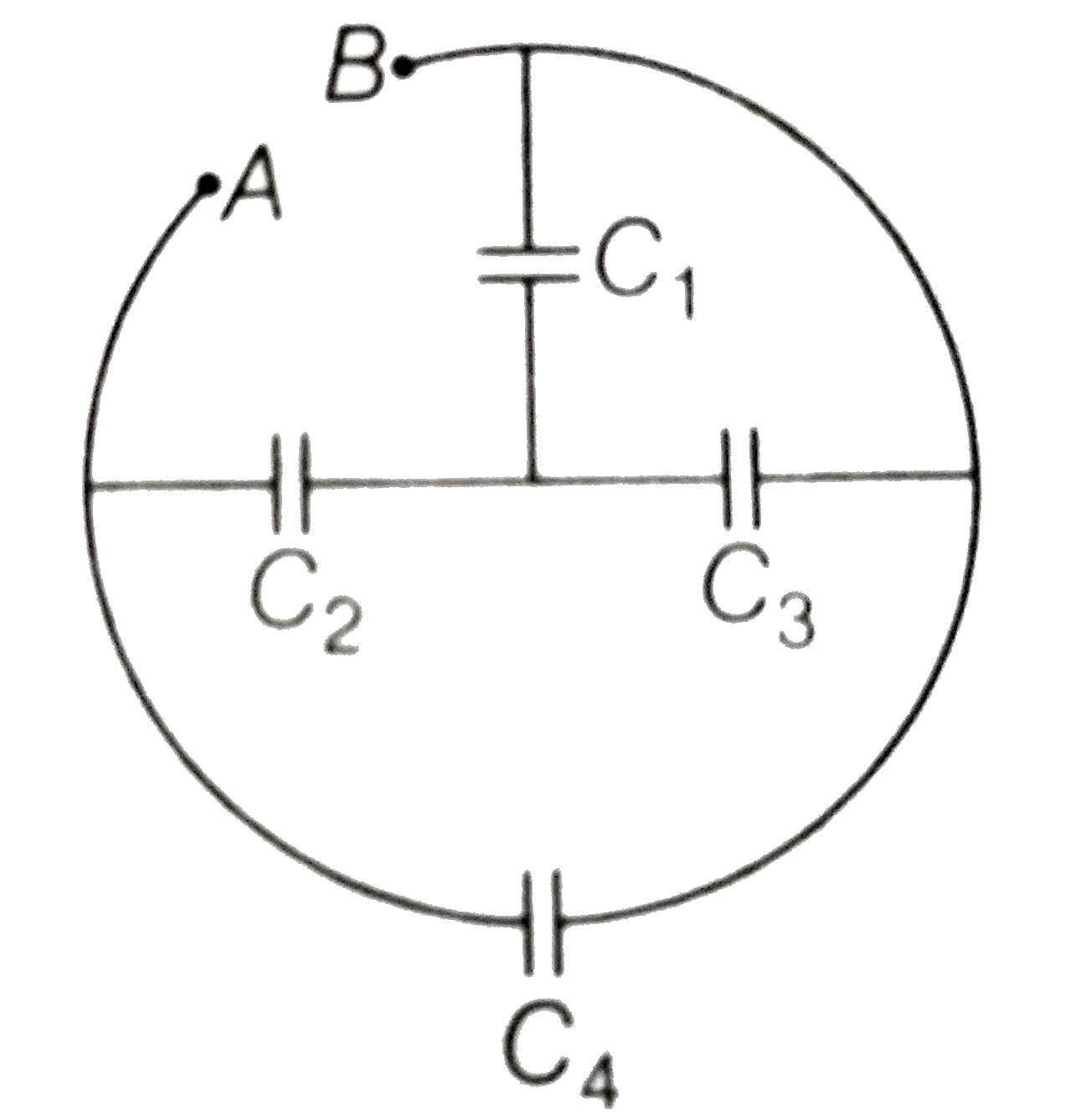
Five capacitors have been arranged in a circuit. Capacitance of each capacitor is C. Determine the effective capacitance between the points A and B.

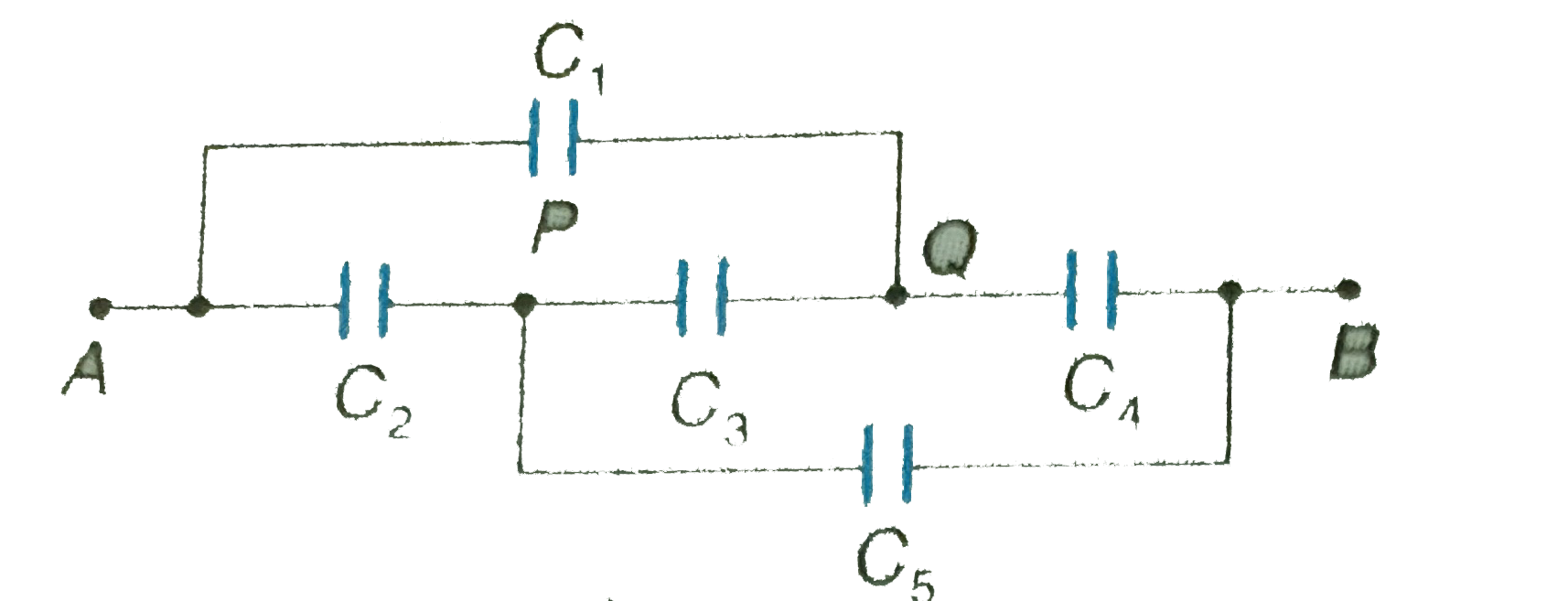
Text Solution

Verified by Experts

Equivalent circuit is like that of Fig. 4.25

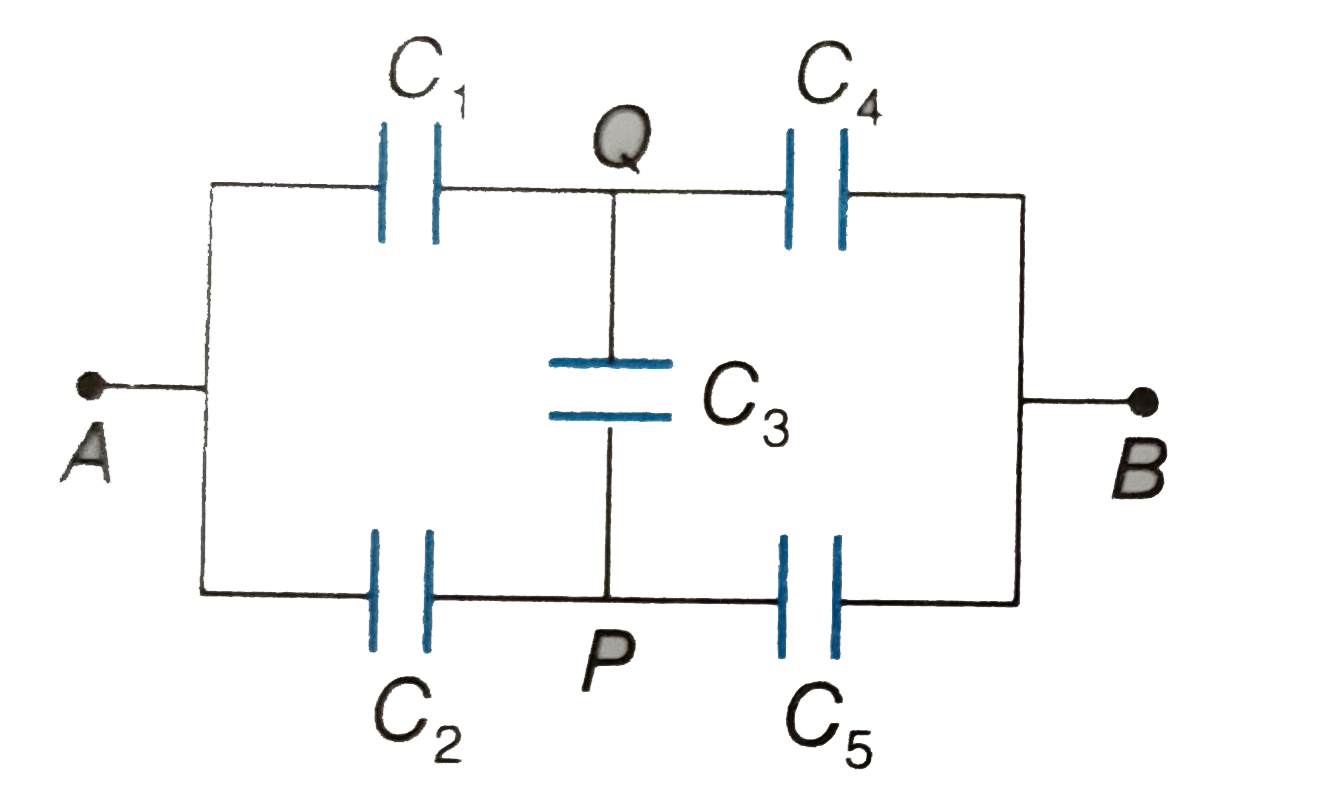
From symmetry it is seen that P and Q are equipotential points. So no current will pass through $C_{3}$ .
So, the effective capacitance between A and $B = \frac{C}{2} + \frac{C}{2} = C$ .

Updated on:21/07/2023

|

Class 12 PHYSICS CAPACITOR

Topper's Solved these Questions

CAPACITANCE AND CAPACITORBOOK - CHHAYA PUBLICATIONCHAPTER - CAPACITANCE AND CAPACITOREXERCISE - SECTION RELATED QUESTIONS
30 Videos
CAPACITANCE AND CAPACITORBOOK - CHHAYA PUBLICATIONCHAPTER - CAPACITANCE AND CAPACITOREXERCISE - HIGHER ORDER THINKING SKILL
39 Videos
ATOMIC NUCLEUSBOOK - CHHAYA PUBLICATIONCHAPTER - ATOMIC NUCLEUSEXERCISE - CBSE SCANNER
14 Videos
COMMUNICATION STSTEMBOOK - CHHAYA PUBLICATIONCHAPTER - COMMUNICATION STSTEMEXERCISE - CBSE SCANNER
28 Videos

Similar Questions

Knowledge Check

In the figure below, the capacitance of each capacitor is $3 μ F$ . The effective capacitance between points A and B is

A $\frac{3}{4} μ F$
B $3 μ F$
C $6 μ F$
D $5 μ F$
In the figure below , the capacitance of each capacitor is $3 μ F$ . The effective capacitance between A and B is

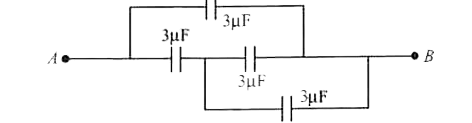A $\frac{3}{4} μ F$
B $3 μ F$
C $6 μ F$
D $5 μ F$
All capacitors used in the diagram are identical and each is of capacitance C. Then, the effective capacitance between the point A and B is :-

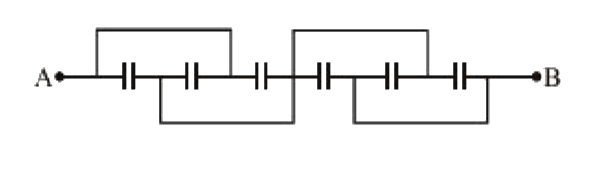A1.5 C
B6 C
C1 C
D3 C

CHHAYA PUBLICATION-CAPACITANCE AND CAPACITOR-CBSE SCANNER

Home
Ask Doubt
Profile