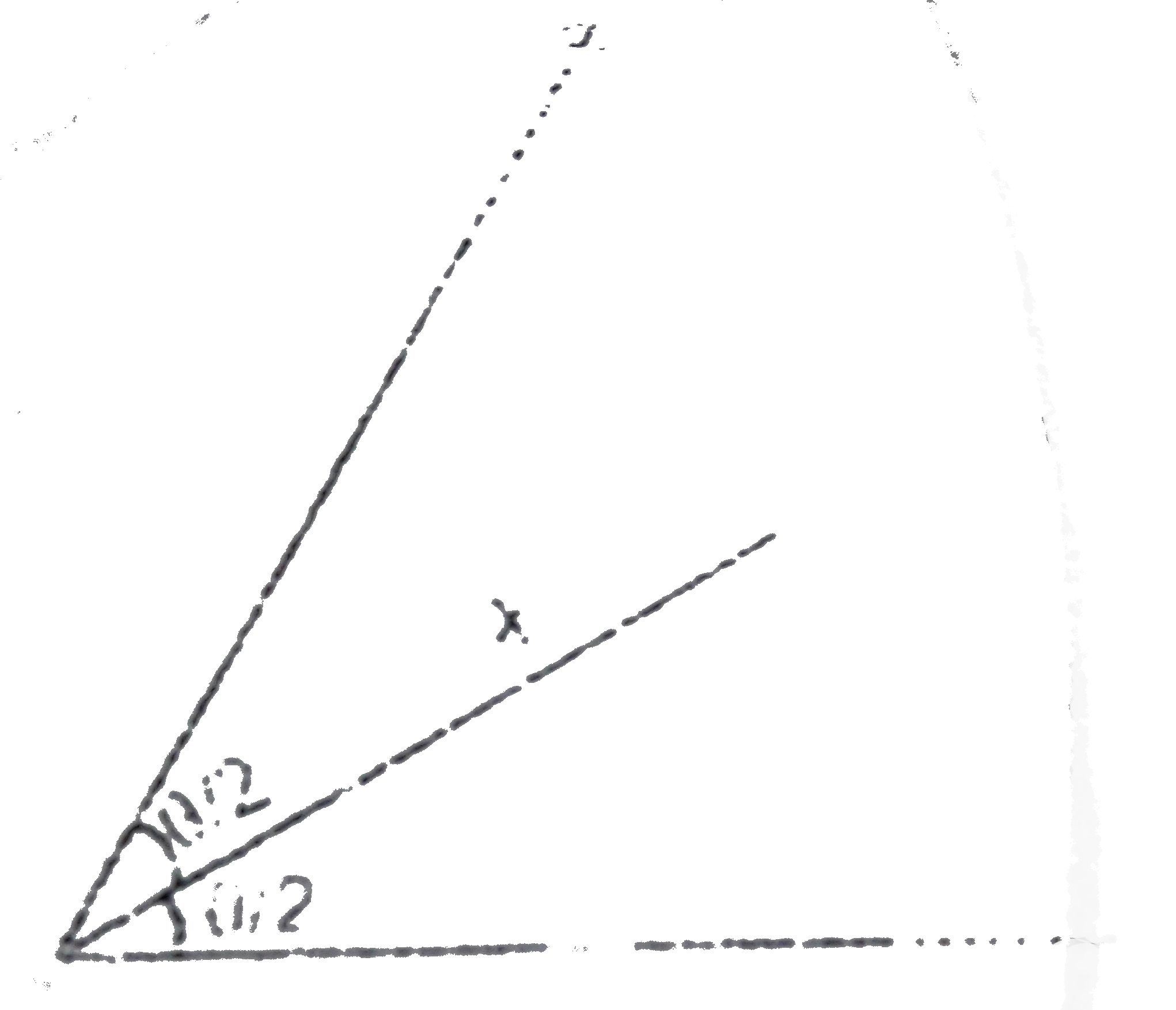
A long conducting wire carrying a current I is bent at $120^{\circ}$ [Fig. 1.128]. The magnetic field B at a point on the right bisector of bending angle at a distance d from the bend is ( $μ_{0}$ is the permeability of free space)Solution in Bengali

A

$\frac{3 μ_{0} I}{2 π d}$

B

$\frac{μ_{0} I}{2 π d}$

C

$\frac{μ_{0} I}{\sqrt{3} π d}$

D

$\frac{\sqrt{3} μ_{0} I}{2 π d}$

Video Solution

Text Solution

Verified by Experts

$P Q = d, P R = \frac{d \sqrt{3}}{2} = P S$
Magnetic field at P due to the portion QT,
$B = \frac{μ_{0}}{4 π} . \frac{I}{\frac{d \sqrt{3}}{2}} ({sin 90}^{\circ} + {sin 30}^{\circ})$
$= \frac{μ_{0}}{4 π} . \frac{2 I}{d \sqrt{3}} . \frac{3}{2} = \frac{\sqrt{3} μ_{0} I}{4 π d}$
Magnetic field at P due to the portion QU, $B_{2} = \frac{\sqrt{3} μ_{0} I}{4 π d}$

$∴$ Total magnetic field at $P = B_{1} + B_{2} = \frac{\sqrt{3} μ_{0} I}{2 π d}$

Updated on:21/07/2023

|

Class 12 PHYSICS MOVING CHARGES AND MAGNETISM

Topper's Solved these Questions

Similar Questions

CHHAYA PUBLICATION-ELECTROMAGNETISM -EXAMINATION ARCHIVE (WBJEE)

Home
Ask Doubt
Profile