A parachutist of weight ‘ w ’ strikes the ground with his legs fixed and comes to rest with an upward acceleration of magnitude 3 g . Force exerted on him by ground during landing is
w
2 w
3 w
4 w
The correct Answer is:D
To solve the problem, we need to analyze the forces acting on the parachutist when he strikes the ground and comes to rest with an upward acceleration of magnitude 3g.
1. Identify the Weight of the Parachutist:
The weight of the parachutist is given as w. This weight can be expressed in terms of mass m and gravitational acceleration g:
w=mg
2. Determine the Downward Acceleration:
The parachutist comes to rest with a downward acceleration of 3g. This means that while he is decelerating, he experiences an effective downward force due to this acceleration.
3. Calculate the Force Due to Downward Acceleration:
The force exerted by the parachutist due to this downward acceleration can be calculated using Newton's second law:
Fdown=m⋅a=m⋅3g=3mg
4. Calculate the Weight Force:
The weight force acting on the parachutist is:
Fweight=mg=w
5. Determine the Total Force Exerted on the Ground:
When the parachutist lands, the total force exerted on the ground (normal force N) is the sum of the weight force and the force due to the downward acceleration:
N=Fweight+Fdown=mg+3mg=4mg
6. Substitute the Weight into the Equation:
Since mg=w, we can substitute this into our equation for the normal force:
N=4mg=4w
7. Final Answer:
Therefore, the force exerted on him by the ground during landing is:
4w
The weight of the parachutist is given as w. This weight can be expressed in terms of mass m and gravitational acceleration g:
w=mg
The parachutist comes to rest with a downward acceleration of 3g. This means that while he is decelerating, he experiences an effective downward force due to this acceleration.
The force exerted by the parachutist due to this downward acceleration can be calculated using Newton's second law:
Fdown=m⋅a=m⋅3g=3mg
The weight force acting on the parachutist is:
Fweight=mg=w
When the parachutist lands, the total force exerted on the ground (normal force N) is the sum of the weight force and the force due to the downward acceleration:
N=Fweight+Fdown=mg+3mg=4mg
Since mg=w, we can substitute this into our equation for the normal force:
N=4mg=4w
Therefore, the force exerted on him by the ground during landing is:
4w
Topper's Solved these Questions
- NEWTONS LAWS OF MOTIONBook:ERRORLESS Chapter:NEWTONS LAWS OF MOTIONExercise:Third Law of Motion
Explore 24 Videos
- NEWTONS LAWS OF MOTIONBook:ERRORLESS Chapter:NEWTONS LAWS OF MOTIONExercise:Conservation of Linear Momentum and Impulse
Explore 27 Videos
- NEWTONS LAWS OF MOTIONBook:ERRORLESS Chapter:NEWTONS LAWS OF MOTIONExercise:Self Evaluation Test
Explore 16 Videos
- MOTION IN TWO DIMENSIONBook:ERRORLESS Chapter:MOTION IN TWO DIMENSIONExercise:Exercise
Explore 319 Videos
- ROTATIONAL MOTIONBook:ERRORLESS Chapter:ROTATIONAL MOTIONExercise:Practice Problems (Problems based on motion of connected mass)
Explore 10 Videos
Similar Questions
A rail-road car is moving towards right with acceleration a. A man accelerating toward left with an acceleration of magnitude a/3 w.r.t to car. A dog of mass m is following man A with an acceleration a/3 relative to the car. Observer B on ground is observing the dog and man A. Find the
(a) net force experienced by the dog as seen by observer B standing on ground.
(b) rate of change of linear momentum of the dog relative to the man A moving on trolley.
(c ) pseudo-free on the dog as seen from man A.

View SolutionA man of mass m is standing on a plank kept on ground having mass m. There is no friction between the plank and the ground. If the man walks with an acceleration a w.r.t. the plank when calculate the acceleration of the plank w.r.t. the ground. Also calculate frictional force exerted by the plank on man.
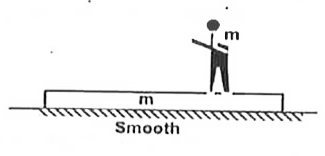
View Solution
Knowledge Check
A packet of weight w is dropped with the help of a parachute and on striking the ground comes to rest with retardation equal to twice the acceleration due to gravity. The force exerted on the ground is
AwB2wC3wD4wA student (m = 63 kg) falls freely from rest and strikes the ground. During the collision with the ground, he comes to rest in a time of 0.010 s. The average force exerted on him by the ground is +18,000 N, where the upward direction is taken to be the positive direction. From what height did the student fall ? Assume that the only force acting on him during the collision is that due to the ground.
A0.15 mB0.21 mC0.30 mD0.42 mA parachutist with total weight 75 kg drops vertically onto a sandy ground with a speed of 2ms−1 and comes to a halt over a distance of 0.25m. The average force from the ground on her is close to -
A600 NB1200 NC1350 ND1950 N
A 40 kg boy climbs a rope that passes over an ideal pulley. The other end of the rope is attached to a 60 kg weight placed on the ground. What is the maximum upward acceleration the boy can have without lifting the weight ? If he climbs the rope with upward acceleration 2 g, with what acceleration the weight will rise up ?
View SolutionA stone of weight W is thrown straight up from the ground with an intial speed u. If a drag force of constant magnitude f acts - on the same through out its flight , the speed of stone just before reaching the ground is
(1) u√W−fW (2) u√W+ff
(3)u√W+fW−f (4) u√W−fW+f
View SolutionA boy puts a heavy box of mass M of his head and jumps down from the top of a multistoried building to the ground. How much is the force exerted by the box on hishead during his free fall? Does the for greatly increase during the period he balances himself after striking teh ground?
View SolutionA parachutist after bailing out falls for 10 s without friction. When the parachute opens he descends with an acceleration of 2m/s2 against his direction and reached the ground with 4m/s from what height he has dropped himself? (g=10m/s2)
View SolutionA balloon starts rising from ground from rest with an upward acceleration 2m/s2. Just after 1 s, a stone is dropped from it. The time taken by stone to strike the ground is nearly
View Solution
