The Schrodinger wave equation for hydrogen atom of 4s- orbital is given by :
Ψ(r)=116√4(1a0)3/2[(σ2−1)(σ2−8σ+12)]e−σ/2 where a0=1st Bohr radius and σ=2ra0 . The distance from the nucleus where there will be no radial node will be :
r=a02
r=3a0
r=a0
r=2a0
The correct Answer is:D
Step by step video, text & image solution for The Schrodinger wave equation for hydrogen atom of 4s- orbital is given by : Psi (r) = (1)/(16sqrt4)((1)/(a_(0)))^(3//2)[(sigma^(2) - 1)(sigma^(2) - 8 sigma + 12)]e^(-sigma//2) where a_(0) = 1^(st) Bohr radius and sigma = (2r)/(a_(0)) . The distance from the nucleus where there will be no radial node will be : by Chemistry experts to help you in doubts & scoring excellent marks in Class 11 exams.
Topper's Solved these Questions
- BOOK - GRB PUBLICATIONCHAPTER - ATOMIC STRUCTUREEXERCISE - Reasoning Type11videos
ATOMIC STRUCTURE
- BOOK - GRB PUBLICATIONCHAPTER - ATOMIC STRUCTUREEXERCISE - Multiple Objective Type49videos
ATOMIC STRUCTURE
- BOOK - GRB PUBLICATIONCHAPTER - ATOMIC STRUCTUREEXERCISE - de Brogile and Heisenberg43videos
ATOMIC STRUCTURE
- BOOK - GRB PUBLICATIONCHAPTER - CHEMICAL EQUILIBRIUMEXERCISE - D.Le Chateliera principle16videos
CHEMICAL EQUILIBRIUM
Similar Questions
The Schrodinger wave equation for hydrogen atom is
Ψ(radial)=116√4(Za0)3/2[(σ−1)(σ2−8σ+12)]e−σ/2
where a0 and Z are the constant in which anwer can be expressed and σ=2Zra0
minimum and maximum position of radial nodes from nucleus are .... respectively.View SolutionFor a 3s - orbital, value of Φ is given by following realation:
Ψ(3s)=19√3(1a0)3/2(6−6σ+σ2)e−σ/2, where σ=2r.Z3a0
What is the maximum radial distance of node from nucleus?View Solution
Knowledge Check
- Question 1 - Select One
The Schrodinger wave equation for hydrogen atom is Ψ2s=14√2π(1a0)3/2(2−ra0)e−r/a0 , where a0 is Bohr's radius . If the radial node in 2s be at r0 , then r0 would be equal to :
Aa02B2a0C√2a0Da0√2 - Question 2 - Select One or More
Given the H−atom Rn,l=19√3(1ao)3/2(6−6σ+σ2)e−σ/2
where σ=2Zrnao,ao=0.53Å
Select the correct statement for the given orbital ?
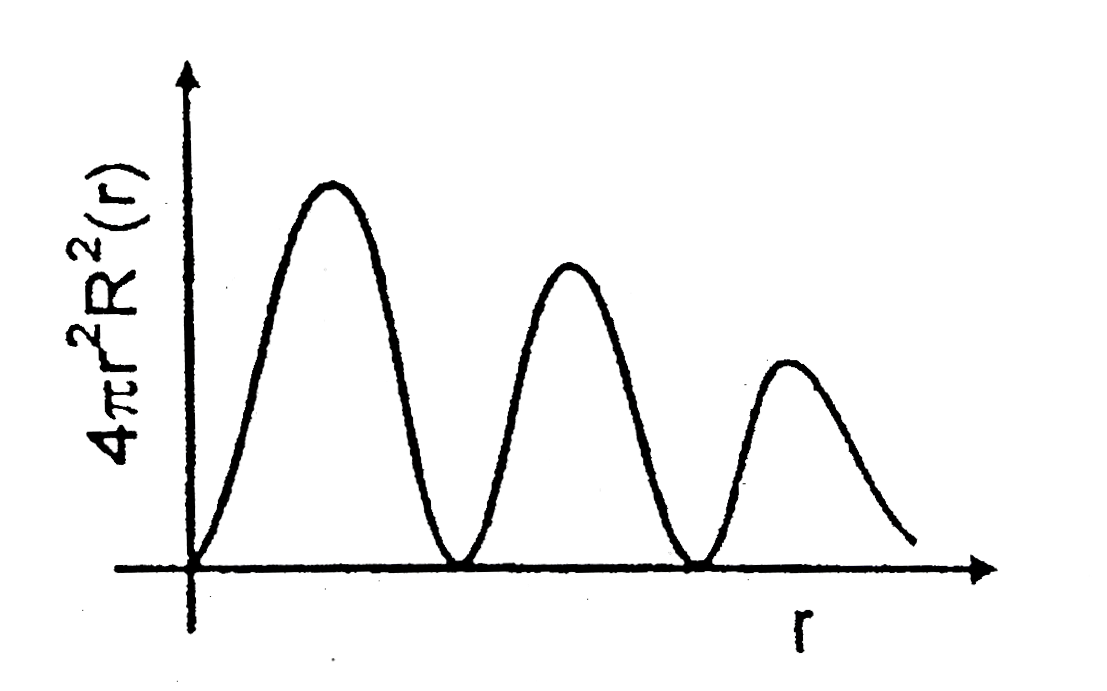AOrbital is 3sBGraph for the given orbital is :
CDistance between radial nodes is equal to 3√3aoDNone of these - Question 3 - Select One
The radial wave equyation for hydrogen of radial nodes from nucleus are:
Ψ1s=116√4(1a0)3/2[(x−1)(x2−8x+12)]e−x/2
where, x=2r/a0,a0 = radius of first Bohar orbit
The minimum and maximum position of radial nodes from nucles are:Aa0,3a0Ba02,3a0Ca02,a0Da02,4a0
[" The distance of spherical nodes "],[" from nucleus for the given orbital "],[" are "],[qquad [psi_(" radial ")=(1)/(9sqrt(2))((Z)/(a_(0)))^(3/2)[(sigma^(2)-4 sigma+3)]exp(-sigma/2],[" where "a_(0)&Z" are the constants and "],[sigma=(2Zr)/(a_(0))]]
View SolutionFor H-atom wave function for a particulaonstate is: Ψ=181√3π(1a0)3/2(σ2−10σ+25)e
Where σ=r/a0 and a0 is Bohr's radius (0.53∘A). Then distance of farthest radius mode is approximately.View SolutionFor a 3s-orbital
Φ(3s)=1a√3(1a0)3/2(6−6σ+σ2)∈−σ/2
where σ=2rZ3aσ
What is the maximum radial distance of node from nucleus?View SolutionThe radial wave equation for hydrogen of radial nodes from nucleus are:
Ψ1s=116√4(1a0)3/2[(x−1)(x2−8x+12)]e−x/2
where, x=2r/a0,a0 = radius of first Bohr orbit
The minimum and maximum position of radial nodes from nucleus are:View SolutionFor an orbital in B+4 radial function is :
R(r)=19√6(za0)34(4−σ)σe−σ/2
where σ=Zra0 and a0=0.529Å,Z = atomic number, r= radial distance from nucleus.
The radial node of orbital is at distance from nucleous.View Solution