Read the following passage carefully and answer the questions
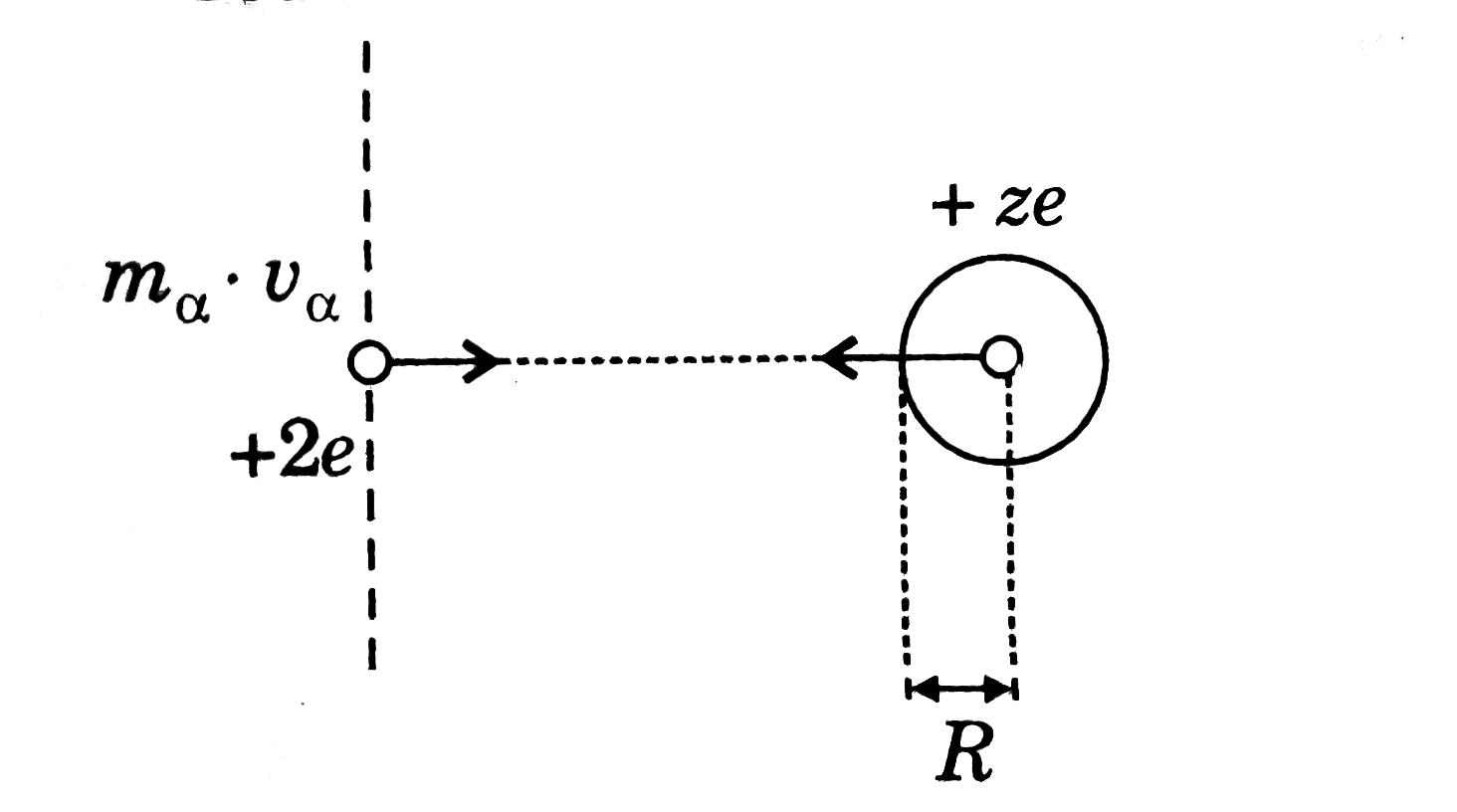
The approximate size of the nucleus can be calculated by using energy conservation theorem in Rutherford's α−scattering experiment. If an α−perticle is projected from infinity with speed v, towards the nucleus having z protons then the apha−perticle which is reflected back or which is deflected by 180∘ must have approach closest to the nucleus. It can be approximated that α−particles collides with the nucleus and gets back. Now if we apply the energy conservation at initial and collision point then:
(Total Energy)initial=(Total Enregy)final
(KE)i+(PE)i=(KE)f+(PE)f
(PE)i=0,sincePE of two charge system separated by infinite distance is zero, finally the particle stops and then starts coming back.
12mαv2α+0=0+kq1q2R
⇒12mαv2α=k2e×zeR⇒R=4kze2mαv2α
Thus the radius of nucleus can be calculated using above equation. The nucleus is so small a particle that we can't define a sharp boundary for it . Experiments show that the average radius R fo a nucleus may be written as
R=R0(A)1/3
where R0=1.2×10−15m
A= atomic mass number
R=radius of nucleus
If the diameter of two different nuclei are in the ratio 1:2 then their mass number are in the ratio:
0.043055555555556
0.33402777777778
0.047222222222222
0.044444444444444
The correct Answer is:c
Step by step video, text & image solution for Read the following passage carefully and answer the questions The approximate size of the nucleus can be calculated by using energy conservation theorem in Rutherford's alpha-scattering experiment. If an alpha-perticle is projected from infinity with speed v, towards the nucleus having z protons then the apha-perticle which is reflected back or which is deflected by 180^(@) must have approach closest to the nucleus. It can be approximated that alpha-particles collides with the nucleus and gets back. Now if we apply the energy conservation at initial and collision point then: ("Total Energy")_("initial") = ("Total Enregy")_("final") (KE)_(i) +(PE)_(i)=(KE)_(f)+(PE)_(f) (PE)_(i) =0, "since"PE of two charge system separated by infinite distance is zero, finally the particle stops and then starts coming back. 1/2m_(alpha)v_(alpha)^(2) + 0=0 + (kq_(1)q_(2))/(R) rArr1/2m_(alpha)v_(alpha)^(2)=k(2exxze)/(R)rArrR=(4kze^(2))/(m_(alpha)v_(alpha)^(2) Thus the radius of nucleus can be calculated using above equation. The nucleus is so small a particle that we can't define a sharp boundary for it . Experiments show that the average radius R fo a nucleus may be written as R=R_(0)(A)^(1//3) where R_(0) = 1.2xx10^(-15)m A= atomic mass number R=radius of nucleus If the diameter of two different nuclei are in the ratio 1:2 then their mass number are in the ratio: by Chemistry experts to help you in doubts & scoring excellent marks in Class 11 exams.
Updated on:21/07/2023Topper's Solved these Questions
- ATOMIC STRUCTUREBOOK - GRB PUBLICATIONCHAPTER - ATOMIC STRUCTUREEXERCISE - Match the column type
16 Videos
- ATOMIC STRUCTUREBOOK - GRB PUBLICATIONCHAPTER - ATOMIC STRUCTUREEXERCISE - Subjective Type
55 Videos
- ATOMIC STRUCTUREBOOK - GRB PUBLICATIONCHAPTER - ATOMIC STRUCTUREEXERCISE - Multiple Objective Type
49 Videos
- CHEMICAL EQUILIBRIUMBOOK - GRB PUBLICATIONCHAPTER - CHEMICAL EQUILIBRIUMEXERCISE - D.Le Chateliera principle
16 Videos
Similar Questions
Rutherford model: The approximate size of the nucleus can be calculated by using energy conservation theorem in Rutherford's α-scattering experiment. If an α-particle is projected from infinity with speed v towards the nucleus having Z protons, then the α-particle which is reflected back or which is deflected by 180∘ must have approached closest to the nucleus .It can be approximated that α particle collides with the nucleus and gets back. Now if we apply the energy conservation equation at initial point and collision point then:
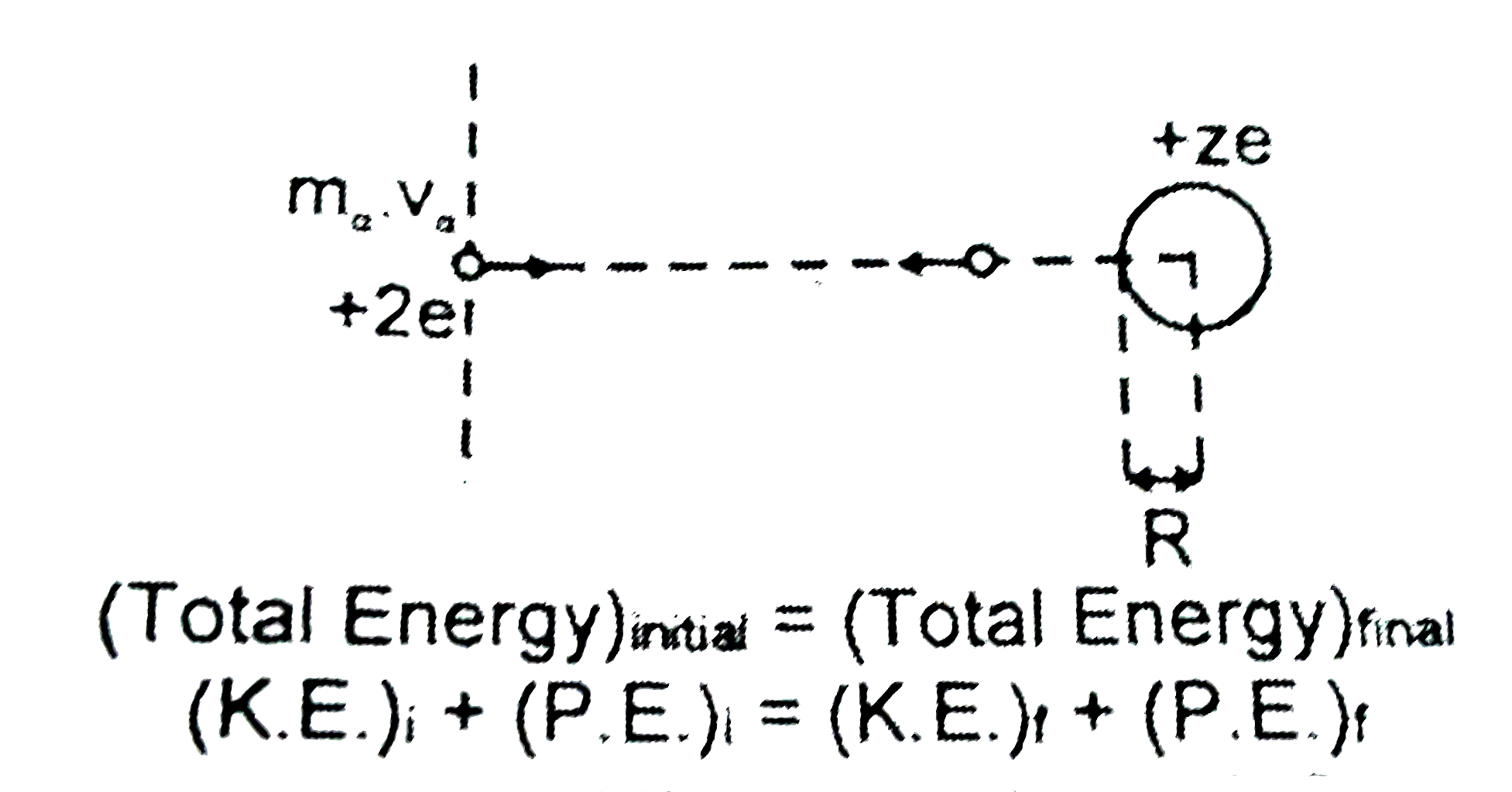
(P.E.)i=0, since P.E. of two charge system separated by infinite distance is zero. Finally the particle stops and then starts coming back.
12mαv2α+0=0+Kq1q2R⇒12mαv2α=K2e×ZeR⇒R=4KZe2mαv2α
Thus the radius of nucleus can be calculated using above equation. The nucleus is so small a particle that we can't define a sharp boundary for it
An α-particle with initial speed v0 is projected from infinity and it approaches up to r0 distance from a nuclie. Then, the initial speed of α-particle, which approaches upto 2r0 distance from the nucleus is :
View SolutionWhich fact is proved by the following observation in Rutherford's scattering experiment' very few alpha particles are deflected back'?
View Solution
Knowledge Check
Read the following passage carefully and answer the questions
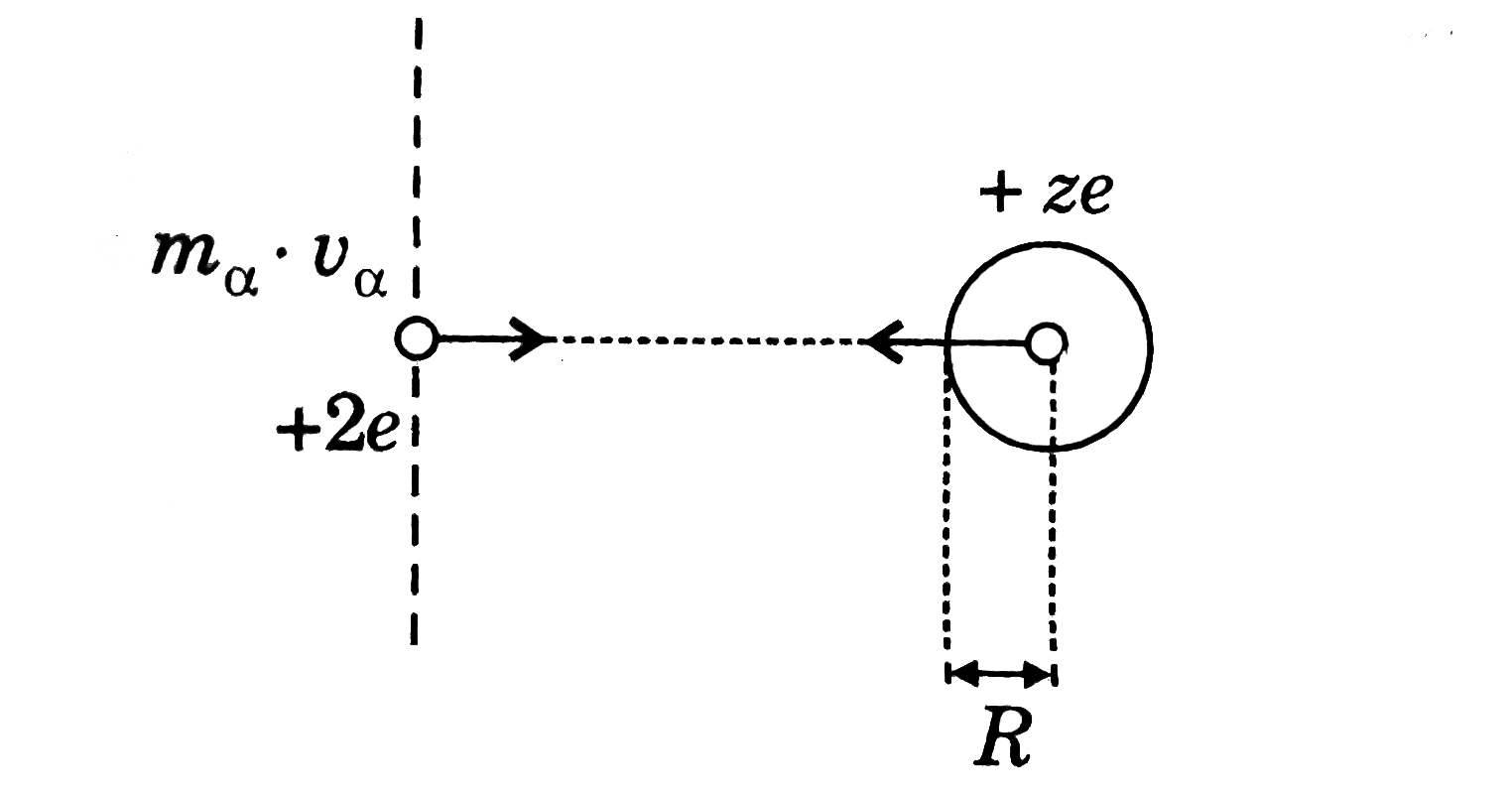
The approximate size of the nucleus can be calculated by using energy conservation theorem in Rutherford's α−scattering experiment. If an α−perticle is projected from infinity with speed v, towards the nucleus having z protons then the apha−perticle which is reflected back or which is deflected by 180∘ must have approach closest to the nucleus. It can be approximated that α−particles collides with the nucleus and gets back. Now if we apply the energy conservation at initial and collision point then:
(Total Energy)initial=(Total Enregy)final
(KE)i+(PE)i=(KE)f+(PE)f
(PE)i=0,sincePE of two charge system separated by infinite distance is zero, finally the particle stops and then starts coming back.
12mαv2α+0=0+kq1q2R
⇒12mαv2α=k2e×zeR⇒R=4kze2mαv2α
Thus the radius of nucleus can be calculated using above equation. The nucleus is so small a particle that we can't define a sharp boundary for it . Experiments show that the average radius R fo a nucleus may be written as
R=R0(A)1/3
where R0=1.2×10−15m
A= atomic mass number
R=radius of nucleus
If α−particle with speed v0 is projected from infinity and it approaches upto r0 distance from the nuclei. Then the speed of α−particle which approaches 2r0 distance from the nucleus isA√2v0Bv0√2C2v0Dv02Read the following passage carefully and answer the questions
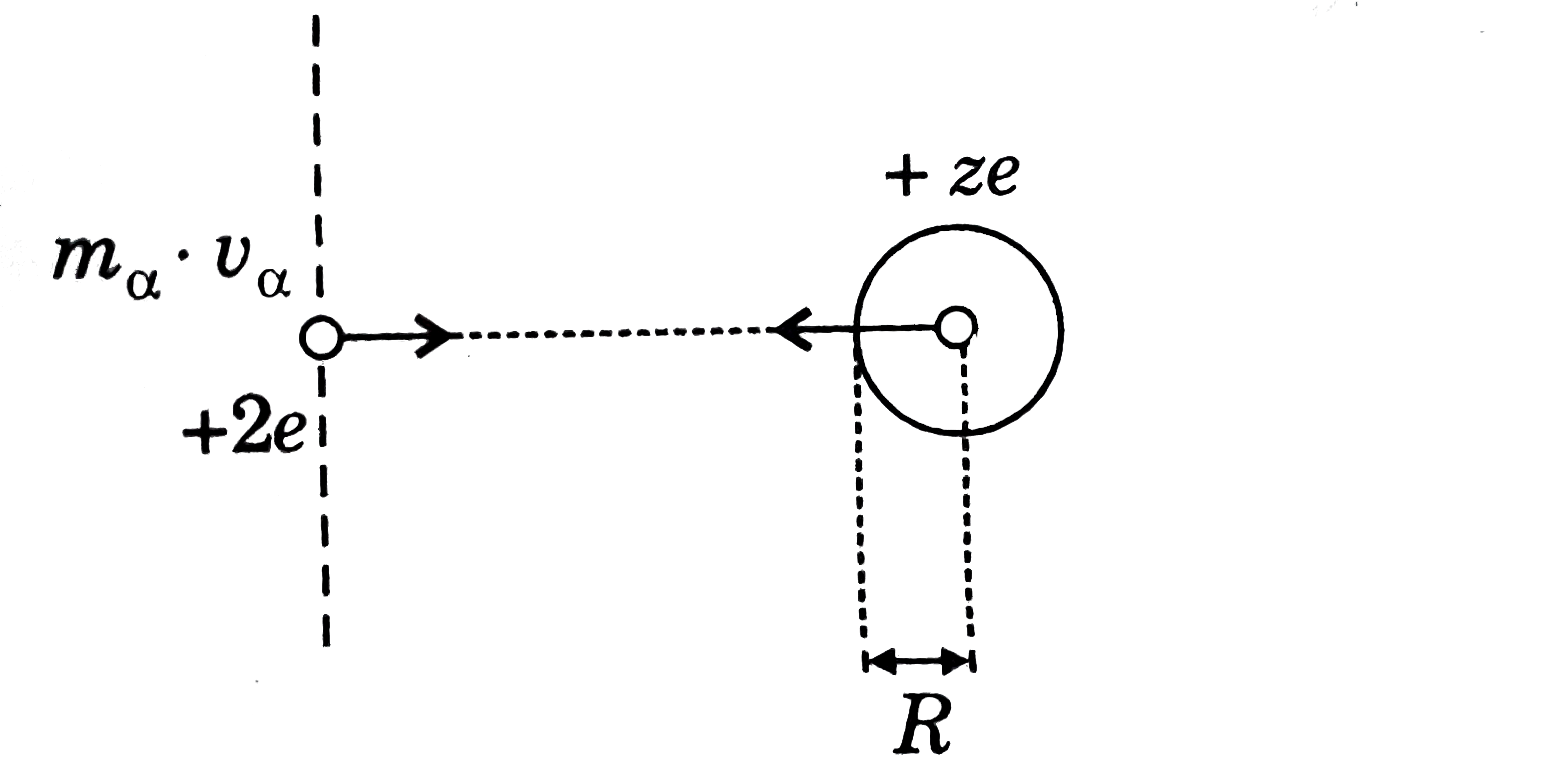
The approximate size of the nucleus can be calculated by using energy conservation theorem in Rutherford's α−scattering experiment. If an α−perticle is projected from infinity with speed v, towards the nucleus having z protons then the apha−perticle which is reflected back or which is deflected by 180∘ must have approach closest to the nucleus. It can be approximated that α−particles collides with the nucleus and gets back. Now if we apply the energy conservation at initial and collision point then:
(Total Energy)initial=(Total Enregy)final
(KE)i+(PE)i=(KE)f+(PE)f
(PE)i=0,sincePE of two charge system separated by infinite distance is zero, finally the particle stops and then starts coming back.
12mαv2α+0=0+kq1q2R
⇒12mαv2α=k2e×zeR⇒R=4kze2mαv2α
Thus the radius of nucleus can be calculated using above equation. The nucleus is so small a particle that we can't define a sharp boundary for it . Experiments show that the average radius R fo a nucleus may be written as
R=R0(A)1/3
where R0=1.2×10−15m
A= atomic mass number
R=radius of nucleus
Radius of a particular nucleus is calculated by the projection of α−particle from infinity at a particular speed. Let this radius be the true radius. If the radius calculation for the same nucleus is made by , α−particle with half of the earlier speed then the percentage error involved in the radius calculation is :A0.75B1C3D4Rutherford's α- scattering experiment related to the size of the-
AnucleusBatomCelectronDneutron
Write two conclusions which have been derived from Rutherford's α-ray scattering experiment .ll
View SolutionA 12.5 MeV α- particle approaching a gold nucleus is deflected 180∘ . What is the closest distance to which it approaches the nucleus ?
View SolutionIn Rutherford's scattering experiment of α-particles by metallic foils, with the increase of atomic number of nucleus, the scattering angle .
View SolutionRutherford's scattering experiment is related to the________of the nucleus.
View SolutionWhich of these is true regarding an α particle with mass 'm' projected towards the nucleus with velocity 'v ?
View Solution
