CH4 gas is behaving non-ideally. Compressibility factor for gas is 1.5 at 2 atm, 400K. Calculate molar volume for gas: [Given: R=0.08Litre−atmK−mole]
24 litre
16 litre
48 litre
8 litre
The correct Answer is:a
Topper's Solved these Questions
Similar Questions
Density of ideal gas at 2.46 atm and 300 K is 0.8g/L Hence g-molar mass of gas is :
[R=0.082L-atm/mol-K]
View SolutionOne mole of an ideal gas at 27∘ ,8.21 atm absorbs 420 cal of heat during a reversible isothermal expansion. Calculate the final volume (in litre) of gas. [Use:R=0.0821 litre-atm/K-mole]
View Solution
Knowledge Check
80 gm of SOx gas occupies 15 litre at 2atm and 300K. The value of x is : (Given: R= 0.08 L-atm/K-mole)
A3B2C1DnoneZ us P graph is plotted for 1 mole of hypothetical gas. Volume of gas at this point A is: [R=0.08atmL/mole−K]
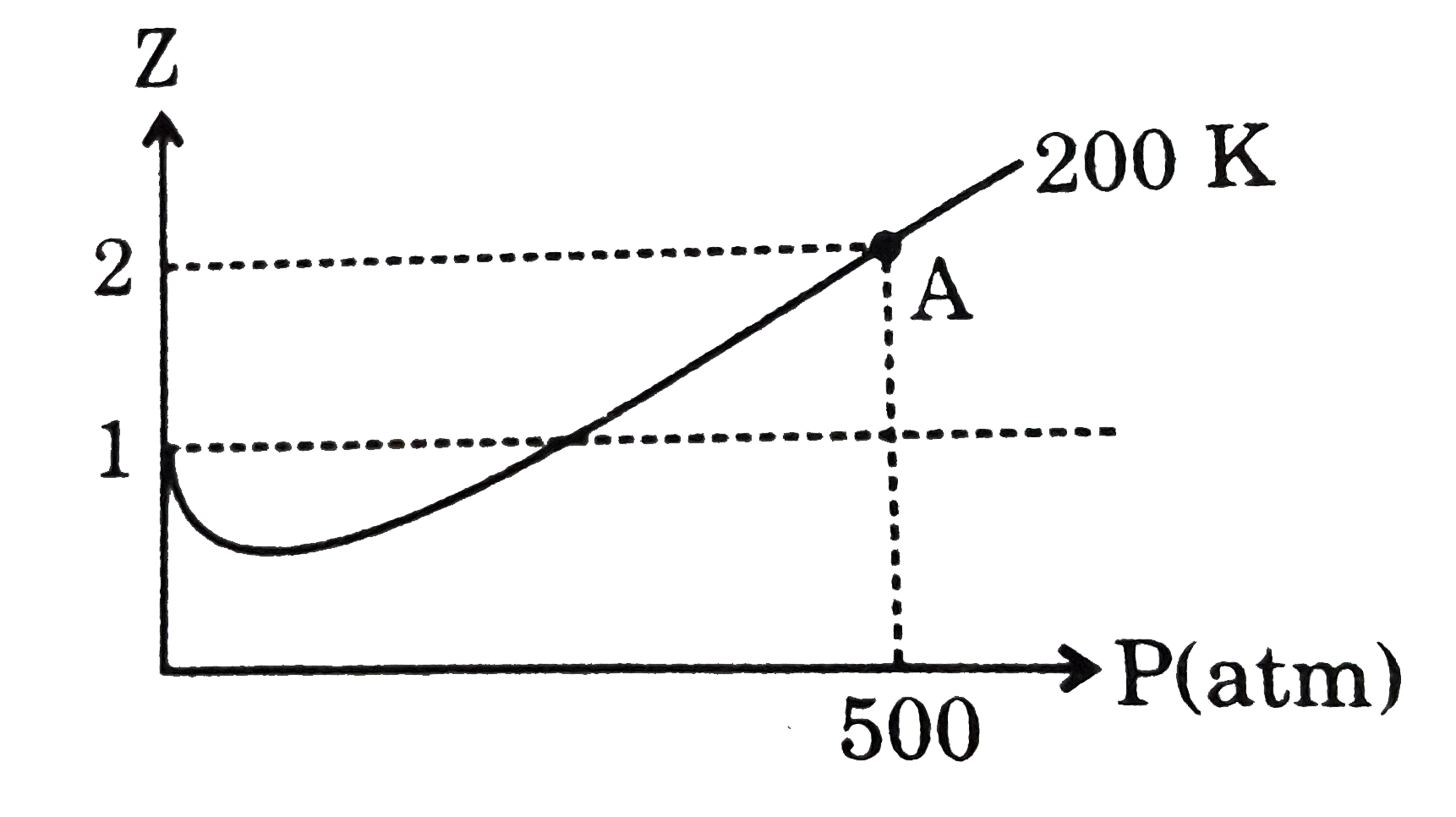 A0.01LB0.09LC0.064LD0.64L
A0.01LB0.09LC0.064LD0.64L5 moles gas are introduced in 1 litre container at 47∘C. Select the correct option(s).
[R=0.08litre-atm/mol-K]APressure would be 128 atm if it behaves ideallyBpressure would be 28 atm if it follows van der Waals' equation, a=4 atm-litre2/mol2 and b=0CPressure would be 33.33 atm if it follows van der Waals' equation , a=4 atm-litre2/mol2 and b=0.04L/moleDPressure would be 160 atm if it follows van der Waal's equation, a=0atm−litre2/mol2 and b=0.04L/mole
The compressibility factor for a given real gas is 0.927 at 273K and 100atm Calculate the amount of gas required to fill a gas cylinder of 100 litre capacity under given conditions
(Mol weight of gas =30) .
View SolutionAt 200 K and 500 atm value of compressibility factor is 2.Then volume of the gas at the point will be
View Solution2.8 g of a gas at 1atm and 273K occupies a volume of 2.24 litres. The gas can not be:
View SolutionIf two moles of an ideal gas at 500 K occupies a volume of 41 litres, the pressure of the gas is (R=0.082LatmK−1mol−1)
View SolutionConsider the given graph: Graph is plotted for 1 mol of gas at 400K, find slope of curve [take: R=0.08L−atmmol−K]

View Solution
