A uniform cube of mass M is floating on the surface of a liquid with three fourth of its volume immersed in the liquid (density of liquid is ρ). Now a cubical cavity is made inside the cube and the cavity is filled by a material having twice the density of the original cube. The minimum dimension (sides) of this cavity such that this cube is now completely immersed in the liquid is [4Mnρ]13. Find the value of n
The correct Answer is:9
Side of cube =a
Mg=34a3ρg
If side of cavity is b.
New mass of cube
=(a3−b3)34ρ+b364ρ=(a3+b2)34ρ
a3ρg=(a3+b3)34ρg
b3=a33,b=[13]13a
b=[49Mρ]13
Similar Questions
A cubical block is floating in a liquid with half of its volume immersed in the liquid.When the whole system accelerates upward with a net acceleration of g/2 the fraction of volume immersed in the liquid will become
View SolutionA cubical block is floating in a liquid with half of its volume immersed in the liquid.When the whole system accelerates upward with a net acceleration of g/2 the fraction of volume immersed in the liquid will become
View Solution
Knowledge Check
- Question 1 - Select One
A uniform cube of mass M is floating on the surface of a liquid with three fourth of its volume immersed in the liquid (density=ρ). The length of the side of the cube is equal to
 A(4M/3ρ)2/3B(M/3ρ)2/3C(M/4ρ)2/3DNone of these
A(4M/3ρ)2/3B(M/3ρ)2/3C(M/4ρ)2/3DNone of these - Question 2 - Select One
A block of density ρ floats in a liquid with its one third volume immersed. The density of the liquid is
AρBρ3Cρ2D3ρ - Question 3 - Select One
A cube of side 20 cm is floating on a liquid with 5 cm of the cube outside the liquid. If the density of liquid is 0.8gm/cc then the mass of the cube is
A4.2 kgB4.8 kgC5 kgD5.2 kg
A cubical block is floating in a liquid with one fourth o its volume immersed in the liquid. If whole of the system accelerates upward with acceleration g/4, the fraction of volume immersed in the liquid will be :-
View SolutionA body floats in water with its one-third volume above the surface. The same body floats in a liquid with one-third volume immersed. The density of the liquid is
View Solution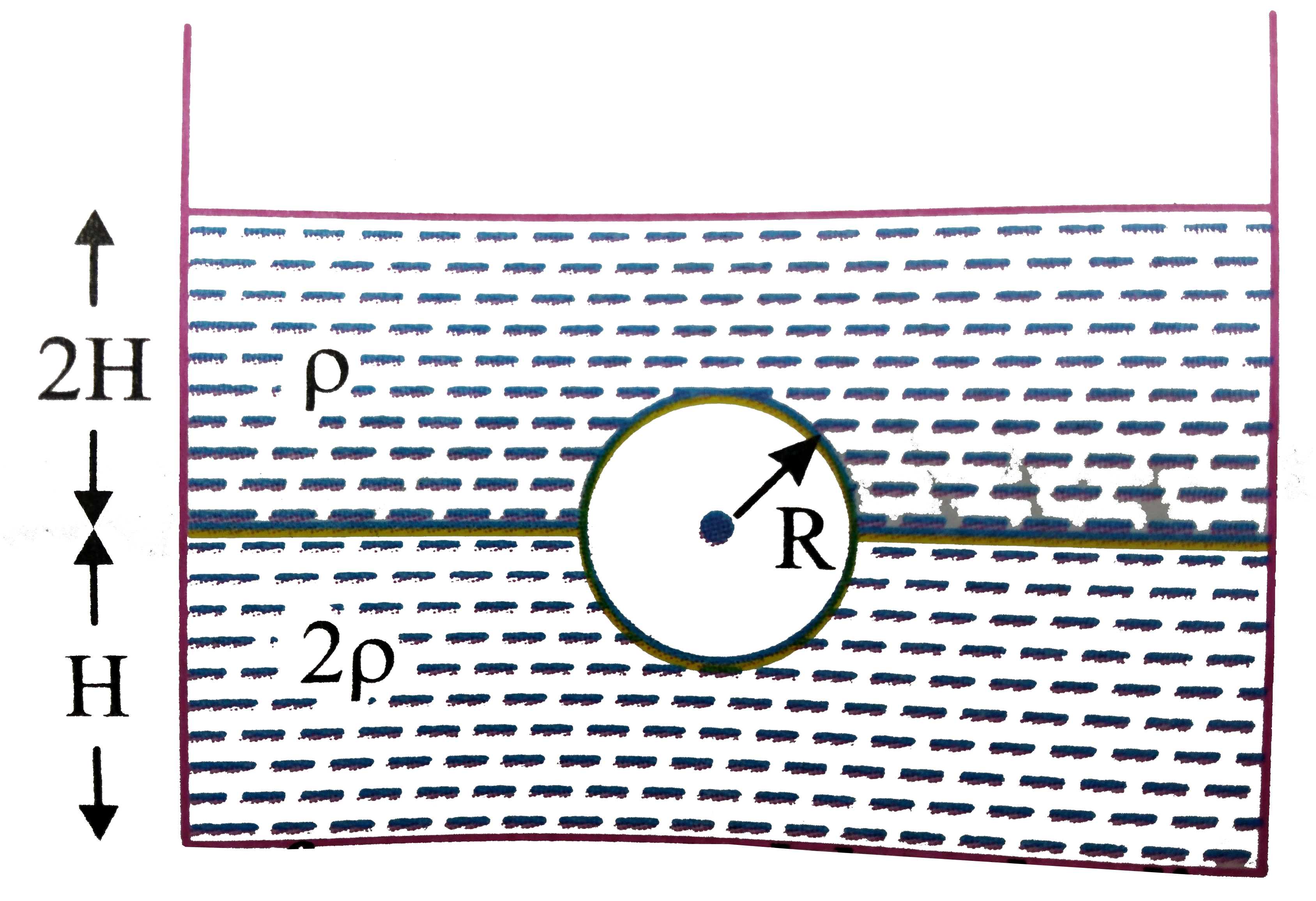
A spherical ball of radius R is floating at the interface of two liquids with densities ρ and 2ρ. The volumes of the ball immersed in two liquids are equal. Answer the following questions:
Find the force exerted by the liquid with density 2ρ on the ballView SolutionA cubical block is floating in a liquid with half of its volume immersed in the liquid. When the whole system accelerates upwards with a net acceleration of g/4, the fraction of volume immersed in the liquid will be
View SolutionA cubical block is floating in a liquid with half of its volume immersed in the liquid. When the whole system accelerates upwards with acceleration of g/3, the fraction of volume immersed in the liquid will be
 View Solution
View Solution

