In case of a simple pendulum, time period versus length is depicted by
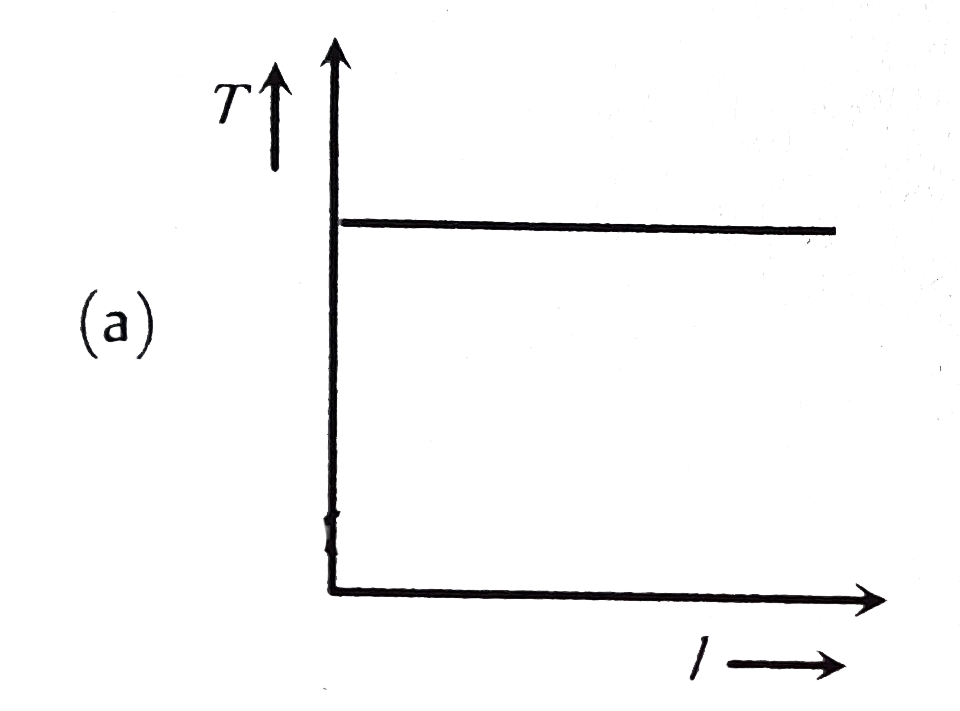
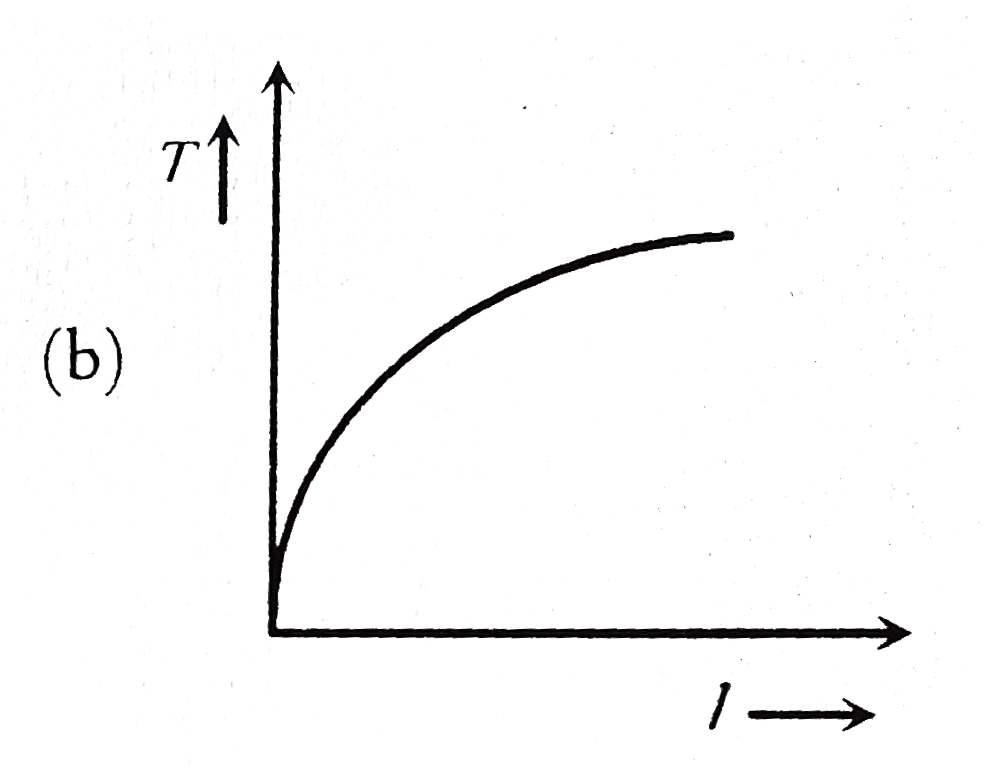
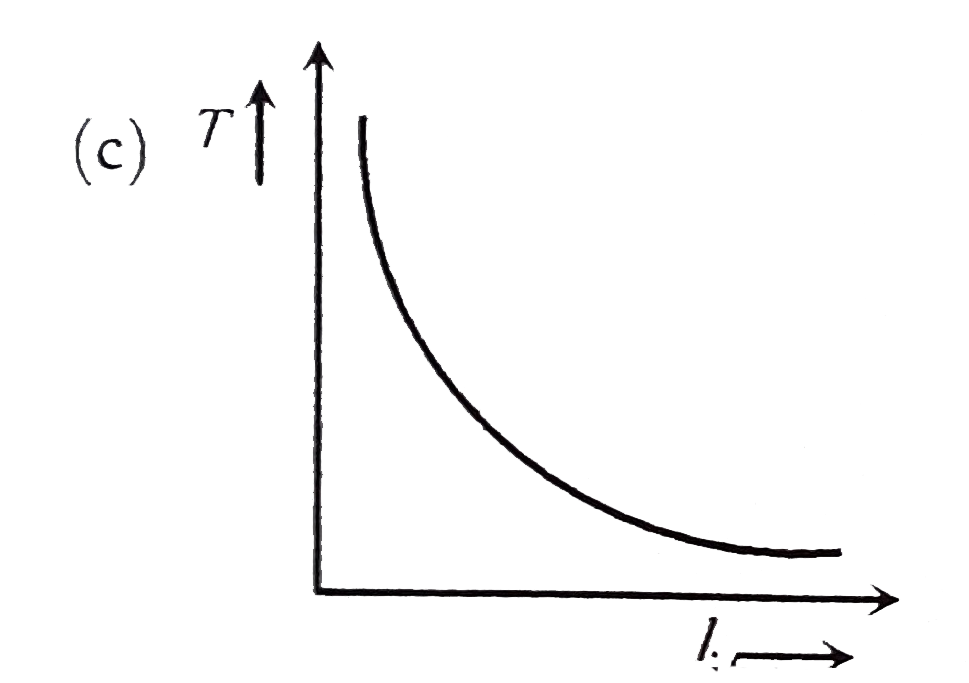
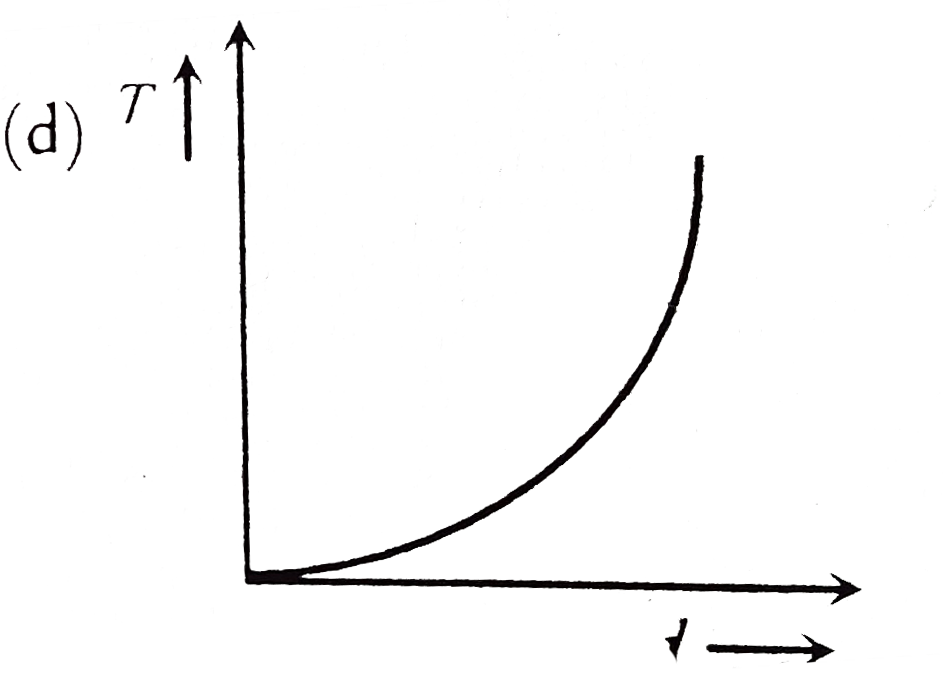
The correct Answer is:B
Step by step video, text & image solution for In case of a simple pendulum, time period versus length is depicted by by Physics experts to help you in doubts & scoring excellent marks in Class 11 exams.
Updated on:21/07/2023Topper's Solved these Questions
- SIMPLE HARMONIC MOTIONBOOK - ERRORLESS CHAPTER - SIMPLE HARMONIC MOTIONEXERCISE - Assertion & Reason
23 Videos
- SIMPLE HARMONIC MOTIONBOOK - ERRORLESS CHAPTER - SIMPLE HARMONIC MOTIONEXERCISE - simple Harmonic Motion
21 Videos
- SIMPLE HARMONIC MOTIONBOOK - ERRORLESS CHAPTER - SIMPLE HARMONIC MOTIONEXERCISE - Superposition of S.H. M and Resonance
14 Videos
- ROTATIONAL MOTIONBOOK - ERRORLESS CHAPTER - ROTATIONAL MOTIONEXERCISE - Practice Problems (Problems based on motion of connected mass)
10 Videos
- SURFACE TENSIONBOOK - ERRORLESS CHAPTER - SURFACE TENSIONEXERCISE - Exercise
214 Videos
Similar Questions
Knowledge Check
Time period of simple pendulum is
AT=2π√lgBT=2π√glCT=√2πlgDT=12π√glAn amplitue of a simple pendulum of a period 'T' and length 'L' is increased by 5%. The new period of that pendulum will be
AT8BT4CT2DTThe period of a simple pendulum is double, when its length is increased by 1.2 m. The original length of the simple pendulum is
A0.2 mB0.3 mC0.4 mD0.5 m
The time period of a simple pendulum is 2s. It its length is increased by 4 times, then its period becomes
View SolutionThe time period of a simple pendulum of infinte length is
View SolutionIn the case of a simple pendulum, a graph is drawn between the displacement and time taken for its oscillations as shown in the figure. Then
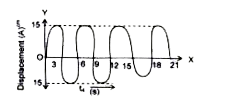
Time period of the pendulum bob is ______ s.
View SolutionThe time period of oscillation of a simple pendulum is √2s. If its length is decreased to half of initial length, then its new period is
View SolutionThe time period of simple pendulum is T. If its length is increased by 2%, the new time period becomes
View Solution
