Figure shows a conductor of length l having a circular cross section. The radius of cross section of the conductor varies linearly from r1 to r2 along its length. IF the specific resistance of the material of the conductor be ρ, find the resistance of the conductor.
Let the axis of the conductor be along the x axis of the coordinate system.
At x=0, radius of cross section is r1 and at x=1 radius of cross section is r2. ltbr Radius of cross section varies along the length of the conductor,So it can be written that
drdx=k ( where k is constant)
Now intergrating the above equation , we get , r=kx+c
where c is integration constant
when x=0, r=r1
∴r1=k.0+cor,c=r1
So, r=kx+r1 ...(1)
Again when x=l, then r=r2
∴r2=kl+r1
or, k=r2−r1l .....(2)
From equation (1) and (2) , we get
r=r2−r1lx+r1
At a distance x from the left side of the conductor, resistance of a circular disc of thickness dx is
dR=ρdxπr2=ρdxπ[(r2−r1l)x+r1]2
∴ Total resistance of the conductor,
R=ρπ∫10dx[(r2−r1l)x+r1]2
Let (r2−r1l)x+r=uor,dx=(lr2−r1)du
when x=0 then u=r1 and when x=1 then u=r2.
∴R=ρlπ∫r2r1du(r2−r1)u2=ρlπ(r2−r1)[−1u]r2r1
=ρlπ(r2−r1)[1r1−1r2]=ρlπr1r2
This is the required resistance.
Topper's Solved these Questions
- CURRENT ELECTRICITY BOOK - CHHAYA PUBLICATIONCHAPTER - CURRENT ELECTRICITY EXERCISE - NCERT TEXTBOOK QUESTION WITH ANSWER HINT
4 Videos
- CURRENT ELECTRICITY BOOK - CHHAYA PUBLICATIONCHAPTER - CURRENT ELECTRICITY EXERCISE - NCERT EXEMPLAR QUESTION WITH ANSWER HINT(MCQ-1)
2 Videos
- CURRENT ELECTRICITY BOOK - CHHAYA PUBLICATIONCHAPTER - CURRENT ELECTRICITY EXERCISE - CBSE SCANNER
16 Videos
- COMMUNICATION STSTEMBOOK - CHHAYA PUBLICATIONCHAPTER - COMMUNICATION STSTEMEXERCISE - CBSE SCANNER
28 Videos
- DIFFRACTION AND POLARISATION OF LIGHTBOOK - CHHAYA PUBLICATIONCHAPTER - DIFFRACTION AND POLARISATION OF LIGHTEXERCISE - CBSE SCANNER
27 Videos
Similar Questions
Figure shows a conductor of length l having a circular cross-section. The radius of cross-section varies linearly from a to b. Find the resistance of the conductor. ltbtgt
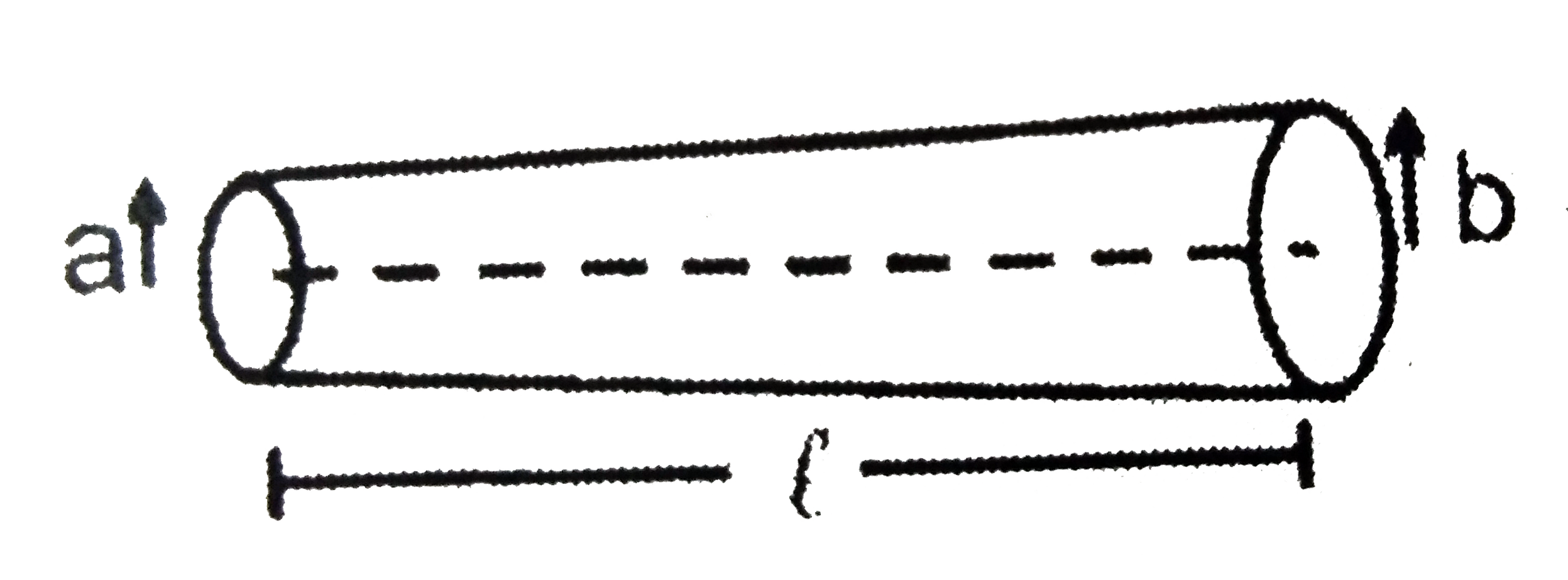
View SolutionShown a conductor of length l having a circular cross section. The radius of cross section varies linearly form a→b. The resistivity of the material is(ρ).Assuming that b−a<<l,find the resistance of the conductor.

View Solution
Knowledge Check
If the radius of cross-section of the conductor is increased by 0.1% keeping volume constant, then percentage change in the resistance of the conductor is
A−0.2%B−0.1%C−0.3%D−0.4%A conductor of length l and area of cross-section A has a resistance R then its specific resistance is
Aρ=RAlBρ=RAlCρ=lRADρ=R2AlHow is electrical conductance of a conductor related with length and area of cross section of the conductor ?
AG=l.a.κ−1BG=κ.l.a−1CG=κ.a.l−1DG=κ.l.a−2
Shown a conductor of length l having a circular cross section. The radius of cross section varies linearly form a→b. The resistivity of the material is(ρ).Assuming that b−a<<l,find the resistance of the conductor.

View SolutionA conductor of length l has a non-uniform cross-section. The radius of cross-section varies linearly from a to b. The resistivity of the material is ρ. Find the resistance of the conductc across its ends.

View SolutionA conductor of length l has a non-uniform cross-section. The radius of cross-section varies linearly from a to b. The resistivity of the material is ρ. Find the resistance of the conductc across its ends.

View SolutionIf the radius of cross-section of the conductor is increased by 0.1% keeping volume constant, then percentage change in the resistance of the conductor is
View SolutionHow is electrical conductance of a conductor related with length and area of cross section of the conductor ?
View Solution
